Clusters should not be sorted from Major to Supporting and then taught in that order. To do so would strip the coherence of the mathematical ideas and miss the opportunity to enhance the major work of the grade with the supporting clusters.
- Assessment Limits :
Items may require the student to use knowledge of other Geometry
standards.Items that use volume should not also assess G-GMD.1.3 or GMG.1.1.
- Calculator :
Neutral
- Clarification :
Students will apply geometric methods to solve design problems. - Stimulus Attributes :
Items must be set in a real-world context. - Response Attributes :
Items may require the student to interpret the results of a solution
within the context of the modeling situation.Items may require the student to apply the basic modeling cycle.
Items may require the student to use or choose the correct unit of
measure
- Test Item #: Sample Item 1
- Question:
The trunk of a palm tree has cylindrical tubes that carry water. Each tube is 0.0003 meters wide. One of the tubes in a palm tree trunk is shown.
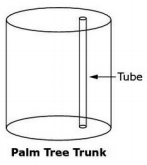
A. Using the diagram as a model, approximately how many tubes could fit in a palm tree trunk with a diameter of 0.5 meters?
B. The tubes in a palm tree are between 20 to 21 meters long. What is the approximate volume, in cubic meters, of one tube?
- Difficulty: N/A
- Type: EE: Equation Editor
Related Courses
Related Access Points
Related Resources
Formative Assessments
Lesson Plans
Perspectives Video: Expert
Perspectives Video: Professional/Enthusiasts
Problem-Solving Tasks
Video/Audio/Animation
STEM Lessons - Model Eliciting Activity
Students use measures and properties of rectangular prisms and cylinders to model and rank 3D printable designs of interchangeable wristwatch bands that satisfy physical constraints.
Model Eliciting Activities, MEAs, are open-ended, interdisciplinary problem-solving activities that are meant to reveal students’ thinking about the concepts embedded in realistic situations. Click here to learn more about MEAs and how they can transform your classroom.
Students apply geometric measures and methods, art knowledge, contextual information, and utilize clear and coherent writing to analyze NASA space shuttle mission patches from both a mathematical design and visual arts perspective.
Model Eliciting Activities, MEAs, are open-ended, interdisciplinary problem-solving activities that are meant to reveal students’ thinking about the concepts embedded in realistic situations. Click here to learn more about MEAs and how they can transform your classroom.
This MEA requires students to design a custom snowboard for five Olympic athletes, taking into consideration how their height and weight affect the design elements of a snowboard. There are several factors that go into the design of a snowboard, and the students must use reasoning skills to determine which factors are more important and why, as well as what factors to eliminate or add based on the athlete's style and preferences. After the students have designed a board for each athlete, they will report their procedure and reasons for their decisions.
"Poly Wants a Bridge" is a model-eliciting activity that allows students to assist the city of Polygon City with selecting the most appropriate bridge to build. Teams of students are required to analyze properties of bridges, such as physical composition and span length in order to solve the problem.
Model Eliciting Activities, MEAs, are open-ended, interdisciplinary problem-solving activities that are meant to reveal students’ thinking about the concepts embedded in realistic situations. Click here to learn more about MEAs and how they can transform your classroom.
The Turning Tires MEA provides students with an engineering problem in which they must work as a team to design a procedure to select the best tire material for certain situations. The main focus of the MEA is applying surface area concepts and algebra through modeling.
Model Eliciting Activities, MEAs, are open-ended, interdisciplinary problem-solving activities that are meant to reveal students’ thinking about the concepts embedded in realistic situations. Click here to learn more about MEAs and how they can transform your classroom.
MFAS Formative Assessments
Students are asked to solve a design problem in which a triangular tract of land is to be partitioned into two regions of equal area.
Students are asked to solve a design problem in which a softball complex is to be located on a given tract of land subject to a set of specifications.
Students are asked to solve a design problem in which the length of wall used in a rectangular floor plan is minimized.
Students are given a grid with three points (vertices of a right triangle) representing the starting locations of three sprinters in a race and are asked to determine the center of the finish circle, which is equidistant from each sprinter.
Student Resources
Perspectives Video: Professional/Enthusiast
<p>See and see far into the future of arts and manufacturing as a technician explains computer numerically controlled (CNC) machining bit by bit.</p>
Type: Perspectives Video: Professional/Enthusiast
Problem-Solving Tasks
Using a chart of diameters of different denominations of coins, students are asked to figure out how many coins fit around a central coin. (For this task, United States coins are used, but the task can be adapted for coins from other countries.)
Type: Problem-Solving Task
In this task, a typographic grid system serves as the background for a standard paper clip. A metric measurement scale is drawn across the bottom of the grid and the paper clip extends in both directions slightly beyond the grid. Students are given the approximate length of the paper clip and determine the number of like paper clips made from a given length of wire.
Type: Problem-Solving Task
In this task, students will provide a sketch of a paper ice cream cone wrapper, use the sketch to develop a formula for the surface area of the wrapper, and estimate the maximum number of wrappers that could be cut from a rectangular piece of paper.
Type: Problem-Solving Task
Parent Resources
Perspectives Video: Professional/Enthusiast
<p>See and see far into the future of arts and manufacturing as a technician explains computer numerically controlled (CNC) machining bit by bit.</p>
Type: Perspectives Video: Professional/Enthusiast
Problem-Solving Tasks
Using a chart of diameters of different denominations of coins, students are asked to figure out how many coins fit around a central coin. (For this task, United States coins are used, but the task can be adapted for coins from other countries.)
Type: Problem-Solving Task
In this task, a typographic grid system serves as the background for a standard paper clip. A metric measurement scale is drawn across the bottom of the grid and the paper clip extends in both directions slightly beyond the grid. Students are given the approximate length of the paper clip and determine the number of like paper clips made from a given length of wire.
Type: Problem-Solving Task
In this task, students will provide a sketch of a paper ice cream cone wrapper, use the sketch to develop a formula for the surface area of the wrapper, and estimate the maximum number of wrappers that could be cut from a rectangular piece of paper.
Type: Problem-Solving Task









