Word (.docx)
Acrobat (.pdf)
Clusters should not be sorted from Major to Supporting and then taught in that order. To do so would strip the coherence of the mathematical ideas and miss the opportunity to enhance the major work of the grade with the supporting clusters.
- Assessment Limits :
The item stem must include at least one fraction. Ratios may be expressed as fractions, with “:” or with words. Units may be the same or different across the two quantities. - Calculator :
yes
- Context :
allowable
- Test Item #: Sample Item 1
- Question: A recipe used
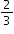cup of sugar for every 2 teaspoons of vanilla. How much sugar
was used per teaspoon of vanilla? - Difficulty: N/A
- Type: MC: Multiple Choice
- Test Item #: Sample Item 2
- Question: A recipe calls fo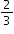r cup of sugar for every 4 teaspoons of vanilla. How much vanilla
should be used for every 1 cup of sugar?
- Difficulty: N/A
- Type: MC: Multiple Choice
- Test Item #: Sample Item 3
- Question: A recipe calls for 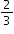cup of sugar for every 2 teaspoons of vanilla. what is the unit
rate in cups per teaspoon?
- Difficulty: N/A
- Type: EE: Equation Editor
- Test Item #: Sample Item 4
- Question: A recipe calls for 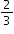cup of sugar for every 4 teaspoons of vanilla. what is the unit
rate in teaspoons per cup?
- Difficulty: N/A
- Type: EE: Equation Editor
- Test Item #: Sample Item 5
- Question:
A recipe calls for
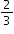cup of sugar for every 
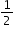teaspoon of vanilla. What is the unit rate of cups per teaspoon? - Difficulty: N/A
- Type: EE: Equation Editor
Related Courses
Related Access Points
Related Resources
Formative Assessments
Lesson Plans
Perspectives Video: Expert
Perspectives Video: Professional/Enthusiasts
Problem-Solving Tasks
Teaching Ideas
Tutorials
STEM Lessons - Model Eliciting Activity
Students at a local middle school are interested in attending a basketball tournament in Orlando. There is an entrance fee and hotel costs to consider. Students must calculate the total cost and the cost per student to attend the tournament. Each hotel has different qualities that could influence the students' choice of which hotel is best for their team.
Model Eliciting Activities, MEAs, are open-ended, interdisciplinary problem-solving activities that are meant to reveal students’ thinking about the concepts embedded in realistic situations. Click here to learn more about MEAs and how they can transform your classroom.
In this Model Eliciting Activities, MEA, students will calculate unit rate & circumference, compare & order decimals, convert metric units, and round decimals. Bubble Burst Corporation has developed some chewing gum prototypes and has requested the students to assist in the selection of which gum prototypes will be mass produced by using both quantitative and qualitative data to rank the prototypes for Bubble Burst Corporation.
Model Eliciting Activities, MEAs, are open-ended, interdisciplinary problem-solving activities that are meant to reveal students’ thinking about the concepts embedded in realistic situations. MEAs resemble engineering problems and encourage students to create solutions in the form of mathematical and scientific models. Students work in teams to apply their knowledge of science and mathematics to solve an open-ended problem while considering constraints and tradeoffs. Students integrate their ELA skills into MEAs as they are asked to clearly document their thought processes. MEAs follow a problem-based, student-centered approach to learning, where students are encouraged to grapple with the problem while the teacher acts as a facilitator. To learn more about MEAs visit: https://www.cpalms.org/cpalms/mea.aspx
Students are presented with the task of evaluating several types of fabric based on each of its characteristics. They need to analyze their current uniform needs and decide by choosing which type of fabric will best fit their uniform needs. Then they have to write a report explaining the procedure they used to analyze their choices, reasoning for their ranking and make the requested recommendations.
Model Eliciting Activities, MEAs, are open-ended, interdisciplinary problem-solving activities that are meant to reveal students’ thinking about the concepts embedded in realistic situations. Click here to learn more about MEAs and how they can transform your classroom.
The principal of Central Middle School is thinking of adding pizza to the lunch menu on Mondays and Fridays but needs help deciding the costs per slice and what students think is important about the pizza. After the students' initial decision about the pizza the principal remembers that there is a delivery charge.The students must revisit their decision and do additional calculations to see if their original process still works.
Model Eliciting Activities, MEAs, are open-ended, interdisciplinary problem-solving activities that are meant to reveal students’ thinking about the concepts embedded in realistic situations. Click here to learn more about MEAs and how they can transform your classroom.
In this 7th grade MEA Laura Banks requests a consulting firm, JJ Consulting, to help her make a decision on an employer. Students are to use the data table to calculate unit rates (nightly rate and hourly rate) and then rank her choices and write a recommendation with the procedure used to come up with the ranking.
Model Eliciting Activities, MEAs, are open-ended, interdisciplinary problem-solving activities that are meant to reveal students’ thinking about the concepts embedded in realistic situations. Click here to learn more about MEAs and how they can transform your classroom.
MFAS Formative Assessments
Students are asked to compute and interpret unit rates in two different ways from values that include fractions and mixed numbers.
Students are asked to convert a ratio of mixed numbers to a unit rate and explain its contextual meaning.
Student Resources
Problem-Solving Tasks
Students are asked to use proportional reasoning to answer a series of questions in the context of a recipe.
Type: Problem-Solving Task
This task asks students to solve a problem in a context involving constant speed. This task provides a transition from working with ratios involving whole numbers to ratios involving fractions. This problem can be thought of in several ways; in particular, this problem also provides an opportunity for students to work with the "How many in one group?'' interpretation of division.
Type: Problem-Solving Task
This problem requires a comparison of rates where one is given in terms of unit rates, and the other is not. See "Music Companies, Variation 2" for a task with a very similar setup but is much more involved and so illustrates .
Type: Problem-Solving Task
This activity asks the student to use unit rate and proportional reasoning to determine which of two runners is the fastest.
Type: Problem-Solving Task
Tutorials
This video demonstrates finding a unit rate from a rate containing fractions.
Type: Tutorial
Watch as we solve a rate problem finding speed in meters per second using distance (in meters) and time (in seconds).
Type: Tutorial
In this lesson, students will be viewing a Khan Academy video that will show how to convert ratios using speed units.
Type: Tutorial
Parent Resources
Problem-Solving Tasks
Students are asked to use proportional reasoning to answer a series of questions in the context of a recipe.
Type: Problem-Solving Task
This task asks students to solve a problem in a context involving constant speed. This task provides a transition from working with ratios involving whole numbers to ratios involving fractions. This problem can be thought of in several ways; in particular, this problem also provides an opportunity for students to work with the "How many in one group?'' interpretation of division.
Type: Problem-Solving Task
This problem requires a comparison of rates where one is given in terms of unit rates, and the other is not. See "Music Companies, Variation 2" for a task with a very similar setup but is much more involved and so illustrates .
Type: Problem-Solving Task
This activity asks the student to use unit rate and proportional reasoning to determine which of two runners is the fastest.
Type: Problem-Solving Task
