Examples
The graph of the function 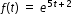 can be transformed into the straight line y=5t+2 by taking the natural logarithm of the function’s outputs.
can be transformed into the straight line y=5t+2 by taking the natural logarithm of the function’s outputs.
Clarifications
Clarification 1: Key features are limited to domain; range; intercepts; intervals where the function is increasing, decreasing, positive or negative; constant percent rate of change; end behavior and asymptotes.Clarification 2: Instruction includes representing the domain, range and constraints with inequality notation, interval notation or set-builder notation.
Clarification 3: Instruction includes understanding that when the logarithm of the dependent variable is taken and graphed, the exponential function will be transformed into a linear function.
Clarification 4: Within the Mathematics for Data and Financial Literacy course, problem types focus on money and business.
Benchmark Instructional Guide
Connecting Benchmarks/Horizontal Alignment
- MA.912.NSO.1.2
- MA.912.NSO.1.7
- MA.912.AR.1.1
- MA.912.AR.1.2
- MA.912.AR.2.5
- MA.912.AR.3.8
- MA.912.AR.9.10
- MA.912.AR.10.2
- MA.912.F.1.2
- MA.912.F.3.2
- MA.912.FL.3
- MA.912.FL.4
Terms from the K-12 Glossary
- Domain
- Exponent
- Exponential function
- Function
- Set-builder notation
Vertical Alignment
Previous Benchmarks
Next Benchmarks
Purpose and Instructional Strategies
In Algebra I, students worked with exponential functions in limited forms. In Math for Data and Financial Literacy, students solve problems modeled with exponential functions.- Instruction focuses on real-world contexts that require students to create a function as a tool to determine requested information or should provide the group a function that models the context.
- For example, students can use exponential decay when analyzing the historical depreciation of a car. In MA.912.AR.2.5, students find the straight-line depreciation. In this benchmark, students could find the percentage the car depreciates instead of the dollar amount (exponential depreciation) using the equation = A(1 − ), where A is the starting value of the car, is the percent of depreciation, is the elapsed time in years, and is the value of the car after years.
- Instruction includes making the connection to logarithms when working with investments formulas involving exponents.
- For example, when using the formula A = P(1 + )
n t r n t - For example, when using the formula A = P
e r t r t 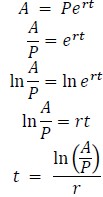
- For example, when using the formula A = P(1 + )
- Instruction includes the use of technology and various representations of functions when solving problems. Given the complexity of the formulas in this course, it may be helpful for students to solve certain problems graphically rather than algebraically. Students can explore entering equations using graphing software.
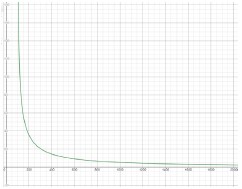
- For example, if Sue deposited $5,000 into an account that compounds interest monthly with an annual rate (APR) of 1.5%, students can determine how long it will take until the account reaches $30,000 algebraically or graphically. To
determine this graphically, students can graph the function
y 0.015 12 t y t - For example, students can graph the equation 20000 =
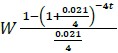 to
explore the relationship between the withdrawal amount and the time it takes to fully deplete a $20,000 balance from an account that compounds quarterly at 2.1%.
to
explore the relationship between the withdrawal amount and the time it takes to fully deplete a $20,000 balance from an account that compounds quarterly at 2.1%.
- For example, if Sue deposited $5,000 into an account that compounds interest monthly with an annual rate (APR) of 1.5%, students can determine how long it will take until the account reaches $30,000 algebraically or graphically. To
determine this graphically, students can graph the function
- Problem types include using instances when periodic deposits are made into an account, and using formulas to find the term of systematic savings account. The formula to calculate the future value of a periodic deposit investment is B =
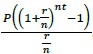 , and the formula for the present value of a periodic deposit investment is P =
, and the formula for the present value of a periodic deposit investment is P = 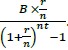 .
. - Problem types include using formulas to find the term of systematic withdrawals, which is when withdrawals are made at regular intervals. The formula to figure out the present
value is P =
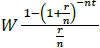 .
. - Be sure to highlight the relative ease and usefulness of graphing financial formulas to explore relationships. Graphs provide the visual data from an infinite number of calculations, saving students time and hassle when exploring relationships between variables.
- Instruction includes making connections to various forms of exponential functions to show their equivalency. Students should understand and interpret when solving the exponential in one form might be more useful than solving in another form depending on context.
- Instruction includes the use of
x y - Instruction includes representing domain, range and intervals where the function is
increasing, decreasing, positive or negative, using words, inequality notation, set-builder notation and interval notation.
- Words
If the domain is all real numbers, it can be written as “all real numbers” or “any value ofx x - Inequality notation
If the domain is all values ofx x - Set-builder notation
If the range is all values ofy y y y y - Interval notation
If the domain is all values ofx x
- Words
Common Misconceptions or Errors
- Ensure students are plugging the correct information into the formulas and using technology correctly. Grouping symbols on graphic software can be tricky. Model the entry of a few formulas with grouping symbols for students before releasing them to explore their own.
- Students may confuse finding the logarithm and natural logarithm.
Instructional Tasks
Instructional Task 1 (MTR.3.1, MTR.4.1)- Luisa deposited $15,000 into a savings account, which compounds interest monthly with an annual rate (APR) of 1.15%. Each month, she withdraws $350 from this account to pay for travel expenses.
- Part A. How long will it take until the account is fully depleted?
- Part B. How can she change the amount she withdrawals so her account lasts twice as long?
Instructional Task 2 (MTR.7.1)
- Han wants to set up a periodic investment account to save for a future car purchase. His goal is to save $40,000. The best account he’s found pays a 2.3% annual interest rate, compounded monthly. Use the periodic investment formula below to graph a relationship between the amount of his monthly periodic investment, P, and the time it will take to achieve his goal,
t
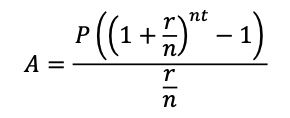
Instructional Items
Instructional Item 1- Mindy purchased a 3-year-old car for $22,300. When the car was new, it sold for $35,500. Find the depreciation rate to the nearest hundredth of a percent.
Related Courses
Related Access Points
Related Resources
Formative Assessment
Lesson Plans
Original Student Tutorials
Perspectives Video: Professional/Enthusiast
Problem-Solving Tasks
MFAS Formative Assessments
Students are asked to interpret parameters of an exponential function in context.
Original Student Tutorials Mathematics - Grades 9-12
Learn about exponential functions and how they are different from linear functions by examining real world situations, their graphs and their tables in this interactive tutorial.
Learn about exponential growth in the context of interest earned as money is put in a savings account by examining equations, graphs, and tables in this interactive tutorial.
Learn about exponential decay as you calculate the value of used cars by examining equations, graphs, and tables in this interactive tutorial.
Student Resources
Original Student Tutorials
Learn about exponential decay as you calculate the value of used cars by examining equations, graphs, and tables in this interactive tutorial.
Type: Original Student Tutorial
Learn about exponential growth in the context of interest earned as money is put in a savings account by examining equations, graphs, and tables in this interactive tutorial.
Type: Original Student Tutorial
Learn about exponential functions and how they are different from linear functions by examining real world situations, their graphs and their tables in this interactive tutorial.
Type: Original Student Tutorial
Problem-Solving Tasks
The coffee cooling experiment is a popular example of an exponential model with immediate appeal. The model is realistic and provides a good context for students to practice work with exponential equations.
Type: Problem-Solving Task
This problem complements the problem "Do two points always determine a linear function?'' There are two constraints on a pair of points R1 and R2 if there is an exponential function f(x) = ae^bx whose graph contains R1 and R2.
Type: Problem-Solving Task
This task gives students an opportunity to work with exponential functions in a real world context involving continuously compounded interest. They will study how the base of the exponential function impacts its growth rate and use logarithms to solve exponential equations.
Type: Problem-Solving Task
This exploratory task requires the student to use properties of exponential functions in order to estimate how much Carbon 14 remains in a preserved plant after different amounts of time.
Type: Problem-Solving Task
In the task "Carbon 14 Dating" the amount of Carbon 14 in a preserved plant is studied as time passes after the plant has died. In practice, however, scientists wish to determine when the plant died, and as this task shows, that is not possible with a simple measurement of the amount of Carbon 14 remaining in the preserved plant.
Type: Problem-Solving Task
The task requires the student to use logarithms to solve an exponential equation in the realistic context of carbon dating, important in archaeology and geology, among other places. Students should be guided to recognize the use of the natural logarithm when the exponential function has the given base of e, as in this problem. Note that the purpose of this task is algebraic in nature -- closely related tasks exist which approach similar problems from numerical or graphical stances.
Type: Problem-Solving Task
This task involves a fairly straightforward decaying exponential. Filling out the table and developing the general formula is complicated only by the need to work with a fraction that requires decisions about rounding and precision.
Type: Problem-Solving Task
This task provides a real world context for interpreting and solving exponential equations. There are two solutions provided for part (a). The first solution demonstrates how to deduce the conclusion by thinking in terms of the functions and their rates of change. The second approach illustrates a rigorous algebraic demonstration that the two populations can never be equal.
Type: Problem-Solving Task
This task provides an interesting context to ask students to estimate values in an exponential function using a graph.
Type: Problem-Solving Task
Parent Resources
Problem-Solving Tasks
The coffee cooling experiment is a popular example of an exponential model with immediate appeal. The model is realistic and provides a good context for students to practice work with exponential equations.
Type: Problem-Solving Task
This problem complements the problem "Do two points always determine a linear function?'' There are two constraints on a pair of points R1 and R2 if there is an exponential function f(x) = ae^bx whose graph contains R1 and R2.
Type: Problem-Solving Task
This task gives students an opportunity to work with exponential functions in a real world context involving continuously compounded interest. They will study how the base of the exponential function impacts its growth rate and use logarithms to solve exponential equations.
Type: Problem-Solving Task
This exploratory task requires the student to use properties of exponential functions in order to estimate how much Carbon 14 remains in a preserved plant after different amounts of time.
Type: Problem-Solving Task
In the task "Carbon 14 Dating" the amount of Carbon 14 in a preserved plant is studied as time passes after the plant has died. In practice, however, scientists wish to determine when the plant died, and as this task shows, that is not possible with a simple measurement of the amount of Carbon 14 remaining in the preserved plant.
Type: Problem-Solving Task
The task requires the student to use logarithms to solve an exponential equation in the realistic context of carbon dating, important in archaeology and geology, among other places. Students should be guided to recognize the use of the natural logarithm when the exponential function has the given base of e, as in this problem. Note that the purpose of this task is algebraic in nature -- closely related tasks exist which approach similar problems from numerical or graphical stances.
Type: Problem-Solving Task
This task involves a fairly straightforward decaying exponential. Filling out the table and developing the general formula is complicated only by the need to work with a fraction that requires decisions about rounding and precision.
Type: Problem-Solving Task
This task provides a real world context for interpreting and solving exponential equations. There are two solutions provided for part (a). The first solution demonstrates how to deduce the conclusion by thinking in terms of the functions and their rates of change. The second approach illustrates a rigorous algebraic demonstration that the two populations can never be equal.
Type: Problem-Solving Task
This task provides an interesting context to ask students to estimate values in an exponential function using a graph.
Type: Problem-Solving Task









