Related Benchmarks
Related Access Points
Access Points
Related Resources
Educational Game
Formative Assessments
Lesson Plans
Problem-Solving Tasks
Tutorial
Student Resources
Educational Game
Test your fraction skills by answering questions on this site. This quiz asks you to simplify fractions, convert fractions to decimals and percentages, and answer algebra questions involving fractions. You can even choose difficulty level, question types, and time limit.
Type: Educational Game
Problem-Solving Tasks
The student is asked to complete a long division which results in a repeating decimal, and then use multiplication to "check" their answer. The purpose of the task is to have students reflect on the meaning of repeating decimal representation through approximation.
Type: Problem-Solving Task
By definition, the square root of a number n is the number you square to get n. The purpose of this task is to have students use the meaning of a square root to find a decimal approximation of a square root of a non-square integer. Students may need guidance in thinking about how to approach the task.
Type: Problem-Solving Task
Requires students to "convert a decimal expansion which repeats eventually into a rational number." Despite this choice of wording, the numbers in this task are rational numbers regardless of the choice of representation. For example, 0.333¯ and 1/3 are two different ways of representing the same number.
Type: Problem-Solving Task
Tutorial
Students will learn that non-zero numbers to the zero power equal one. They will also learn that zero to any positive exponent equals zero.
Type: Tutorial
Parent Resources
Problem-Solving Tasks
The student is asked to complete a long division which results in a repeating decimal, and then use multiplication to "check" their answer. The purpose of the task is to have students reflect on the meaning of repeating decimal representation through approximation.
Type: Problem-Solving Task
This task requires students to work with very large and small values expressed both in scientific notation and in decimal notation (standard form). In addition, students need to convert units of mass. The solution below converts the mass of humans into grams; however, we could just as easily converted the mass of ants into kilograms. Students are unable to go directly to a calculator without taking into account all of the considerations mentioned above. Even after converting units and decimals to scientific notation, students should be encouraged to use the structure of scientific notation to regroup the products by extending the properties of operations and then use the properties of exponents to more fluently perform the calculations involved rather than rely heavily on a calculator.
Type: Problem-Solving Task
By definition, the square root of a number n is the number you square to get n. The purpose of this task is to have students use the meaning of a square root to find a decimal approximation of a square root of a non-square integer. Students may need guidance in thinking about how to approach the task.
Type: Problem-Solving Task
Requires students to "convert a decimal expansion which repeats eventually into a rational number." Despite this choice of wording, the numbers in this task are rational numbers regardless of the choice of representation. For example, 0.333¯ and 1/3 are two different ways of representing the same number.
Type: Problem-Solving Task
In this task, students explore some important mathematical implications of using a calculator. Specifically, they experiment with the approximation of common irrational numbers such as pi (p) and the square root of 2 (v2) and discover how to properly use the calculator for best accuracy. Other related activities involve converting fractions to decimal form and a concrete example where rounding and then multiplying does not yield the same answer as multiplying and then rounding.
Type: Problem-Solving Task






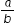 by completing an exploration worksheet. They will conclude that any number which can be written in this form is called a rational number.
by completing an exploration worksheet. They will conclude that any number which can be written in this form is called a rational number.
