Clusters should not be sorted from Major to Supporting and then taught in that order. To do so would strip the coherence of the mathematical ideas and miss the opportunity to enhance the major work of the grade with the supporting clusters.
Related Standards
Related Access Points
Access Points
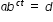
Related Resources
Formative Assessments
Lesson Plans
Original Student Tutorials
Perspectives Video: Expert
Perspectives Video: Professional/Enthusiasts
Problem-Solving Tasks
Professional Development
Unit/Lesson Sequences
Video/Audio/Animation
Virtual Manipulatives
Student Resources
Original Student Tutorials
Learn about exponential growth in the context of interest earned as money is put in a savings account by examining equations, graphs, and tables in this interactive tutorial.
Type: Original Student Tutorial
Follow as we construct an exponential function from a graph, from a table of values, and from a description of a relationship in the real world in this interactive tutorial.
Type: Original Student Tutorial
Problem-Solving Tasks
In this example, students are asked to write a function describing the population growth of algae. It is implied that this is exponential growth.
Type: Problem-Solving Task
This problem solving task challenges students to find the linear, exponential and quadratic functions based on two points.
Type: Problem-Solving Task
This problem solving task asks students to predict and model US population based on a chart of US population data from 1982 to 1988.
Type: Problem-Solving Task
This problem solving task asks students to solve five exponential and linear function problems based on a US population chart for the years 1790-1860.
Type: Problem-Solving Task
This problem solving tasks asks students to find the values of points on a graph.
Type: Problem-Solving Task
This problem solving task asks students to graph a function and find the values of points on a graph.
Type: Problem-Solving Task
The purpose of this task is to give students experience modeling a real-world example of exponential growth, in a context that provides a vivid illustration of the power of exponential growth, for example the cost of inaction for a year. There is an opportunity for further discussion based on part (c), since the ratio of costs from one year to the next is the same in each part.
Type: Problem-Solving Task
The task provides an opportunity for students to engage in detailed analysis of the rate of change of the elevation.
Type: Problem-Solving Task
This problem is an exponential function example that uses the real-world problem of how fast rumors spread.
Type: Problem-Solving Task
The purpose of this task is to give students an opportunity to explore various aspects of exponential models (e.g., distinguishing between constant absolute growth and constant relative growth, solving equations using logarithms, applying compound interest formulas) in the context of a real world problem with ties to developing financial literacy skills. In particular, students are introduced to the idea of inflation of prices of a single commodity, and are given a very brief introduction to the notion of the Consumer Price Index for measuring inflation of a body of goods.
Type: Problem-Solving Task
The coffee cooling experiment is a popular example of an exponential model with immediate appeal. The model is realistic and provides a good context for students to practice work with exponential equations.
Type: Problem-Solving Task
This task gives a variation of real-life contexts which could be modeled by a linear or exponential function. The key distinguishing feature between the two is whether the change by equal factors over equal intervals (exponential functions), or by a constant increase per unit interval (linear functions). The task could either be used as an assessment problem on this distinction, or used as an introduction to the differences between these very important classes of functions.
Type: Problem-Solving Task
This task requires students to use the fact that on the graph of the linear function h(x) = ax + b, the y-coordinate increases by a when x increases by one. Specific values for a and b were left out intentionally to encourage students to use the above fact as opposed to computing the point of intersection, (p,q), and then computing respective function values to answer the question.
Type: Problem-Solving Task
This problem complements the problem "Do two points always determine a linear function?'' There are two constraints on a pair of points R1 and R2 if there is an exponential function f(x) = ae^bx whose graph contains R1 and R2.
Type: Problem-Solving Task
In a geometric context, two distinct points ??1 and ??2 always determine a unique line in the Cartesian plane (this is one of the basic postulates of Euclidean geometry). Only the non-vertical lines, however, can be described by the graph of a function ??(??)=????+??. This task focuses on producing an explicit function ??(??) as long as the line is not vertical.
This problem allows the student to think geometrically about lines and then relate this geometry to linear functions. Or the student can work algebraically with equations to find the explicit equation of the line through two points (when that line is not vertical).
Type: Problem-Solving Task
This task gives students an opportunity to work with exponential functions in a real world context involving continuously compounded interest. They will study how the base of the exponential function impacts its growth rate and use logarithms to solve exponential equations.
Type: Problem-Solving Task
This exploratory task requires the student to use properties of exponential functions in order to estimate how much Carbon 14 remains in a preserved plant after different amounts of time.
Type: Problem-Solving Task
This problem introduces the method used by scientists to date certain organic material. It is based not on the amount of the Carbon 14 isotope remaining in the sample but rather on the ratio of Carbon 14 to Carbon 12. This ratio decreases, hypothetically, at a constant exponential rate as soon as the organic material has ceased to absorb Carbon 14, that is, as soon as it dies.
Type: Problem-Solving Task
The task requires the student to use logarithms to solve an exponential equation in the realistic context of carbon dating, important in archaeology and geology, among other places. Students should be guided to recognize the use of the natural logarithm when the exponential function has the given base of e, as in this problem. Note that the purpose of this task is algebraic in nature -- closely related tasks exist which approach similar problems from numerical or graphical stances.
Type: Problem-Solving Task
This task involves a fairly straightforward decaying exponential. Filling out the table and developing the general formula is complicated only by the need to work with a fraction that requires decisions about rounding and precision.
Type: Problem-Solving Task
This task provides a real world context for interpreting and solving exponential equations. There are two solutions provided for part (a). The first solution demonstrates how to deduce the conclusion by thinking in terms of the functions and their rates of change. The second approach illustrates a rigorous algebraic demonstration that the two populations can never be equal.
Type: Problem-Solving Task
In this task students use verbal descriptions to construct and compare linear and exponential functions and to find where the two functions intersect (F-LE.2, F-LE.3, A-REI.11).
Type: Problem-Solving Task
The purpose of this task it to have students discover how (and how quickly) an exponentially increasing quantity eventually surpasses a linearly increasing quantity. Students' intuitions will probably have them favoring Option A for much longer than is actually the case, especially if they are new to the phenomenon of exponential growth. Teachers might use this surprise as leverage to segue into a more involved task comparing linear and exponential growth.
Type: Problem-Solving Task
This task requires students to use the fact that the value of an exponential function f(x) = a · b^x increases by a multiplicative factor of b when x increases by one. It intentionally omits specific values for c and d in order to encourage students to use this fact instead of computing the point of intersection, (p,q), and then computing function values to answer the question.
Type: Problem-Solving Task
This problem assumes that students are familiar with the notation x0 and ?x. However, the language "successive quotient" may be new.
Type: Problem-Solving Task
This task assumes that students are familiar with the ?x and ?y notations. Students most likely developed this familiarity in their work with slope.
Type: Problem-Solving Task
An important property of linear functions is that they grow by equal differences over equal intervals. In F.LE Equal Differences over Equal Intervals 1, students prove this for equal intervals of length one unit, and note that in this case the equal differences have the same value as the slope.
Type: Problem-Solving Task
This problem-solving task asks students to examine if linear modeling would be appropriate to describe and predict population growth from select years.
Type: Problem-Solving Task
This problem-solving task provides students an opportunity to experiment with modeling real data by using population growth rates from the past two centuries.
Type: Problem-Solving Task
This problem-solving task challenges students to write expressions and create a table to calculate how much money can be gained after investing at different banks with different interest rates.
Type: Problem-Solving Task
This problem-solving task asks students to describe exponential growth through a real-world problem involving the illegal introduction of fish into a lake.
Type: Problem-Solving Task
This task asks students to write equations to predict how much money will be in a savings account at the end of each year, based on different factors like interest rates.
Type: Problem-Solving Task
This problem-solving emphasizes the expectation that students know linear functions grow by constant differences over equal intervals and exponential functions grow by constant factors over equal intervals.
Type: Problem-Solving Task
This problem solving task shows that an exponential function takes larger values than a cubic polynomial function provided the input is sufficiently large. This resource also includes standards alignment commentary and annotated solutions.
Type: Problem-Solving Task
This task asks students to calculate exponential functions with a base larger than one.
Type: Problem-Solving Task
Virtual Manipulatives
In this activity, students plug values into the independent variable to see what the output is for that function. Then based on that information, they have to determine the coefficient (slope) and constant(y-intercept) for the linear function. This activity allows students to explore linear functions and what input values are useful in determining the linear function rule. This activity includes supplemental materials, including background information about the topics covered, a description of how to use the application, and exploration questions for use with the Java applet.
Type: Virtual Manipulative
Using this virtual manipulative, students are able to graph a function and a set of ordered pairs on the same coordinate plane. The constants, coefficients, and exponents can be adjusted using slider bars, so the student can explore the affect on the graph as the function parameters are changed. Students can also examine the deviation of the data from the function. This activity includes supplemental materials, including background information about the topics covered, a description of how to use the application, and exploration questions for use with the java applet.
Type: Virtual Manipulative
In this online tool, students input a function to create a graph where the constants, coefficients, and exponents can be adjusted by slider bars. This tool allows students to explore graphs of functions and how adjusting the numbers in the function affect the graph. Using tabs at the top of the page you can also access supplemental materials, including background information about the topics covered, a description of how to use the application, and exploration questions for use with the java applet.
Type: Virtual Manipulative
This interactive simulation investigates graphing linear and quadratic equations. Users are given the ability to define and change the coefficients and constants in order to observe resulting changes in the graph(s).
Type: Virtual Manipulative
Parent Resources
Problem-Solving Tasks
In this example, students are asked to write a function describing the population growth of algae. It is implied that this is exponential growth.
Type: Problem-Solving Task
This problem solving task challenges students to find the linear, exponential and quadratic functions based on two points.
Type: Problem-Solving Task
This problem solving task asks students to predict and model US population based on a chart of US population data from 1982 to 1988.
Type: Problem-Solving Task
This problem solving task asks students to solve five exponential and linear function problems based on a US population chart for the years 1790-1860.
Type: Problem-Solving Task
This problem solving tasks asks students to find the values of points on a graph.
Type: Problem-Solving Task
This problem solving task asks students to graph a function and find the values of points on a graph.
Type: Problem-Solving Task
The purpose of this task is to give students experience modeling a real-world example of exponential growth, in a context that provides a vivid illustration of the power of exponential growth, for example the cost of inaction for a year. There is an opportunity for further discussion based on part (c), since the ratio of costs from one year to the next is the same in each part.
Type: Problem-Solving Task
The task provides an opportunity for students to engage in detailed analysis of the rate of change of the elevation.
Type: Problem-Solving Task
This problem is an exponential function example that uses the real-world problem of how fast rumors spread.
Type: Problem-Solving Task
The purpose of this task is to give students an opportunity to explore various aspects of exponential models (e.g., distinguishing between constant absolute growth and constant relative growth, solving equations using logarithms, applying compound interest formulas) in the context of a real world problem with ties to developing financial literacy skills. In particular, students are introduced to the idea of inflation of prices of a single commodity, and are given a very brief introduction to the notion of the Consumer Price Index for measuring inflation of a body of goods.
Type: Problem-Solving Task
The coffee cooling experiment is a popular example of an exponential model with immediate appeal. The model is realistic and provides a good context for students to practice work with exponential equations.
Type: Problem-Solving Task
This task gives a variation of real-life contexts which could be modeled by a linear or exponential function. The key distinguishing feature between the two is whether the change by equal factors over equal intervals (exponential functions), or by a constant increase per unit interval (linear functions). The task could either be used as an assessment problem on this distinction, or used as an introduction to the differences between these very important classes of functions.
Type: Problem-Solving Task
This task requires students to use the fact that on the graph of the linear function h(x) = ax + b, the y-coordinate increases by a when x increases by one. Specific values for a and b were left out intentionally to encourage students to use the above fact as opposed to computing the point of intersection, (p,q), and then computing respective function values to answer the question.
Type: Problem-Solving Task
This problem complements the problem "Do two points always determine a linear function?'' There are two constraints on a pair of points R1 and R2 if there is an exponential function f(x) = ae^bx whose graph contains R1 and R2.
Type: Problem-Solving Task
In a geometric context, two distinct points ??1 and ??2 always determine a unique line in the Cartesian plane (this is one of the basic postulates of Euclidean geometry). Only the non-vertical lines, however, can be described by the graph of a function ??(??)=????+??. This task focuses on producing an explicit function ??(??) as long as the line is not vertical.
This problem allows the student to think geometrically about lines and then relate this geometry to linear functions. Or the student can work algebraically with equations to find the explicit equation of the line through two points (when that line is not vertical).
Type: Problem-Solving Task
This task gives students an opportunity to work with exponential functions in a real world context involving continuously compounded interest. They will study how the base of the exponential function impacts its growth rate and use logarithms to solve exponential equations.
Type: Problem-Solving Task
This exploratory task requires the student to use properties of exponential functions in order to estimate how much Carbon 14 remains in a preserved plant after different amounts of time.
Type: Problem-Solving Task
This problem introduces the method used by scientists to date certain organic material. It is based not on the amount of the Carbon 14 isotope remaining in the sample but rather on the ratio of Carbon 14 to Carbon 12. This ratio decreases, hypothetically, at a constant exponential rate as soon as the organic material has ceased to absorb Carbon 14, that is, as soon as it dies.
Type: Problem-Solving Task
The task requires the student to use logarithms to solve an exponential equation in the realistic context of carbon dating, important in archaeology and geology, among other places. Students should be guided to recognize the use of the natural logarithm when the exponential function has the given base of e, as in this problem. Note that the purpose of this task is algebraic in nature -- closely related tasks exist which approach similar problems from numerical or graphical stances.
Type: Problem-Solving Task
This task involves a fairly straightforward decaying exponential. Filling out the table and developing the general formula is complicated only by the need to work with a fraction that requires decisions about rounding and precision.
Type: Problem-Solving Task
This task provides a real world context for interpreting and solving exponential equations. There are two solutions provided for part (a). The first solution demonstrates how to deduce the conclusion by thinking in terms of the functions and their rates of change. The second approach illustrates a rigorous algebraic demonstration that the two populations can never be equal.
Type: Problem-Solving Task
In this task students use verbal descriptions to construct and compare linear and exponential functions and to find where the two functions intersect (F-LE.2, F-LE.3, A-REI.11).
Type: Problem-Solving Task
The purpose of this task it to have students discover how (and how quickly) an exponentially increasing quantity eventually surpasses a linearly increasing quantity. Students' intuitions will probably have them favoring Option A for much longer than is actually the case, especially if they are new to the phenomenon of exponential growth. Teachers might use this surprise as leverage to segue into a more involved task comparing linear and exponential growth.
Type: Problem-Solving Task
This task requires students to use the fact that the value of an exponential function f(x) = a · b^x increases by a multiplicative factor of b when x increases by one. It intentionally omits specific values for c and d in order to encourage students to use this fact instead of computing the point of intersection, (p,q), and then computing function values to answer the question.
Type: Problem-Solving Task
This problem assumes that students are familiar with the notation x0 and ?x. However, the language "successive quotient" may be new.
Type: Problem-Solving Task
This task assumes that students are familiar with the ?x and ?y notations. Students most likely developed this familiarity in their work with slope.
Type: Problem-Solving Task
An important property of linear functions is that they grow by equal differences over equal intervals. In F.LE Equal Differences over Equal Intervals 1, students prove this for equal intervals of length one unit, and note that in this case the equal differences have the same value as the slope.
Type: Problem-Solving Task
This problem-solving task asks students to examine if linear modeling would be appropriate to describe and predict population growth from select years.
Type: Problem-Solving Task
This problem-solving task provides students an opportunity to experiment with modeling real data by using population growth rates from the past two centuries.
Type: Problem-Solving Task
This problem-solving task challenges students to write expressions and create a table to calculate how much money can be gained after investing at different banks with different interest rates.
Type: Problem-Solving Task
This problem-solving task asks students to describe exponential growth through a real-world problem involving the illegal introduction of fish into a lake.
Type: Problem-Solving Task
This task asks students to write equations to predict how much money will be in a savings account at the end of each year, based on different factors like interest rates.
Type: Problem-Solving Task
This problem-solving emphasizes the expectation that students know linear functions grow by constant differences over equal intervals and exponential functions grow by constant factors over equal intervals.
Type: Problem-Solving Task
This problem solving task shows that an exponential function takes larger values than a cubic polynomial function provided the input is sufficiently large. This resource also includes standards alignment commentary and annotated solutions.
Type: Problem-Solving Task
This task asks students to calculate exponential functions with a base larger than one.
Type: Problem-Solving Task