MA.8.G.2.3Archived Standard

loading spinner
Export Print
Demonstrate that the sum of the angles in a triangle is 180-degrees and apply this fact to find unknown measure of angles and the sum of angles in polygons.

Remarks

Example 1: "Make a paper triangle and cut off regions around the vertices. Then place the vertices together, meeting at a common point, to see that they form a (approximate) straight angle."

Example 2: In the following diagram, line k is parallel to line l. Use properties of angles made when parallel lines are cut by transverse lines to demonstrate that the sum of the three interior angles of a planar triangle is 180 degrees.


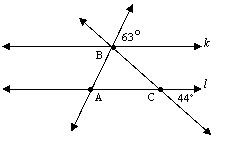
Example 3: Determine the sum of the internal angles of a regular hexagon. Investigate whether this sum is the same or different for different hexagons. Explain your findings.

 

General Information
Subject Area: X-Mathematics (former standards - 2008)
Grade: 8
Body of Knowledge: Geometry
Idea: Level 2: Basic Application of Skills & Concepts
Big Idea: BIG IDEA 2 - Analyze two- and three-dimensional figures by using distance and angle.
Date Adopted or Revised: 09/07
Content Complexity Rating: Level 2: Basic Application of Skills & Concepts - More Information
Date of Last Rating: 06/07
Status: State Board Approved - Archived
Assessed: Yes
Test Item Specifications
  • Item Type(s): This benchmark may be assessed using: MC , GR item(s)

  • Clarification :
    Students will determine the measures of angles in triangles and other polygons when some of the angle measures are given.
  • Content Limits :
    Polygons will not exceed a maximum of eight sides.
  • Stimulus Attributes :
    Graphics should be used in all of these items.

    Items should be set in either a real-world or mathematical context.
Sample Test Items (2)
  • Test Item #: Sample Item 1
  • Question: Lou is studying the design of a honeycomb. The honeycomb design is made up of regular hexagons, as shown below.

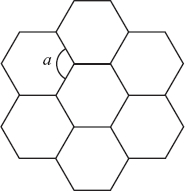
    honeycomb design is made up of regular hexagons


    what is the measure, in degrees, of ∠ a above?
  • Difficulty: N/A
  • Type: MC: Multiple Choice
  • Test Item #: Sample Item 2
  • Question: Vertices Q and T of ΔQTU are located on opposite sides of rectangle PRSU, as shown in the diagram below.

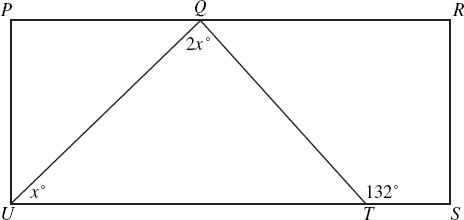
     rectangle


    what is the value of x?
  • Difficulty: N/A
  • Type: GR: Gridded-Response

Related Access Points

Alternate version of this benchmark for students with significant cognitive disabilities.

Related Resources

Vetted resources educators can use to teach the concepts and skills in this benchmark.

Image/Photograph

Clipart: Geometric Shapes:

In this lesson, you will find clip art and various illustrations of polygons, circles, ellipses, star polygons, and inscribed shapes.

Type: Image/Photograph

Lesson Plans

The Ins and Outs of Polygons:

In this lesson, students will explore how to find the sum of the measures of the angles of a triangle, then use this knowledge to find the sum of the measures of angles of other polygons. They will also be able to find the sum of the exterior angles of triangles and other polygons. Using both concepts, students will be able to find missing measurements.

Type: Lesson Plan

How Many Degrees?:

This lesson facilitates the discovery of a formula for the sum of the interior angles of a regular polygon. Students will draw all the diagonals from one vertex of various polygons to find how many triangles are formed. They will use this and their prior knowledge of triangles to figure out the sum of the interior angles. This will lead to the development of a formula for finding the sum of interior angles and the measure of one interior angle.

Type: Lesson Plan

Student Resources

Vetted resources students can use to learn the concepts and skills in this benchmark.

Parent Resources

Vetted resources caregivers can use to help students learn the concepts and skills in this benchmark.

Image/Photograph

Clipart: Geometric Shapes:

In this lesson, you will find clip art and various illustrations of polygons, circles, ellipses, star polygons, and inscribed shapes.

Type: Image/Photograph