Clusters should not be sorted from Major to Supporting and then taught in that order. To do so would strip the coherence of the mathematical ideas and miss the opportunity to enhance the major work of the grade with the supporting clusters.
- Assessment Limits :
Items may require the student to recall the formula for the volume of
a sphere.Items may require the student to find a dimension.
Items that involve cones, cylinders, and spheres should require the
student to do more than just find the volume.Items may include composite figures, including three-dimensional
figures previously learned.Items may not include oblique figures.
Items may require the student to find the volume when one or more
dimensions are changed.Items may require the student to find a dimension when the volume
is changed. - Calculator :
Neutral
- Clarification :
Students will use volume formulas for cylinders, pyramids, cones, and
spheres to solve problems - Stimulus Attributes :
Items must be set in a real-world context.Items may require the student to apply the basic modeling cycle
- Response Attributes :
Items may require the student to use or choose the correct unit of
measure.Items may require the student to apply the basic modeling cycle
- Test Item #: Sample Item 1
- Question:
A phosphate is mined, it moves along a conveyor belt, falling off of the end of the belt into the shape of a right circular cone, as shown.
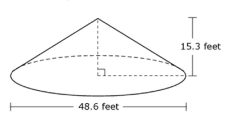
A shorter conveyor belt also has phosphate falling off of the end into the shape of a right circular cone. The height of the second pile of phosphate is 3.6 feet shorter than the height of the first. The volume of both piles is the same.
To the nearest tenth of a foot, what is the diameter of the second pile of phosphate?
- Difficulty: N/A
- Type: EE: Equation Editor
Related Courses
Related Access Points
Related Resources
Formative Assessments
Lesson Plans
Original Student Tutorial
Perspectives Video: Expert
Perspectives Video: Professional/Enthusiasts
Problem-Solving Tasks
Unit/Lesson Sequence
STEM Lessons - Model Eliciting Activity
The student will assist Yogurt Land on choosing a new size container to offer their customers. The choice of containers are different three dimensional figures. Students will revisit the concepts of volume, surface area, and profit in order to make a decision.
Model Eliciting Activities, MEAs, are open-ended, interdisciplinary problem-solving activities that are meant to reveal students’ thinking about the concepts embedded in realistic situations. Click here to learn more about MEAs and how they can transform your classroom.
MFAS Formative Assessments
Students are asked to solve a problem that requires calculating the volumes of a sphere and a cylinder.
Students are asked to solve a problem that requires calculating the volumes of a cone and a cylinder.
Students are asked to solve a problem that requires calculating the volume of a large cylindrical sports drink container and comparing it to the combined volumes of 24 individual containers.
Students are asked to find the height of the Great Pyramid of Giza given its volume and the length of the edge of its square base.
Original Student Tutorials Mathematics - Grades 9-12
Learn to calculate the volume of a cone as you solve real-world problems in this ice cream-themed, interactive tutorial.
Student Resources
Original Student Tutorial
Learn to calculate the volume of a cone as you solve real-world problems in this ice cream-themed, interactive tutorial.
Type: Original Student Tutorial
Perspectives Video: Professional/Enthusiast
<p>You'll need to bring your computer skills and math knowledge to estimate oil volume and rate as it seeps from the ocean floor. Dive in!</p>
Type: Perspectives Video: Professional/Enthusiast
Problem-Solving Tasks
The purpose of the task is to analyze a plausible real-life scenario using a geometric model. The task requires knowledge of volume formulas for cylinders and cones, some geometric reasoning involving similar triangles, and pays attention to reasonable approximations and maintaining reasonable levels of accuracy throughout.
Type: Problem-Solving Task
The purpose of this task is to use geometric and algebraic reasoning to model a real-life scenario. In particular, students are in several places (implicitly or explicitly) to reason as to when making approximations is reasonable and when to round, when to use equalities vs. inequalities, and the choice of units to work with (e.g., mm vs. cm).
Type: Problem-Solving Task
Parent Resources
Perspectives Video: Professional/Enthusiast
<p>You'll need to bring your computer skills and math knowledge to estimate oil volume and rate as it seeps from the ocean floor. Dive in!</p>
Type: Perspectives Video: Professional/Enthusiast
Problem-Solving Tasks
The purpose of the task is to analyze a plausible real-life scenario using a geometric model. The task requires knowledge of volume formulas for cylinders and cones, some geometric reasoning involving similar triangles, and pays attention to reasonable approximations and maintaining reasonable levels of accuracy throughout.
Type: Problem-Solving Task
The purpose of this task is to use geometric and algebraic reasoning to model a real-life scenario. In particular, students are in several places (implicitly or explicitly) to reason as to when making approximations is reasonable and when to round, when to use equalities vs. inequalities, and the choice of units to work with (e.g., mm vs. cm).
Type: Problem-Solving Task








