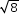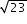Clusters should not be sorted from Major to Supporting and then taught in that order. To do so would strip the coherence of the mathematical ideas and miss the opportunity to enhance the major work of the grade with the supporting clusters.
- Assessment Limits :
All irrational numbers may be used, excluding e. Irrational expressions should only use one operation. - Calculator :
No
- Context :
No context
- Test Item #: Sample Item 1
- Question:
What is the approximate value of
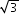 , to the nearest whole number?
, to the nearest whole number? - Difficulty: N/A
- Type: EE: Equation Editor
- Test Item #: Sample Item 2
- Question:
What is the approximate value of
 ?
? - Difficulty: N/A
- Type: MC: Multiple Choice
- Test Item #: Sample Item 3
- Question:
A number line is shown.
Place the following numbers in the proper location on the number line.
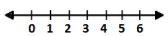
- Difficulty: N/A
- Type: GRID: Graphic Response Item Display
- Test Item #: Sample Item 4
- Question:
Drag the numbers shown to their approximate locations on the number line.
- Difficulty: N/A
- Type: GRID: Graphic Response Item Display
Related Courses
Related Access Points
Related Resources
Formative Assessments
Lesson Plans
Problem-Solving Tasks
Student Center Activity
Tutorials
MFAS Formative Assessments
Students are asked to plot the square root of eight on three number lines, scaled to progressively more precision.
Students are asked to estimate the value of several irrational numbers using a calculator and order them on a number line.
Students are asked to graph three different irrational numbers on number lines.
Students are asked to find and interpret lower and upper bounds of an irrational expression using a calculator.
Student Resources
Problem-Solving Tasks
When students plot irrational numbers on the number line, it helps reinforce the idea that they fit into a number system that includes the more familiar integer and rational numbers. This is a good time for teachers to start using the term "real number line" to emphasize the fact that the number system represented by the number line is the real numbers. When students begin to study complex numbers in high school, they will encounter numbers that are not on the real number line (and are, in fact, on a "number plane"). This task could be used for assessment, or if elaborated a bit, could be used in an instructional setting.
Type: Problem-Solving Task
Students are given a pair of numbers. They are asked to determine which is larger, and then justify their answer. The numbers involved are rational numbers and common irrational numbers, such p and square roots. This task can be used to either build or assess initial understandings related to rational approximations of irrational numbers.
Type: Problem-Solving Task
Student Center Activity
Students can practice answering mathematics questions on a variety of topics. With an account, students can save their work and send it to their teacher when complete.
Type: Student Center Activity
Tutorials
In this video, you will practice comparing an irrational number to a percent. First you will try it without a calculator. Then you will check your answer using a calculator.
Type: Tutorial
In this video, you will learn how to approximate a square root to the hundredths place.
Type: Tutorial
In this video, you will practice approximating square roots of numbers that are not perfect squares. You will find the perfect square below and above to approximate the value of the square root between two whole numbers.
Type: Tutorial
Parent Resources
Problem-Solving Tasks
When students plot irrational numbers on the number line, it helps reinforce the idea that they fit into a number system that includes the more familiar integer and rational numbers. This is a good time for teachers to start using the term "real number line" to emphasize the fact that the number system represented by the number line is the real numbers. When students begin to study complex numbers in high school, they will encounter numbers that are not on the real number line (and are, in fact, on a "number plane"). This task could be used for assessment, or if elaborated a bit, could be used in an instructional setting.
Type: Problem-Solving Task
Students are given a pair of numbers. They are asked to determine which is larger, and then justify their answer. The numbers involved are rational numbers and common irrational numbers, such p and square roots. This task can be used to either build or assess initial understandings related to rational approximations of irrational numbers.
Type: Problem-Solving Task