- Develop a uniform probability model by assigning equal probability to all outcomes, and use the model to determine probabilities of events. For example, if a student is selected at random from a class, find the probability that Jane will be selected and the probability that a girl will be selected.
- Develop a probability model (which may not be uniform) by observing frequencies in data generated from a chance process. For example, find the approximate probability that a spinning penny will land heads up or that a tossed paper cup will land open-end down. Do the outcomes for the spinning penny appear to be equally likely based on the observed frequencies?
Clusters should not be sorted from Major to Supporting and then taught in that order. To do so would strip the coherence of the mathematical ideas and miss the opportunity to enhance the major work of the grade with the supporting clusters.
-
Also Assesses:
- Assessment Limits :
N/A
- Calculator :
Neutral
- Context :
Required
MAFS.7.SP.3.8 Find probabilities of compound events using organized lists, tables, tree diagrams, and simulation.
MAFS.7.SP.3.8a Understand that, just as with simple events, the probability of a compound event is the fraction of outcomes in the sample space for which the compound event occurs.
MAFS.7.SP.3.8b Represent sample spaces for compound events using methods such as organized lists, tables, and tree diagrams. For an event described in everyday language (e.g., “rolling double sixes”), identify the outcomes in the sample space which compose the event.
MAFS.7.SP.3.8c Design and use a simulation to generate frequencies for compound events. For example, use random digits as a simulation tool to approximate the answer to the question: If 40% of donors have type A blood, what is the probability that it will take at least 4 donors to find one with type A blood?
- Test Item #: Sample Item 1
- Question: A bag contains 3 red marbles and 6 blue marbles.
What is the probability of randomly selecting a red marble from the bag?
- Difficulty: N/A
- Type: EE: Equation Editor
- Test Item #: Sample Item 2
- Question: Tony has a bucket filled with 10 green, 3 blue, 1 red, and 7 yellow tennis balls. He
removes 4 tennis balls from the bucket, without replacement.
Which of the following outcomes could represent this selection?
- Difficulty: N/A
- Type: MS: Multiselect
- Test Item #: Sample Item 3
- Question: Select all situations that describe a probability of
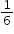 of drawing a red marble out of the
bag.
of drawing a red marble out of the
bag. - Difficulty: N/A
- Type: MS: Multiselect
- Test Item #: Sample Item 4
- Question: A bucket contains 5 green tennis balls and 2 yellow tennis balls. Tony removes 2 tennis
balls, with replacement, from the bucket shown.

What is the probability that Tony will choose a yellow tennis ball and then a green tennis ball?
- Difficulty: N/A
- Type: EE: Equation Editor
- Test Item #: Sample Item 5
- Question: A bucket contains 5 green tennis balls, 2 yellow tennis balls, and 6 red tennis balls. Tony
removes 3 tennis balls, with replacement, from the bucket shown.

What is the probability that the first tennis ball is yellow, the second tennis ball is green, and the third tennis ball is red?
- Difficulty: N/A
- Type: EE: Equation Editor
- Test Item #: Sample Item 6
- Question:
A bag contains 4 red, 4 blue, 4 green, and 4 yellow marbles. A marble is randomly pulled from the bag and replaced seven times. The table shows the outcome of the experiment.
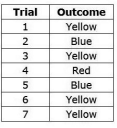
Which marble color's observed frequency is closest to its expected frequency?
- Difficulty: N/A
- Type: MC: Multiple Choice
Related Courses
Related Access Points
Related Resources
Formative Assessments
Image/Photograph
Lesson Plans
Perspectives Video: Experts
Problem-Solving Tasks
Tutorials
Video/Audio/Animation
Virtual Manipulatives
MFAS Formative Assessments
Students are asked to determine the probability of a chance event and explain possible causes for the difference between the probability and observed frequencies.
Students are asked to determine probabilities based on observed outcomes and to determine if the outcomes appear to be equally likely.
Students are asked to determine probabilities based on observed outcomes and to determine if the outcomes appear to be equally likely.
Students are given a scenario and asked to determine the probability of two different events.
Student Resources
Problem-Solving Tasks
This resource involves a simple data-gathering activity which furnishes data that students organize into a table. They are then asked to refer to the data and determine the probability of various outcomes.
Type: Problem-Solving Task
This task is intended as a classroom activity. Students pool the results of many repetitions of the random phenomenon (rolling dice) and compare their results to the theoretical expectation they develop by considering all possible outcomes of rolling two dice. This gives them a concrete example of what we mean by long term relative frequency.
Type: Problem-Solving Task
Tutorials
This video demonstrates several examples of finding probability of random events.
Type: Tutorial
This video compares theoretical and experimantal probabilities and sources of possible discrepancy.
Type: Tutorial
This video demonstrates how to find the probability of a simple event.
Type: Tutorial
Video/Audio/Animation
This 8-minute video provides an introduction to the concept of probability through the example of flipping a coin and rolling a die.
Type: Video/Audio/Animation
Virtual Manipulatives
In this activity, students adjust how many sections there are on a fair spinner then run simulated trials on that spinner as a way to develop concepts of probability. A table next to the spinner displays the theoretical probability for each color section of the spinner and records the experimental probability from the spinning trials. This activity allows students to explore the topics of experimental and theoretical probability by seeing them displayed side by side for the spinner they have created. This activity includes supplemental materials, including background information about the topics covered, a description of how to use the application, and exploration questions for use with the java applet.
Type: Virtual Manipulative
This online manipulative allows the student to simulate placing marbles into a bag and finding the probability of pulling out certain combinations of marbles. This allows exploration of probabilities of multiple events as well as probability with and without replacement. The tabs above the applet provide access to supplemental materials, including background information about the topics covered, a description of how to use the application, and exploration questions for use with the Java applet.
Type: Virtual Manipulative
The students will play a classic game from a popular show. Through this they can explore the probability that the ball will land on each of the numbers and discover that more accurate results coming from repeated testing. The simulation can be adjusted to influence fairness and randomness of the results.
Type: Virtual Manipulative
Parent Resources
Problem-Solving Tasks
This resource involves a simple data-gathering activity which furnishes data that students organize into a table. They are then asked to refer to the data and determine the probability of various outcomes.
Type: Problem-Solving Task
This task is intended as a classroom activity. Students pool the results of many repetitions of the random phenomenon (rolling dice) and compare their results to the theoretical expectation they develop by considering all possible outcomes of rolling two dice. This gives them a concrete example of what we mean by long term relative frequency.
Type: Problem-Solving Task








