Clarifications
Clarification 1: Multi-digit decimals are limited to no more than 5 total digits.Benchmark Instructional Guide
Connecting Benchmarks/Horizontal Alignment
Terms from the K-12 Glossary
- Area Model
- Commutative Property of Multiplication
- Expression
- Dividend
- Divisor
Vertical Alignment
Previous Benchmarks
http://flbt5.floridaearlylearning.com/standards.html
Next Benchmarks
Purpose and Instructional Strategies
In grade 5, students multiplied and divided multi-digit whole numbers and represented remainders as fractions. They also estimated and determined the product and quotient of multi-digit numbers with decimals to the hundredths and multiplied and divided the product and quotient of multi-digit numbers with decimals to the hundredths by one-tenth and one-hundredth with procedural reliability. In grade 6, students multiply and divide positive rational numbers with procedural fluency, including dividing numerators by denominators to rewrite fractions as decimals. In grade 7, students will become fluent in all operations with positive and negative rational numbers.- Instruction includes representing multiplication in various ways.
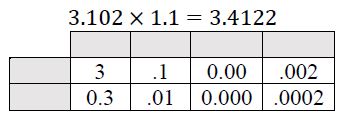
- 3.102 × 1.1 = 3.4122
- (3.102)(1.1) = 3.4122
- 3.102(1.1) = 3.4122
- 3.102 · 1.1 = 3.4122
- Students should continue demonstrating their understanding from grade 5 that division can be represented as a fraction.
- A standard algorithm is a systematic method that students can use accurately, reliably and efficiently (no matter how many digits) depending on the content of the problem. It is not the intention to require students to use a standard algorithm all of the time. However, students are expected to become fluent with a standard algorithm by certain grade levels as stated within the benchmarks.
- Instruction includes a variety of methods and strategies to multiply and divide multi-digit numbers with decimals.
- Area Models
- Partial Products
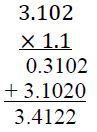
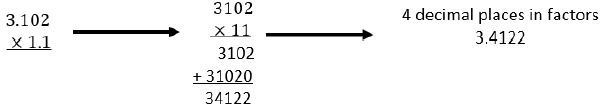
- Multiplying as if the factors are whole numbers and applying the decimal places to the final product based on the number of decimals represented in the factors (MTR.3.1).
- Area Models
- Students should develop fluency with and without the use of a calculator when performing operations with positive decimals.
Common Misconceptions or Errors
- Students may incorrectly apply rules for adding or subtracting decimals to multiplication of decimals, believing place values must be aligned.
- Students may confuse the lining up of place values when multiplying or dividing vertically by omitting or forgetting to include zeros as place holders in the partial products or quotients.
Strategies to Support Tiered Instruction
- Instruction includes the use of estimation to ensure the proper placement of the decimal point in the final product or quotient of decimals.
- For example, if finding the product of 12.3 and 4.8, students should estimate the product to be close to 60, by using 12 and 5 as friendly numbers, then apply the decimal to the actual product of 123 and 48, which is 5904. Based on the estimate, the decimal should be placed after 59 to produce 59.04.
- Teacher encourages and allows for students who have a firm understanding of multiplying and dividing fractions to convert the provided decimal values to their equivalent fractional form before performing the desired operation and converting the solution back to decimal form.
- Teacher provides graph paper to utilize while applying an algorithm for multiplying or dividing to keep numbers lined up and help students focus on place value.
- Instruction includes providing opportunities to reinforce place values with the use of base ten blocks or hundredths grids.
Instructional Tasks
Instructional Task 1 (MTR.6.1)Part A. Complete the table below using a calculator.
Part B. Talk with a partner about what you notice from the table in Part A.
Expression Solution Expression Solution 5.59(5) 325(25) 3.25(2.5) 19(93) 19(9.3)
Part C. How are the expressions without decimals related to the expressions with decimals? Is there a relationship between the decimal placements in the expressions and the solutions?
Part D. If 2368(421) = 996,928, what would you expect 2.368(4.21) be equal to?
Instructional Items
Instructional Item 1Related Courses
Related Access Points
Related Resources
Formative Assessments
Lesson Plans
Perspectives Video: Experts
Perspectives Video: Teaching Idea
Problem-Solving Tasks
Tutorials
STEM Lessons - Model Eliciting Activity
In this Model Eliciting Activity, MEA, students will use the provided data to calculate travel time and total cost after tax. Students will consider this data and other provided criteria to assist a travel agent in determining which airline to choose for a client.
Model Eliciting Activities, MEAs, are open-ended, interdisciplinary problem-solving activities that are meant to reveal students’ thinking about the concepts embedded in realistic situations. Click here to learn more about MEAs and how they can transform your classroom.
This lesson requires students to use mathematical data and logic/reasoning to place vendors into retail spaces in a shopping plaza. Students will first rank five vendor types on their profitability (based on average sales and average overhead/upkeep costs), then place the vendor types into the 11-13 retail spaces. They are also required to find the area of each space and calculate the total leasing charges. The plans for the plaza are given on a coordinate plane, so students will need to find the lengths of horizontal and vertical line segments (using the coordinates of the endpoints) to calculate the areas of the rectangular and composite spaces.
Model Eliciting Activities, MEAs, are open-ended, interdisciplinary problem-solving activities that are meant to reveal students’ thinking about the concepts embedded in realistic situations. MEAs resemble engineering problems and encourage students to create solutions in the form of mathematical and scientific models. Students work in teams to apply their knowledge of science and mathematics to solve an open-ended problem while considering constraints and tradeoffs. Students integrate their ELA skills into MEAs as they are asked to clearly document their thought processes. MEAs follow a problem-based, student-centered approach to learning, where students are encouraged to grapple with the problem while the teacher acts as a facilitator. To learn more about MEAs visit: https://www.cpalms.org/cpalms/mea.aspx
In this Model Eliciting Activity, MEA, students are to decide on a pressure cleaning machine that will provide the Sidewalks and Roof Cleaning Services Incorporated with the best value for their money. Students are asked to provide a "Best Value" pressure cleaner to the company owner and explain how they arrived at their solution.
Model Eliciting Activities are open-ended, interdisciplinary problem-solving activities that are meant to reveal students’ thinking about the concepts embedded in realistic situations. MEAs resemble engineering problems and encourage students to create solutions in the form of mathematical and scientific models. Students work in teams to apply their knowledge of science and mathematics to solve an open-ended problem, while considering constraints and tradeoffs. Students integrate their ELA skills into MEAs as they are asked to clearly document their thought process. MEAs follow a problem-based, student centered approach to learning, where students are encouraged to grapple with the problem while the teacher acts as a facilitator. To learn more about MEA’s visit: https://www.cpalms.org/cpalms/mea.aspx
In this Model Eliciting Activity (MEA), students will use the 4 operations with decimal numbers and calculate profit (including negative numbers) as well as use the resulting data to help a business owner make decisions about their candy stores.
Model Eliciting Activities, MEAs, are open-ended, interdisciplinary problem-solving activities that are meant to reveal students’ thinking about the concepts embedded in realistic situations. MEAs resemble engineering problems and encourage students to create solutions in the form of mathematical and scientific models. Students work in teams to apply their knowledge of science and mathematics to solve an open-ended problem, while considering constraints and tradeoffs. Students integrate their ELA skills into MEAs as they are asked to clearly document their thought process. MEAs follow a problem-based, student-centered approach to learning, where students are encouraged to grapple with the problem while the teacher acts as a facilitator. To learn more about MEA’s visit: https://www.cpalms.org/cpalms/mea.aspx
In this Model Eliciting Activity, MEA Students will look at a data set to determine which vending machine should be placed in a school. Students have to determine profit and consider other provided data.
Model Eliciting Activities, MEAs, are open-ended, interdisciplinary problem-solving activities that are meant to reveal students’ thinking about the concepts embedded in realistic situations. MEAs resemble engineering problems and encourage students to create solutions in the form of mathematical and scientific models. Students work in teams to apply their knowledge of science and mathematics to solve an open-ended problem while considering constraints and tradeoffs. Students integrate their ELA skills into MEAs as they are asked to clearly document their thought processes. MEAs follow a problem-based, student-centered approach to learning, where students are encouraged to grapple with the problem while the teacher acts as a facilitator. To learn more about MEAs visit: https://www.cpalms.org/cpalms/mea.aspx.
In this Model Eliciting Activity, MEA, "Sticks and Stones May Break My Bones", teams of students work as forensic anthropologists and use equations to determine the height and gender of persons to whom a series of newly discovered bones may belong.
Model Eliciting Activities, MEAs, are open-ended, interdisciplinary problem-solving activities that are meant to reveal students’ thinking about the concepts embedded in realistic situations. Click here to learn more about MEAs and how they can transform your classroom.
In the Sugar Scrub MEA students will analyze 5 sugar scrub formulas. In the first part, students are asked to evaluate each formula based on color, scent, and exfoliation. In the second part, students apply their methodology to a cost analysis of the scrubs.
Model Eliciting Activities, MEAs, are open-ended, interdisciplinary problem-solving activities that are meant to reveal students’ thinking about the concepts embedded in realistic situations. MEAs resemble engineering problems and encourage students to create solutions in the form of mathematical and scientific models. Students work in teams to apply their knowledge of science and mathematics to solve an open-ended problem while considering constraints and tradeoffs. Students integrate their ELA skills into MEAs as they are asked to clearly document their thought processes. MEAs follow a problem-based, student-centered approach to learning, where students are encouraged to grapple with the problem while the teacher acts as a facilitator. To learn more about MEA’s visit: https://www.cpalms.org/cpalms/mea.aspx.
MFAS Formative Assessments
Students are asked to add multidigit decimal numbers and are assessed for both accuracy and fluency.
Students are asked to divide multidigit decimal numbers and are assessed for both accuracy and fluency.
Students are asked to multiply multidigit decimal numbers and are assessed for both accuracy and fluency.
Students are asked to solve a word problem involving division of a whole number by a decimal using a model or drawing, a strategy based on place value, the relationship between multiplication and division, or properties of operations.
Students are asked to subtract multidigit decimal numbers and are assessed for both accuracy and fluency.
Student Resources
Problem-Solving Tasks
The purpose of this task is for students to solve problems involving decimals in a context involving a concept that supports financial literacy, namely inflation. Inflation is a sustained increase in the average price level. In this task, students are asked to compare the buying power of $20 in 1987 and 2012, at least with respect to movie tickets.
Type: Problem-Solving Task
Given the fact 13 x 17 = 221, students are asked to reason about and explain the decimal placement in multiplication and division problems where some of the numbers involved have been changed by powers of ten.
Type: Problem-Solving Task
Students are asked to solve problems from context by using multiplication or division of decimals.
Type: Problem-Solving Task
Students are asked to add or subtract decimals to solve problems in context.
Type: Problem-Solving Task
Tutorials
This Khan Academy tutorial video explains patterns in the placement of the decimal point, when a decimal is multiplied by a power of 10. Exponents are NOT discussed.
Type: Tutorial
This Khan Academy tutorial video presents the methodology of understanding and using patterns in the number of zeros of products that have a factor that is a power of 10. While the standard does not mention exponents, the place value understanding of multiplying or dividing by powers of ten will help students understand multiplying and dividing by decimals.
Type: Tutorial
This video demonstrates dividing two numbers that are decimals.
Type: Tutorial
Parent Resources
Problem-Solving Tasks
The purpose of this task is for students to solve problems involving decimals in a context involving a concept that supports financial literacy, namely inflation. Inflation is a sustained increase in the average price level. In this task, students are asked to compare the buying power of $20 in 1987 and 2012, at least with respect to movie tickets.
Type: Problem-Solving Task
Given the fact 13 x 17 = 221, students are asked to reason about and explain the decimal placement in multiplication and division problems where some of the numbers involved have been changed by powers of ten.
Type: Problem-Solving Task
There are two aspects to fluency with division of multi-digit numbers: knowing when it should be applied, and knowing how to compute it. While this task is very straightforward, it represents the kind of problem that sixth graders should be able to recognize and solve relatively quickly. Easily recognizing contexts that require division is a necessary conceptual prerequisite to more complex modeling problems that students will be asked to solve later in middle and high school.
This task also has a natural carryover to work with ratios and rates, so students should also be building connections between these kinds of division problems and finding unit rates.
Type: Problem-Solving Task
Students are asked to solve problems from context by using multiplication or division of decimals.
Type: Problem-Solving Task
Students are asked to add or subtract decimals to solve problems in context.
Type: Problem-Solving Task









