Related Benchmarks
Related Access Points
Access Points
Given the x-intercepts and another point on the graph of a quadratic function, select the equation for the function.
Related Resources
Formative Assessments
Lesson Plans
Original Student Tutorials
Perspectives Video: Experts
Perspectives Video: Professional/Enthusiasts
Perspectives Video: Teaching Ideas
Problem-Solving Tasks
Tutorials
Student Resources
Original Student Tutorials
Learn about different formats of quadratic equations and their graphs with experiments involving launching and shooting of balls in this interactive tutorial.
This is part 2 of a two-part series: Click HERE to open part 1.
Type: Original Student Tutorial
Join us as we watch ball games and explore how the height of a ball bounce over time is represented by quadratic functions, which provides opportunities to interpret key features of the function in this interactive tutorial.
This is part 1 of a two-part series: Click HERE to open part 2.
Type: Original Student Tutorial
Learn the process of completing the square of a quadratic function to find the maximum or minimum to discover how high a dolphin jumped in this interactive tutorial.
This is part 2 of a 2 part series. Click HERE to open part 1.
Type: Original Student Tutorial
Learn the process of completing the square of a quadratic function to find the maximum or minimum to discover how high a dolphin jumped in this interactive tutorial.
This is part 1 of a 2 part series. Click HERE to open Part 2.
Type: Original Student Tutorial
Learn how to solve rational linear and quadratic equations using cross multiplication in this interactive tutorial.
Type: Original Student Tutorial
Learn to find the zeros of a quadratic function and interpret their meaning in real-world contexts with this interactive tutorial.
Type: Original Student Tutorial
Learn to complete the square of a quadratic expression and identify the maximum or minimum value of the quadratic function it defines. In this interactive tutorial, you'll also interpret the meaning of the maximum and minimum of a quadratic function in a real world context.
Type: Original Student Tutorial
Follow as we discover key features of a quadratic equation written in vertex form in this interactive tutorial.
Type: Original Student Tutorial
Perspectives Video: Experts
<p>Jump to it and learn more about how quadratic equations are used in robot navigation problem solving!</p>
Type: Perspectives Video: Expert
<p>It's important to stay inside the lines of your project constraints to finish in time and under budget. This NASA systems engineer explains how constraints can actually promote creativity and help him solve problems!</p>
Type: Perspectives Video: Expert
Perspectives Video: Professional/Enthusiast
<p>Get in gear with robotics as this engineer explains how quadratic equations are used in programming robotic navigation.</p>
Type: Perspectives Video: Professional/Enthusiast
Problem-Solving Tasks
This problem asks students to model phenomena on the surface of the earth by examining the visibility of the lamp in a lighthouse from a boat.
Type: Problem-Solving Task
This problem solving task challenges students to find the linear, exponential and quadratic functions based on two points.
Type: Problem-Solving Task
Tutorials
This tutorial will help the students to identify the vertex of a parabola from the equation, and then graph the parabola.
Type: Tutorial
This tutorial helps the learners to graph the equation of a quadratic function using the coordinates of the vertex of a parabola and its x- intercepts.
Type: Tutorial
Parent Resources
Perspectives Video: Expert
<p>It's important to stay inside the lines of your project constraints to finish in time and under budget. This NASA systems engineer explains how constraints can actually promote creativity and help him solve problems!</p>
Type: Perspectives Video: Expert
Perspectives Video: Professional/Enthusiast
<p>Get in gear with robotics as this engineer explains how quadratic equations are used in programming robotic navigation.</p>
Type: Perspectives Video: Professional/Enthusiast
Problem-Solving Tasks
This problem asks students to model phenomena on the surface of the earth by examining the visibility of the lamp in a lighthouse from a boat.
Type: Problem-Solving Task
This problem solving task challenges students to find the linear, exponential and quadratic functions based on two points.
Type: Problem-Solving Task






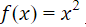 .
.
