MAFS.912.C.1.9Archived Standard

loading spinner
Export Print
Understand continuity in terms of limits.

Remarks

Example 1: Show that  f(x)=3x + 1 is continuous at x = 2 by finding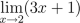  and comparing it with f(2).

Example 2: Given that the limg(x) as x approaches to 5 exists, is the statement “g(x) is continuous at x=5” necessarily true? Provide example functions to support your conclusion. 
General Information
Subject Area: Mathematics
Grade: 912
Domain-Subdomain: Calculus
Cluster: Level 3: Strategic Thinking & Complex Reasoning
Cluster: Limits and Continuity - Develop an understanding of the concept of limit by estimating limits graphically and numerically and evaluating limits analytically. Extend the idea of a limit to one-sided limits and limits at infinity. Use limits to define and understand the concept of continuity, decide whether a function is continuous at a point, and find types of discontinuities. Understand and apply continuity theorems.
Date Adopted or Revised: 02/14
Content Complexity Rating: Level 3: Strategic Thinking & Complex Reasoning - More Information
Date of Last Rating: 02/14
Status: State Board Approved - Archived

Related Courses

This benchmark is part of these courses.
1202340: Precalculus Honors (Specifically in versions: 2014 - 2015, 2015 - 2022, 2022 - 2024, 2024 and beyond (current))

Related Access Points

Alternate version of this benchmark for students with significant cognitive disabilities.

Related Resources

Vetted resources educators can use to teach the concepts and skills in this benchmark.

Lesson Plan

Leap Frog Review Game:

In this lesson students will demonstrate their knowledge of limits, graphing, and exact trig limits evaluated using substitution. The students will play a game in which they evaluate their knowledge of problems in the unit while it serves as a formative assessment for the teacher. The students receive immediate feedback on their work while the teacher works the problems, correcting errors or misconceptions. This lesson gives the student a power review of the concepts in the unit because the timing is determined by the teacher. All students are engaged and focused while playing this game. Giving students access to the PowerPoint of the game after the lesson provides a good study tool for the students.

Type: Lesson Plan

Tutorials

Limits to Define Continuity:

We will use limits to define continuity.

Type: Tutorial

Determining which limit statements are true:

This video demonstrates how to determine which limit statements are true.

Type: Tutorial

Student Resources

Vetted resources students can use to learn the concepts and skills in this benchmark.

Tutorials

Limits to Define Continuity:

We will use limits to define continuity.

Type: Tutorial

Determining which limit statements are true:

This video demonstrates how to determine which limit statements are true.

Type: Tutorial

Parent Resources

Vetted resources caregivers can use to help students learn the concepts and skills in this benchmark.