Benchmark Instructional Guide
Connecting Benchmarks/Horizontal Alignment
Terms from the K-12 Glossary
- Conditional relative Frequency
- Event
- Experimental probability
- Frequency table
- Joint frequency
- Sample space
- Theoretical probability
Vertical Alignment
Previous Benchmarks
Next Benchmarks
Purpose and Instructional Strategies
In middle grades, students began working with theoretical probabilities and comparing them to experimental probability. In Mathematics for College Liberal Arts, students determine if two events are independent of each other.
- Independence in this sense means that knowing whether one event occurred does not change the probability of the other event occurring. For this benchmark, students determine independence using conditional probabilities. Two events, A and B, are independent if P(A | B) = P(A) and P(B | A) = P(B).
- Students also use the product of probabilities to check independence (MA.912.DP.4.2).
- If P(A ∩ B) = P(A) × P(B), then the events are independent.
- Be sure to distinguish independence from mutually exclusive events.
- In mutually exclusive events (A ∩ B) = 0. This means that the two events cannot occur at the same time.
- Instruction includes the understanding that P(A | B) ≠ P(B | A) unless P(A) = P(B).
- When we check for independence in real-world data sets, it's rare to get perfectly equal probabilities. We often assume that events are independent and test that assumption on sample data. If the probabilities are significantly different, then we conclude the events are not independent.
Common Misconceptions or Errors
- Students may confuse what it means to be dependent and independent.
- Students may confuse independence with mutually exclusive events.
- Students may have difficulty recalling how to convert fractions, decimals and percentages.
- Students may think the symbol used for conditional probability is a slash that would be used to represent division and simply divide the probability of A by the probability of B.
- Students may get confused as to which event probability should be the denominator.
- Students may get confused when working with a two-way table that they need to restrict
their answer to a certain section that is from the “given” conditional piece.
- For example, when given the condition of male they are only looking in the row or column containing males to get the total.
- Students who have difficulty with the terminology and notation will also have difficulty in understanding what is being asked by the questions.
Instructional Tasks
Instructional Task 1- The table below displays the results of a survey of eating preferences.
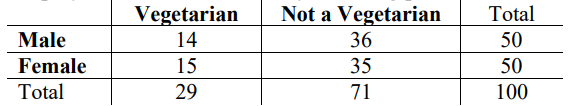
- Part A. Name two events that can be represented by the two-way table.
- Part B. Are the two events in Part A independent events? Explain why or why not using conditional probability.
Instructional Items
Instructional Item 1- There are 16 cards in a deck of cards. There are 4 red cards, 4 green cards, 4 blue cards and 4 yellow cards. The cards in each color are numbered 1 to 4. Are picking the number 4 and picking a yellow card independent events? Why or why not?
*The strategies, tasks and items included in the B1G-M are examples and should not be considered comprehensive.
Related Courses
Related Access Points
Related Resources
Lesson Plans
Perspectives Video: Expert
Problem-Solving Tasks
Student Resources
Problem-Solving Tasks
This problem solving task challenges students to determine if two weather events are independent, and use that conclusion to find the probability of having similar weather events under certain conditions.
Type: Problem-Solving Task
Students answer questions about the probabilities of independent and dependent events.
Type: Problem-Solving Task
This problem solving task lets students explore the concept of independence of events.
Type: Problem-Solving Task
This task lets students explore the concepts of probability as a fraction of outcomes using two-way tables.
Type: Problem-Solving Task
Parent Resources
Problem-Solving Tasks
This problem solving task challenges students to determine if two weather events are independent, and use that conclusion to find the probability of having similar weather events under certain conditions.
Type: Problem-Solving Task
Students answer questions about the probabilities of independent and dependent events.
Type: Problem-Solving Task
This problem solving task lets students explore the concept of independence of events.
Type: Problem-Solving Task
This task lets students explore the concepts of probability as a fraction of outcomes using two-way tables.
Type: Problem-Solving Task









