Clarifications
Clarification 1: Instruction includes using the Pythagorean Theorem and completing the square.Clarification 2: Within the Geometry course, key features are limited to the radius, diameter and the center.
Benchmark Instructional Guide
Connecting Benchmarks/Horizontal Alignment
Terms from the K-12 Glossary
- Circle
- Diameter
- Radius
Vertical Alignment
Previous Benchmarks
Next Benchmarks
Purpose and Instructional Strategies
In grade 8, students solved problems involving the Pythagorean Theorem. In Algebra 1, students rearranged formulas to highlight a quantity of interest, worked with quadratic equations and functions and performed single transformations on functions. In Geometry, this background provides a solid foundation for writing the equation of a circle to represent a variety of contexts, based on key features of the circle. In later courses, students will expand these skills to write equations for other circles, parabolas, hyperbolas and ellipses based on their key features.- Instruction includes students deriving the equation of a circle centered at the origin and at a point (, ). Students should make the connection to the Pythagorean Theorem by drawing right triangles from the center of the circle. Students should make the connection between the equations centered at the origin and at a point (, ); realizing that if the point (, ) is the origin then the equation can be written as either 2 + 2 = 2 or as
( − 0)2 + ( − 0)2 = 2.
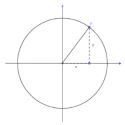
- For example, given a circle centered at the origin on the coordinate plane and
have students select a point (, ) on the circle. Students should be able to see that the distance from to the center is the radius of the circle. Students can then draw a right triangle, like the one shown below, that includes the center and as two of its vertices. Students can use their knowledge of the Pythagorean Theorem to develop the equation 2 + 2 = 2, showing the relationship between , and the radius, . Students should be able to realize that this equation is valid for every point P(, ) on the circle and is not valid for any point not on the circle.
- For example, given a circle centered at (, ) on the coordinate plane and have students select a point (, ) on the circle. Students should be able to see that the distance from to the center is the radius of the circle. Students can then draw a right triangle, like the one shown below, that includes the center and as two of its vertices. Students can use their knowledge of the Pythagorean Theorem to develop the equation ( − )2 + ( − )2 = 2, showing the relationship between , , , and the radius, . Students should be able to realize that this equation is valid for every point (, ) on the circle and is not valid for any point not on the circle.
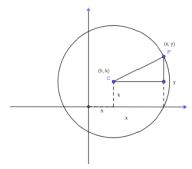
- For example, given a circle centered at the origin on the coordinate plane and
have students select a point (, ) on the circle. Students should be able to see that the distance from to the center is the radius of the circle. Students can then draw a right triangle, like the one shown below, that includes the center and as two of its vertices. Students can use their knowledge of the Pythagorean Theorem to develop the equation 2 + 2 = 2, showing the relationship between , and the radius, . Students should be able to realize that this equation is valid for every point P(, ) on the circle and is not valid for any point not on the circle.
- Instruction includes the connection to transformation of functions (as was done in Algebra 1) when developing an understanding of the relationship between the equations 2 + 2 = 2 and ( − )2 + ( − )2 = 2.
- Students should have experience working with the equation of a circle in center-radius form, ( − )2 + ( − )2 = 2, and in general form, 2 + 2 + + + = 0 where and are the same real, non-zero number. Students may need to convert from one form to another, particularly from the general form to the center-radius form. Instruction includes discussing how this conversion can be done and the benefits of each form of the equation of a circle.
- Instruction includes exploring the pattern of completing the square for the general form and the way the center and the radius can be obtained from this procedure. Exploration includes cases where the radius may result in a complex number; noting that the equation does not give a circle when this happens.
- For example, given the equation 2 + 2 + + + = 0 when = 1 and = 1, students can rewrite this as 2 + + 2 + = −. Then students can complete the square for both the -part of the equation and the -part of the
equation. Students should obtain the equation ( + )2 + (
y D 2 E C 2 E 2 C 2 D 2 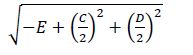 .
.
- For example, given the equation 2 + 2 + + + = 0 when = 1 and = 1, students can rewrite this as 2 + + 2 + = −. Then students can complete the square for both the -part of the equation and the -part of the
equation. Students should obtain the equation ( + )2 + (
- Problem types include cases where
A B - For enrichment of this benchmark, instruction includes the connection to finding the equation of the circumscribed circle about a triangle whose vertices are given on the coordinate plane. (MTR.5.1)
Common Misconceptions or Errors
- Students may confuse the signs of (
h k - For example, given the equation (
x y r
- For example, given the equation (
Instructional Tasks
Instructional Task 1 (MTR.4.1)- A circle on the coordinate plane is given. Segments
C B E D A C D B E 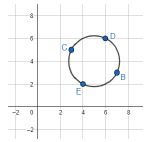
- Part A. Determine the center of the circle. Explain your method.
- Part B. Find the length of the radius of circle
A - Part C. Write the equation of the circle and check that the points
B C D E
Instructional Task 2 (MTR.2.1, MTR.5.1)
- Point (
x y h k 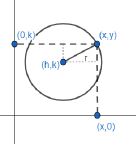
- Part A. The horizontal distance between point (
x y h k x y h k - Part B. Using Pythagorean Theorem, write an equation for the radius in terms of (
x y h k - Part C. Because (
x y h k
- Part A. The horizontal distance between point (
Instructional Task 3 (MTR.7.1)
- A school’s campus is designed in the shape of a circle. The architect would like to place the cafeteria equidistant from the Freshman building and from the Senior building. On a coordinate plane, the Freshman building is located at the point (431, 219) and the Senior building is located at the point (0,0), where the coordinates are given in feet. Assume that the endpoints of the diameter of circle are the Freshman building and the Senior building and that the cafeteria is on the line connecting the two buildings.
- Part A. Determine the location of the cafeteria.
- Part B. How far is it from the cafeteria to the Freshman building?
- Part C. Write an equation that represents the boundary of the circular campus.
- Part D. If the campus were to have a circular fence along its boundary, what is the total length of the fence, in feet?
Instructional Items
Instructional Item 1- Given the equation
x x y y E E
Instructional Item 2
- What is the equation of a circle centered at (−1, 2), with a diameter of 2 units?
Instructional Item 3
- What is the equation of the circle centered at (−2, −5) and passing through (5, 0)?
Related Courses
Related Access Points
Related Resources
Formative Assessments
Lesson Plans
Original Student Tutorials
Problem-Solving Tasks
MFAS Formative Assessments
Students are asked to find the center and radius of a circle given its equation in general form.
Students are asked to find the center and radius of a circle given its equation in general form.
Students are given the coordinates of the center, (h, k), and the radius, r, of a circle and are asked to derive the equation of the circle using the Pythagorean Theorem.
Students are given the coordinates of the center and the radius of a circle and are asked to derive the equation of the circle using the Pythagorean Theorem.
Original Student Tutorials Mathematics - Grades 9-12
Learn how to write the equation of a circle using Pythagorean Theorem given its center and radius using step-by-step instructions in this interactive tutorial.
Find the location and coverage area of cell towers to determine the center and radius of a circle given its equation, using a strategy completing the square in this interactive tutorial.
Student Resources
Original Student Tutorials
Find the location and coverage area of cell towers to determine the center and radius of a circle given its equation, using a strategy completing the square in this interactive tutorial.
Type: Original Student Tutorial
Learn how to write the equation of a circle using Pythagorean Theorem given its center and radius using step-by-step instructions in this interactive tutorial.
Type: Original Student Tutorial
Problem-Solving Tasks
The purpose of this task is to lead students through an algebraic approach to a well-known result from classical geometry, namely, that a point X is on the circle of diameter AB whenever angle AXB is a right angle.
Type: Problem-Solving Task
This problem solving task challenges students to use ideas about linear functions in order to determine when certain angles are right angles.
Type: Problem-Solving Task
Parent Resources
Problem-Solving Tasks
The purpose of this task is to lead students through an algebraic approach to a well-known result from classical geometry, namely, that a point X is on the circle of diameter AB whenever angle AXB is a right angle.
Type: Problem-Solving Task
This problem solving task challenges students to use ideas about linear functions in order to determine when certain angles are right angles.
Type: Problem-Solving Task









