MA.5.AR.2.4

Benchmark Instructional Guide

Connecting Benchmarks/Horizontal Alignment

• MA.5.NSO.2.1
• MA.5.NSO.2.2 
• MA.5.AR.1.1
• MA.5.AR.3.1

Terms from the K-12 Glossary

• Equal Sign 
• Equation 
• Expression 
• Whole Number

Vertical Alignment

Previous Benchmarks

• MA.4.AR.2.2

 

Next Benchmarks

• MA.6.AR.1.4 
• MA.6.AR.2

Purpose and Instructional Strategies

• Instruction should focus on helping students translate mathematical and real-world contexts to equations. Instructional emphasis should be placed on students’ comprehension of the contexts to then translate to equations more easily. An instructional strategy that helps students translate from context to symbolic equations is to first present contexts with some or all their numerical information omitted. In a mathematical context, this may look like showing a data display with some numerical information covered. In a real-world context, this may look like a word problem with quantities covered. This allows students to comprehend what the problem is trying to find and allows students to think deeper about what operations will be required to do so. It can also help students estimate reasonable solution ranges. Once students can predict an equation (or equations) to solve the problem, then the teacher can reveal all numerical information and allow students to solve (MTR.5.1). 
• In each context, students may provide many examples of equations that can be used to solve. During instruction, teachers should have students compare their equations and evaluate whether they can be used to solve (MTR.4.1). 
• During instruction, students should justify how their equations match the mathematical and real-world contexts through checking solutions. Students should substitute their solution for their letter symbol and use the order of operations to check that it makes the equation true.

Common Misconceptions or Errors

• When students have trouble comprehending contexts, they tend to just grab numbers from a given context and begin computing without justifying their arguments. Emphasis of instruction should be on the comprehension of problems through classroom discussion, sharing strategies, estimating reasonable solutions, and justifying equations and solutions.

Strategies to Support Tiered Instruction

• Instruction focuses on the comprehension of problems through classroom discussion, sharing strategies, estimating reasonable solutions, and justifying equations and solutions. 
• Instruction includes opportunities to connect real-world situations to write equations using any of the four operations to determine an unknown whole number with the unknown in any position. Students apply the order of operations to solve for the unknown. The teacher emphasizes the inverse relationships between addition and subtraction, and multiplication and division as applicable in order to help students solve for the unknown, while reinforcing conceptual understanding by having students use drawings, models and equations to solve real world problems.
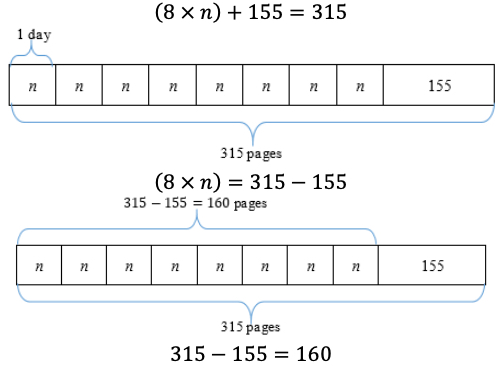
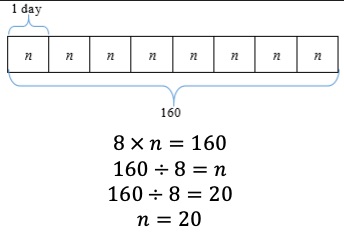
  • For example, the teacher displays and reads the following problem aloud: “Renaldo read the same number of pages of his book each day for 8 days. He needs to read a total of 315 pages, and still needs to read 155 pages to meet his goal. How many pages did he read on each of the 8 days so far?” Students are provided manipulatives, such as counters or base-ten blocks, to model the problem or to use a drawing, such as a bar model, to solve and to write an equation. Through prompting and questioning, students explain their models, justify their solutions, and check their solution, repeating with multiple examples of real-world problems. 

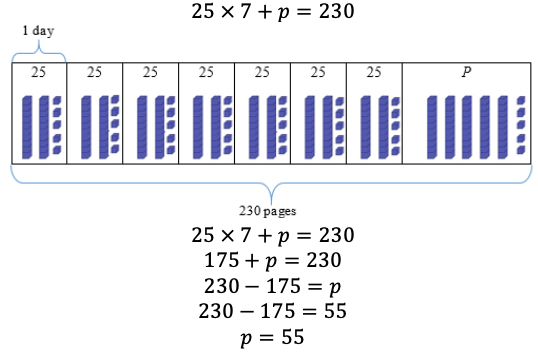
• For example, Elijah reads 25 pages of a novel per day for 7 days. The entire novel is 230 pages, how many pages does he have left to read? Students are provided manipulatives, such as counters or base-ten blocks, to model the problem or to use a drawing, such as a bar model, to solve and to write an equation. Through prompting and questioning, students explain their models, justify their solutions, and check their solution, repeating with multiple examples of real-world problems.

Instructional Tasks

Instructional Task 1 (MTR.7.1) 

• Part A. Write an equation that can be used to solve. Use a letter to represent the unknown number. 
• Part B. What is the greatest number of cookies each of Dr. Ocasio’s students can receive? 
• Part C. Prove that your answer is correct by showing how your equation is true. Instructional Items

Instructional Items

Instructional Item 1 

• a. 96–21–12=c 
• b. 96−(21×c)=12 
• c. 12+c=96−21 
• d. 21×c+12=96 

Related Courses

Related Access Points

Related Resources

Student Resources

Parent Resources