Examples
Ken is filling his garden containers with a cup that holds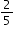 pounds of soil. If he uses 8 cups to fill his garden containers, how many pounds of soil did Ken use?
pounds of soil. If he uses 8 cups to fill his garden containers, how many pounds of soil did Ken use?
Clarifications
Clarification 1: Problems include creating real-world situations based on an equation or representing a real-world problem with a visual model or equation.Clarification 2: Fractions within problems must reference the same whole.
Clarification 3: Within this benchmark, the expectation is not to simplify or use lowest terms.
Clarification 4: Fractions limited to fractions less than one with denominators of 2, 3, 4, 5, 6, 8, 10, 12, 16 and 100.
Benchmark Instructional Guide
Connecting Benchmarks/Horizontal Alignment
Terms from the K-12 Glossary
- Equation
- Expression
- Whole Number
Vertical Alignment
Previous Benchmarks
Next Benchmarks
Purpose and Instructional Strategies
The purpose of this benchmark is to complement the instruction of MA.4.FR.2.4 with real-world context.- Instruction should refer back to the two types of problems described in MA.4.FR.2.4 (whole number times a fraction and fraction times a whole number), and give students opportunities to work with real-world examples of both types.
- Instruction should help students bring their understanding of working with units involving whole numbers (1 cup, 2 miles, etc.) to working with units involving fractions ( cup, miles, etc.)
- During instruction it is acceptable to have students work with problems where fractional parts represent more than 1 whole (e.g., a cake recipe calls for cup of water and oz of vanilla extract, and the baker wants to double the recipe).
- Instruction may include having students create real-world situations that can be modeled by a given expression like × 10 (I have completed of my 10-mile run).
- During instruction, models and explanations should relate fraction multiplication to equal groups. This will activate prior knowledge and relate what students know to whole-number multiplication.
- For example, teachers can help students make connections to multiplication from grade 3 by referring to an expression like 4 × as “ four groups of 3 fifths,” that 5 is, “ four groups that each contain 3 items and each item is one fifth” (MTR.2.1, MTR.5.1).
- Example: have table/bar to show that there are 4 groups of .
- Exploring patterns of what happens to the numerator when a whole number is multiplied by a fraction will help students make sense of multiplying fractions by fractions in grade 5 (MTR.2.1). When multiplying whole numbers by mixed numbers, students can use the distributive property or write the mixed number as a fraction greater than one. During instruction, students should compare both strategies (MTR.6.1). Using the distributive property to multiply a whole number by a mixed number could look like this.
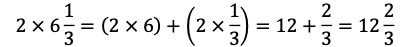
- In the example, 2 groups of 6 was written as “ the sum of 2 groups of 6 and 2 groups of 1 third.” The products of 12 and 2 thirds are added to show the product of 12 .
Common Misconceptions or Errors
- Students may not understand that fractions are numbers (just as whole numbers are numbers) and this misconception may at first be reinforced by the fact that the phrase “ group size” works well with whole numbers, but not so well with fractions; therefore, special attention should be given with many different real-world examples.
- Students may not understand what a fractional portion represents within the context of a real-world situation.
Strategies to Support Tiered Instruction
- Instruction includes opportunities to engage in teacher-directed practice using visual representations to solve real-world problems involving the multiplication of a fraction by a whole number. Students are directed on how to use models or equations based on real-world situations. Through questioning, the teacher guides students to explain what each fractional portion represents in the problems used during instruction and practice.
- For example, the teacher displays and reads aloud the following problem: “ Angelica walks her dog of a mile every day. How far does she walk her dog after 7 days?” Using models, students solve the problem with explicit instruction and guided questioning. Students explain how to use models to solve this addition by guiding students to represent this problem as + + + + + + and that 7 × is the same as seven groups of four fifths. The teacher guides students to create an equation to represent the problem, repeating multiple real-world problems that involve the multiplication of a whole number by a fraction or a fraction by a whole number.
- Instruction includes opportunities for students to use hands-on models and manipulatives to solve real-world problems involving the multiplication of a whole number by a fraction. Students are guided in explaining how each model represents the real-world situation. Explicitly direct students on how to use models or equations based on real-world situations. Through questioning, students explain what each fractional portion represents in the problems used during instruction and practice.
- For example, the teacher displays and reads aloud the following problem: “ Ramon is baking cupcakes for his cousin’s birthday party. The recipe calls for 2 cups of sugar. He only needs to make as many cupcakes as the recipe call for. How many cups of sugar will Ramon need to use?” It may be useful to provide students with paper models that represent the cups of sugar. Students should cut the models into thirds to determine how much sugar will be needed for the recipe.
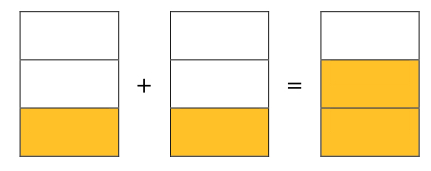
Instructional Tasks
Instructional Task 1 (MTR.2.1)
Lorelei is having a dessert party and wants to determine how much sugar she will need. For the party, she will make 4 batches of chocolate chip cookies and 8 vanilla smoothies. 1 batch of chocolate chip cookies requires cup of sugar and 1 vanilla smoothie requires cup of sugar. How much total sugar will she need for her dessert party? Draw a model to explain your thinking.
Instructional Items
Instructional Item 1
A butcher has 10 pounds of meat and sells of it in one day. How many pounds does the butcher sell?*The strategies, tasks and items included in the B1G-M are examples and should not be considered comprehensive.
Related Courses
Related Access Points
Related Resources
Lesson Plans
Original Student Tutorial
Problem-Solving Task
STEM Lessons - Model Eliciting Activity
In this Model Eliciting Activity, MEA, students will rank four promotional deals that a travel agency is running. Before they make their decision, the students must find the discounted price by multiplying a whole number by a fraction and convert the duration of the trip to the same unit.
Model Eliciting Activities, MEAs, are open-ended, interdisciplinary problem-solving activities that are meant to reveal students’ thinking about the concepts embedded in realistic situations. MEAs resemble engineering problems and encourage students to create solutions in the form of mathematical and scientific models. Students work in teams to apply their knowledge of science and mathematics to solve an open-ended problem, while considering constraints and tradeoffs. Students integrate their ELA skills into MEAs as they are asked to clearly document their thought process. MEAs follow a problem-based, student centered approach to learning, where students are encouraged to grapple with the problem while the teacher acts as a facilitator. To learn more about MEA’s visit: https://www.cpalms.org/cpalms/mea.aspx
Original Student Tutorials Mathematics - Grades K-5
Help April calculate area and missing measurements for items in her perfect dream home in this interactive tutorial.
This is the first in a three-part series. Click below to open the other tutorials in this series
Student Resources
Original Student Tutorial
Help April calculate area and missing measurements for items in her perfect dream home in this interactive tutorial.
This is the first in a three-part series. Click below to open the other tutorials in this series
Type: Original Student Tutorial
Problem-Solving Task
This task provides a familiar context allowing students to visualize multiplication of a fraction by a whole number. This task could form part of a very rich activity which includes studying soda can labels.
Type: Problem-Solving Task
Parent Resources
Problem-Solving Task
This task provides a familiar context allowing students to visualize multiplication of a fraction by a whole number. This task could form part of a very rich activity which includes studying soda can labels.
Type: Problem-Solving Task









