Word (.docx)
Acrobat (.pdf)
Examples
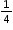can be represented as 
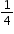of a pie (parts of a shape), as 1 out of 4 trees (parts of a set) or as 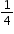on the number line.
Clarifications
Clarification 1: This benchmark emphasizes conceptual understanding through the use of manipulatives or visual models.Clarification 2: Instruction focuses on representing a unit fraction as part of a whole, part of a set, a point on a number line, a visual model or in fractional notation.
Clarification 3: Denominators are limited to 2, 3, 4, 5, 6, 8, 10 and 12.
Benchmark Instructional Guide
Connecting Benchmarks/Horizontal Alignment
Terms from the K-12 Glossary
- Number line
Vertical Alignment
Previous Benchmarks
Next Benchmarks
Purpose and Instructional Strategies
The purpose of this benchmark is for students to understand that unit fractions are the foundation for all fractions. Second, the purpose is for students to understand that fractions are numbers. This benchmark continues instruction of fractions from Grade 2, where students partitioned circles and rectangles into two, three or four equal-sized parts (MA.2.FR.1.1 and MA.2.FR.1.2).- To activate prior knowledge in Grade 3, instruction should:
- Relate how unit fractions build fractions to how whole-number units build whole numbers, and
- Show models with non-equal parts as non-examples (MTR.2.1).
- Unit fractions are defined as one part when a whole is partitioned in any number of equal parts. It is in this benchmark that students conclude that the greater a unit fraction’s denominator, the greater its number of parts.
- Instruction should demonstrate how to represent unit fractions using manipulatives (e.g., fraction strips, circles, relationship rods), visual area models (e.g., partitioned shapes), on a number line, and as 1 object in a set of objects (MTR.2.1, MTR.5.1).
- Denominators are limited in Grade 3 to facilitate the visualizing and reasoning required while students plot, compare and identify equivalence in fractions.
Common Misconceptions or Errors
- Students can misconceive the difference between the meaning of numerators and denominators in fractions. For this reason, it is important for teachers and students to represent unit fractions in multiple ways to understand how they relate to a whole. Representations can be modeled together (e.g., fraction strips side-by-side with number lines, or relationship rods side-by-side with number lines) to help build student understanding.
- Students can misconceive that the smaller the denominator, the smaller the piece, or the larger the denominator, the larger the piece. This is due to thinking and reasoning where students worked with whole numbers (the smaller a number, the less it is, or the larger a number, the more it is). To correct this misconception, have students utilize different models, such as fraction bars and number lines, which would provide students opportunities to compare unit fractions and to reason about their sizes.
- Students can misconceive that all shapes can be partitioned the same way. To assist with this misconception, have students practice with presenting shapes other than circles, squares or rectangles to prevent students from overgeneralizing that all shapes can be divided the same way.
Strategies to Support Tiered Instruction
- Teacher represents unit fractions in multiple ways to show understanding of how they relate to a whole. Representations are modeled together (e.g., fraction strips side-by-side with number lines, or relationship rods side-by-side with number lines) to help build understanding.
- Instruction includes partitioning shapes into different denominators.
- For example, students compare what they notice about partitioning a rectangle into halves versus fourths. Teacher asks students, “What do you notice about the pieces? How can we write what one piece of the rectangle is worth with a fraction?” Instruction includes the vocabulary of numerator and denominator.
- Instruction includes shapes other than circles and rectangles. Items like pattern blocks allow students to partition shapes like hexagons and rhombi into equal-sized pieces. This prevents students from over-generalizing that all shapes can be divided the same way.
- Instruction includes folding and/or cutting premade shapes into different amounts. Students benefit from beginning with halves and fourths, folding the paper in half, and then folding those halves into halves to make fourths.
- For example, the teacher asks students, “What do you notice about the shapes? About the size? We now have 4 pieces, Do we have more than we did before?&rdquo The conversation includes the size of the pieces and how that relates to the denominator.
Instructional Tasks
Instructional Task 1Mrs. Asbel wants to paint a mural on her classroom wall. She creates six equal sections in her mural.
- Part A. Use manipulatives to create a visual model of the mural.
- Part B. What fraction represents each section of the mural?
Instructional Task 2
Madison wants to divide a pan of brownies into 12 equal pieces.
- Part A. Show three different ways that Madison can divide her pan of brownies into 12 equal pieces.
- Part B. One piece of brownie would represent what fraction?
Instructional Task 3
Students at River Valley Elementary School are building a vegetable garden. Here is a picture of the garden so far:
- Part A. Show how you would divide the vegetable garden into eight equal parts.
- Part B. Explain how you know that the parts you made in Part A are equal.
- Part C. Shade in one part of the vegetable garden. What fraction does this equal?
Instructional Task 4
Terry wants to show the unit fraction using an area model, a number line, and as a set.- Part A. Into how many equal parts should Terry partition his area model? How many of those parts should be shaded? Explain in words.
- Part B. Represent using the number line below.
- Part C. Draw a model that represents of a set of juice boxes.
Instructional Items
Instructional Item 1
Each model shown has been shaded to represent a fraction. Which model shows shaded?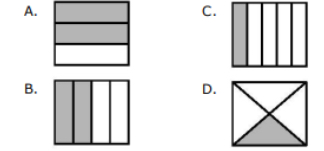
Instructional Item 2
Write the fraction that is shown on the number line.
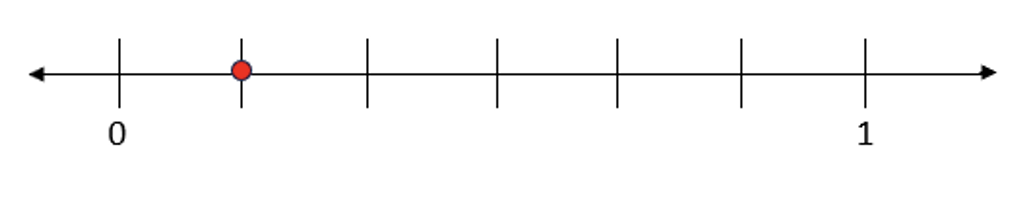
Instructional Item 3
Select all of the following that show 1/8.
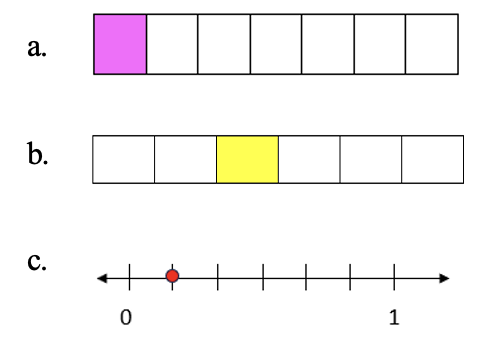
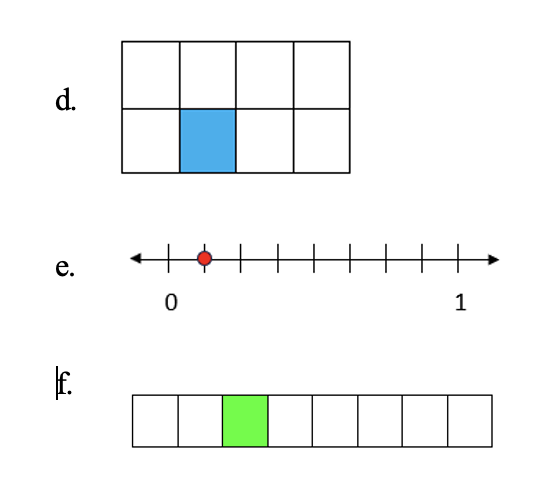
Instructional Item 4
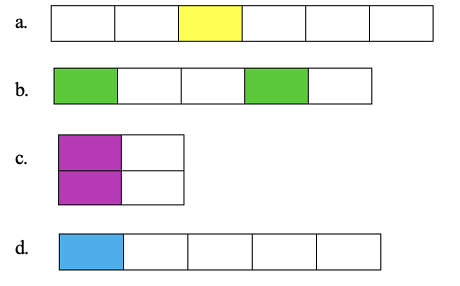
Jackson is helping hang his school flag outside the building. The flag has five equal-sized sections on it, each in a different color. Which fraction model represents one section of the school flag?
*The strategies, tasks and items included in the B1G-M are examples and should not be considered comprehensive.
Related Courses
Related Access Points
Explore unit fractions in the form as the quantity formed by one part when a whole is partitioned into n equal parts. Denominators are limited to 2, 3 and 4.
Related Resources
Educational Game
Formative Assessments
Image/Photograph
Lesson Plans
Original Student Tutorials
Perspectives Video: Teaching Ideas
Problem-Solving Tasks
Virtual Manipulatives
MFAS Formative Assessments
Students partition a rectangle into four equal parts and describe each part using a fraction.
Students partition an irregular hexagon into two equal parts and describe each part using a unit fraction.
Students divide figures into two parts, each having the same area, and write the unit fraction representing each part.
Students divide a hexagon into two, three, and six equal parts and write the unit fraction representing each part.
Students are shown the fraction one fifth and asked to describe what it means.
Students are shown three circles and asked to select the one that correctly shows one third shaded and explain why the other two do not.
Original Student Tutorials Mathematics - Grades K-5
Joey learns about the location of unit fractions on a number line while at camp in this interactive tutorial.
Learn about unit fractions and how to partition number lines to plot unit fractions' locations. Join Nik, Natalia, and their neighborhood friends on a number line fraction finding adventure in this interactive tutorial.
Learn to name or identify fractions, especially unit fractions, and justify the fractional value using an area model in this pizza-themed, interactive tutorial.
Student Resources
Original Student Tutorials
Learn about unit fractions and how to partition number lines to plot unit fractions' locations. Join Nik, Natalia, and their neighborhood friends on a number line fraction finding adventure in this interactive tutorial.
Type: Original Student Tutorial
Joey learns about the location of unit fractions on a number line while at camp in this interactive tutorial.
Type: Original Student Tutorial
Learn to name or identify fractions, especially unit fractions, and justify the fractional value using an area model in this pizza-themed, interactive tutorial.
Type: Original Student Tutorial
Educational Game
This is a fun and interactive game that helps students practice ordering rational numbers, including decimals, fractions, and percents. You are planting and harvesting flowers for cash. Allow the bee to pollinate, and you can multiply your crops and cash rewards!
Type: Educational Game
Problem-Solving Tasks
This task continues "Which pictures represent half of a circle?" moving into more complex shapes where geometric arguments about cutting or work using simple equivalences of fractions is required to analyze the picture. In order for students to be successful with this task, they need to understand that area is additive.
Type: Problem-Solving Task
This task presents students with some creative geometric ways to represent the fraction one half. The goal is both to appeal to students' visual intuition while also providing a hands on activity to decide whether or not two areas are equal. In order for students to be successful with this task, they need to understand that area is additive.
Type: Problem-Solving Task
This task is designed to help students focus on the whole that a fraction refers. It provides a context where there are two natural ways to view the coins. While the intent is to deepen a student's understanding of fractions, it does go outside the requirements of the standard.
Type: Problem-Solving Task
The goal of this task is to show that when the whole is not specified, which fraction is being represented is left ambiguous.
Type: Problem-Solving Task
Virtual Manipulatives
This virtual manipulative will help the students to build fractions from shapes and numbers to earn stars in this fraction lab. To challenge the children there are multiple levels, where they can earn lots of stars.
Some of the sample learning goals can be:
- Build equivalent fractions using numbers and pictures.
- Compare fractions using numbers and patterns
- Recognize equivalent simplified and unsimplified fractions
Type: Virtual Manipulative
This virtual manipulative allows individual students to work with fraction relationships. (There is also a link to a two-player version.)
Type: Virtual Manipulative
Parent Resources
Image/Photograph
Illustrations that can be used for teaching and demonstrating fractions. Fractional representations are modeled in wedges of circles ("pieces of pie") and parts of polygons. There are also clipart images of numerical fractions, both proper and improper, from halves to twelfths. Fraction charts and fraction strips found in this collection can be used as manipulatives and are ready to print for classroom use.
Type: Image/Photograph
Problem-Solving Tasks
This task continues "Which pictures represent half of a circle?" moving into more complex shapes where geometric arguments about cutting or work using simple equivalences of fractions is required to analyze the picture. In order for students to be successful with this task, they need to understand that area is additive.
Type: Problem-Solving Task
This task presents students with some creative geometric ways to represent the fraction one half. The goal is both to appeal to students' visual intuition while also providing a hands on activity to decide whether or not two areas are equal. In order for students to be successful with this task, they need to understand that area is additive.
Type: Problem-Solving Task
This task is designed to help students focus on the whole that a fraction refers. It provides a context where there are two natural ways to view the coins. While the intent is to deepen a student's understanding of fractions, it does go outside the requirements of the standard.
Type: Problem-Solving Task
The goal of this task is to show that when the whole is not specified, which fraction is being represented is left ambiguous.
Type: Problem-Solving Task
Virtual Manipulative
This virtual manipulative will help the students to build fractions from shapes and numbers to earn stars in this fraction lab. To challenge the children there are multiple levels, where they can earn lots of stars.
Some of the sample learning goals can be:
- Build equivalent fractions using numbers and pictures.
- Compare fractions using numbers and patterns
- Recognize equivalent simplified and unsimplified fractions
Type: Virtual Manipulative
