General Information
Clusters should not be sorted from Major to Supporting and then taught in that order. To do so would strip the coherence of the mathematical ideas and miss the opportunity to enhance the major work of the grade with the supporting clusters.
Test Item Specifications
- Interpreting diagrams.
- Identifying mathematical knowledge relevant to an argument.
- Linking visual and algebraic representations.
- Producing and evaluating mathematical arguments.
Items may assess theorems and their converses for interior triangle
sum, base angles of isosceles triangles, mid-segment of a triangle,
concurrency of medians, concurrency of angle bisectors, concurrency
of perpendicular bisectors, triangle inequality, and the Hinge
Theorem.
Items may include narrative proofs, flow-chart proofs, two-column
proofs, or informal proofs.
In items that require the student to justify, the student should not be
required to recall from memory the formal name of a theorem.
Neutral
Students will prove theorems about triangles.
Students will use theorems about triangles to solve problems
Items may be set in a real-world or mathematical context
Items may require the student to give statements and/or
justifications to complete formal and informal proofs.
Items may require the student to justify a conclusion from a
construction.
Sample Test Items (1)
| Test Item # | Question | Difficulty | Type |
| Sample Item 1 | 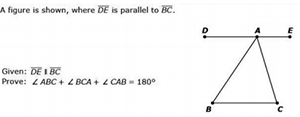 Drag statements from the statements column and reasons from the reasons column to their correct location to complete the proof. |
N/A | DDHT: Drag-and-Drop Hot Text |
Related Courses
| Course Number1111 | Course Title222 |
| 1200400: | Foundational Skills in Mathematics 9-12 (Specifically in versions: 2014 - 2015, 2015 - 2022, 2022 - 2024, 2024 and beyond (current)) |
| 1206310: | Geometry (Specifically in versions: 2014 - 2015, 2015 - 2022, 2022 - 2024, 2024 and beyond (current)) |
| 1206320: | Geometry Honors (Specifically in versions: 2014 - 2015, 2015 - 2022, 2022 - 2024, 2024 and beyond (current)) |
| 1206315: | Geometry for Credit Recovery (Specifically in versions: 2014 - 2015, 2015 - 2022, 2022 - 2024, 2024 and beyond (current)) |
| 7912065: | Access Geometry (Specifically in versions: 2015 - 2022, 2022 and beyond (current)) |
Related Resources
Formative Assessments
| Name | Description |
| The Measure of an Angle of a Triangle | Students are given the measure of one interior angle of an isosceles triangle and are asked to find the measure of another interior angle. |
| Proving the Triangle Inequality Theorem | Students are asked to prove the Triangle Inequality Theorem. |
| An Isosceles Trapezoid Problem | Students are asked to explain why the sum of the lengths of the diagonals of an isosceles trapezoid is less than its perimeter. |
| Triangles and Midpoints | Students are asked to explain why a quadrilateral formed by drawing the midsegments of a triangle is a parallelogram and to find the perimeter of the triangle formed by the midsegments. |
| Interior Angles of a Polygon | Students are asked to explain why the sum of the measures of the interior angles of a convex n-gon is given by the formula (n – 2)180°. |
| The Third Side of a Triangle | Students are given the lengths of two sides of a triangle and asked to describe all possible lengths of the remaining side. |
| Locating the Missing Midpoint | Students are given a triangle in which the midpoints of two sides are shown and are asked to describe a method for locating the midpoint of the remaining side using only a straight edge and pencil. |
| Median Concurrence Proof | Students are asked to prove that the medians of a triangle are concurrent. |
| Triangle Sum Proof | Students are asked prove that the measures of the interior angles of a triangle sum to 180°. |
| Isosceles Triangle Proof | Students are asked to prove that the base angles of an isosceles triangle are congruent. |
| Triangle Midsegment Proof | Students are asked to prove that the segment joining the midpoints of two sides of a triangle is parallel to the third side of the triangle and half of its length. |
Lesson Plans
| Name | Description |
| Engineering Design Challenge: Exploring Structures in High School Geometry | Students explore ideas on how civil engineers use triangles when constructing bridges. Students will apply knowledge of congruent triangles to build and test their own bridges for stability. |
| Triangle Mid-Segment Theorem | The Triangle Mid-Segment Theorem is used to show the writing of a coordinate proof clearly and concisely. |
| Keeping Triangles in Balance: Discovering Triangle Centroid is Concurrent Medians | In this lesson, students identify, analyze, and understand the Triangle Centroid Theorem. Students discover that the centroid is a point of concurrency for the medians of a triangle and recognize its associated usage with the center of gravity or barycenter. This set of instructional materials provides the teacher with hands-on activities using technology as well as paper-and-pencil methods. |
| Triangles: To B or not to B? | Students will explore triangle inequalities that exist between side lengths with physical models of segments. They will determine when a triangle can/cannot be created with given side lengths and a range of lengths that can create a triangle. |
| Observing the Centroid | Students will construct the medians of a triangle then investigate the intersections of the medians. |
| Determination of the Optimal Point | Students will use dynamic geometry software to determine the optimal location for a facility under a variety of scenarios. The experiments will suggest a relation between the optimal point and a common concept in geometry; in some cases, there will be a connection to a statistical concept. Algebra can be used to verify some of the conjectures. |
| The Centroid | Students will construct the centroid of a triangle using graph paper or GeoGebra in order to develop conjectures. Then students will prove that the medians of a triangle actually intersect using the areas of triangles. |
| Discovering Triangle Sum | This lesson is designed to address all levels and types of learners to improve understanding of the triangle sum theorem from the simplest perspective and progress steadily by teacher led activities to a more complex level. It is intended to create a solid foundation in geometric reasoning to help students advance to higher levels in confidence. |
| Geometer Sherlock: Triangle Investigations | The students will investigate and discover relationships within triangles; such as, the triangle angle sum theorem, and the triangle inequality theorem. |
| Proofs of the Pythagorean Theorem | This lesson enriches the students' perspective of geometric proofs that are not in two-column form. Students will apply algebaic skills and geometric properties to prove the Pythagorean Theorem in a variety of ways. This unit is designed to help you identify and assist students who have difficulties in: |
| Evaluating Statements About Length and Area | This lesson unit is intended to help you assess how well students can understand the concepts of length and area, use the concept of area in proving why two areas are or are not equal and construct their own examples and counterexamples to help justify or refute conjectures. |
| Intersecting Medians and the Resulting Ratios | This lesson leads students to discover empirically that the distance from each vertex to the intersection of the medians of a triangle is two-thirds of the total length of each median. |
| Shape It Up | Students will derive the formula for the sum of the interior angles of a polygon by drawing diagonals and applying the Triangle Sum Theorem. The measure of each interior angle of a regular polygon is also determined. |
| Right turn, Clyde! | Students will develop their knowledge of perpendicular bisectors & point of concurrency of a triangle, as well as construct perpendicular bisectors through real world problem solving with a map. |
| Halfway to the Middle! | Students will develop their knowledge of mid-segments of a triangle, construct and provide lengths of mid-segments. |
| Determination of the Optimal Point | Students will use dynamic geometry software to determine the optimal location for a facility under a variety of scenarios. The experiments will suggest a relation between the optimal point and a common concept in geometry; in some cases, there will be a connection to a statistical concept. Algebra can be used to verify some of the conjectures. |
Problem-Solving Tasks
| Name | Description |
| Finding the area of an equilateral triangle | This problem solving task asks students to find the area of an equilateral triangle. Various solutions are presented that include the Pythagoren theorem and trigonometric functions. |
| Seven Circles I | This task is intended to help model a concrete situation with geometry. Placing the seven pennies in a circular pattern is a concrete and fun experiment which leads to a genuine mathematical question: does the physical model with pennies give insight into what happens with seven circles in the plane? |
Tutorials
| Name | Description |
| Sum of Exterior Angles of an Irregular Pentagon | In this video, students will learn how to use what they know about the sum of angles in a triangle to determine the sum of the exterior angles of an irregular pentagon. |
| Proof: Sum of Measures of Angles in a Triangle Are 180 | Lets prove that the sum of interior angles of a triangle are equal to 180 degrees. |
| Triangle Angle Example 1 | Let's find the measure of an angle, using interior and exterior angle measurements. |
Student Resources
Problem-Solving Tasks
| Name | Description |
| Finding the area of an equilateral triangle: | This problem solving task asks students to find the area of an equilateral triangle. Various solutions are presented that include the Pythagoren theorem and trigonometric functions. |
| Seven Circles I: | This task is intended to help model a concrete situation with geometry. Placing the seven pennies in a circular pattern is a concrete and fun experiment which leads to a genuine mathematical question: does the physical model with pennies give insight into what happens with seven circles in the plane? |
Tutorials
| Name | Description |
| Sum of Exterior Angles of an Irregular Pentagon: | In this video, students will learn how to use what they know about the sum of angles in a triangle to determine the sum of the exterior angles of an irregular pentagon. |
| Proof: Sum of Measures of Angles in a Triangle Are 180: | Lets prove that the sum of interior angles of a triangle are equal to 180 degrees. |
| Triangle Angle Example 1: | Let's find the measure of an angle, using interior and exterior angle measurements. |
Parent Resources
Problem-Solving Tasks
| Name | Description |
| Finding the area of an equilateral triangle: | This problem solving task asks students to find the area of an equilateral triangle. Various solutions are presented that include the Pythagoren theorem and trigonometric functions. |
| Seven Circles I: | This task is intended to help model a concrete situation with geometry. Placing the seven pennies in a circular pattern is a concrete and fun experiment which leads to a genuine mathematical question: does the physical model with pennies give insight into what happens with seven circles in the plane? |
