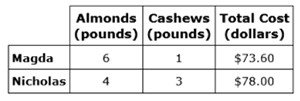
Solve systems of linear equations exactly and approximately (e.g., with graphs), focusing on pairs of linear equations in two variables.
| Course Number1111 |
Course Title222 |
| 1200310: | Algebra 1 (Specifically in versions: 2014 - 2015, 2015 - 2022, 2022 - 2024, 2024 and beyond (current)) |
| 1200320: | Algebra 1 Honors (Specifically in versions: 2014 - 2015, 2015 - 2022, 2022 - 2024, 2024 and beyond (current)) |
| 1200330: | Algebra 2 (Specifically in versions: 2014 - 2015, 2015 - 2022, 2022 - 2024, 2024 and beyond (current)) |
| 1200340: | Algebra 2 Honors (Specifically in versions: 2014 - 2015, 2015 - 2022, 2022 - 2024, 2024 and beyond (current)) |
| 1200370: | Algebra 1-A (Specifically in versions: 2014 - 2015, 2015 - 2022, 2022 - 2024, 2024 and beyond (current)) |
| 1200400: | Foundational Skills in Mathematics 9-12 (Specifically in versions: 2014 - 2015, 2015 - 2022, 2022 - 2024, 2024 and beyond (current)) |
| 1200410: | Mathematics for College Success (Specifically in versions: 2014 - 2015, 2015 - 2022 (course terminated)) |
| 1200700: | Mathematics for College Algebra (Specifically in versions: 2014 - 2015, 2015 - 2022 (course terminated)) |
| 7912070: | Access Mathematics for Liberal Arts (Specifically in versions: 2014 - 2015, 2015 - 2018, 2018 - 2019, 2019 - 2022, 2022 - 2023, 2023 and beyond (current)) |
| 7912080: | Access Algebra 1A (Specifically in versions: 2014 - 2015, 2015 - 2018, 2018 - 2019, 2019 - 2022, 2022 and beyond (current)) |
| 1200315: | Algebra 1 for Credit Recovery (Specifically in versions: 2014 - 2015, 2015 - 2022, 2022 - 2024, 2024 and beyond (current)) |
| 1200335: | Algebra 2 for Credit Recovery (Specifically in versions: 2014 - 2015, 2015 - 2019 (course terminated)) |
| 1200375: | Algebra 1-A for Credit Recovery (Specifically in versions: 2014 - 2015, 2015 - 2022, 2022 - 2024, 2024 and beyond (current)) |
| 7912100: | Fundamental Algebraic Skills (Specifically in versions: 2013 - 2015, 2015 - 2017 (course terminated)) |
| 1207300: | Liberal Arts Mathematics 1 (Specifically in versions: 2014 - 2015, 2015 - 2022 (course terminated)) |
| 7912075: | Access Algebra 1 (Specifically in versions: 2014 - 2015, 2015 - 2018, 2018 - 2019, 2019 - 2022, 2022 and beyond (current)) |
| 7912095: | Access Algebra 2 (Specifically in versions: 2016 - 2018, 2018 - 2019, 2019 - 2022, 2022 and beyond (current)) |
| 1200387: | Mathematics for Data and Financial Literacy (Specifically in versions: 2016 and beyond (current)) |
| Name |
Description |
| Steel vs. Wooden Roller Coaster Lab | This lesson is a Follow Up Activity to the Algebra Institute and allows students to apply their skills on analyzing bivariate data. This STEM lesson allows students the opportunity to investigate if there is a linear relationship between a coaster's height and speed. Using technology the students can determine the line of best fit, correlation coefficient and use the line for interpolation. This lesson also uses prior knowledge and has students solve systems of equations graphically to determine which type of coaster is faster. |
| Solving Linear Equations in Two Variables | This lesson unit is intended to help you assess how well students can formulate and solve problems using algebra and, in particular, to identify and help students who have difficulties solving a problem using two linear equations with two variables and interpreting the meaning of algebraic expressions. |
| Optimization Problems: Boomerangs | This lesson is designed to help students develop strategies for solving optimization problems. Such problems typically involve scenarios where limited resources must be used to greatest effect, as in, for example, the allocation of time and materials to maximize profit. |
| Solving Systems of Equations by Substitution | In this lesson, students will learn how to solve systems of equations using substitution. Students will have the opportunity for small group and whole class discussion related to using substitution. |
| Systems of the Linear Round Table | This lesson is a follow-up review of systems of linear equations. Students will complete a group activity called Simultaneous Round Table to solve given systems of equations. Students will solve by graphing, elimination, and substitution. Each student will also perform error analysis on the work from their peers, which will allow them to help each other to correct those mistakes. Class will use data from error analysis to create a plan of action to decrease errors in their work. Students will discuss the concepts and analyze problems with each other. These concepts were taught in an earlier lesson. This lesson will also help students identify common mistakes and find solutions to remedy them. |
| Exploring Systems of Equations using Graphing Calculators | This lesson plan introduces the concept of graphing a system of linear equations. Students will use graphing technology to explore the meaning of the solution of a linear system including solutions that correspond to intersecting lines, parallel lines, and coinciding lines.
Students will also do graph linear systems by hand. |
| Graphing vs. Substitution. Which would you choose? | Students will solve multiple systems of equations using two methods: graphing and substitution. This will help students to make a connection between the two methods and realize that they will indeed get the same solution graphically and algebraically. Students will compare the two methods and think about ways to decide which method to use for a particular problem. This lesson connects prior instruction on solving systems of equations graphically with using algebraic methods to solve systems of equations. |
| My Candles are MELTING! | In this lesson, students will apply their knowledge to model a real-world linear situation in a variety of ways. They will analyze a situation in which 2 candles burn at different rates. They will create a table of values, determine a linear equation, and graph each to determine if and when the candles will ever be the same height. They will also determine the domain and range of their functions and determine whether there are constraints on their functions. |
| Exploring Systems with Piggies, Pizzas and Phones | Students write and solve linear equations from real-life situations. |
| When Two Lines Meet | Learn how to graph a system of two equations in two variables, and find the solution(s), if one exists. |
| Name |
Description |
| Quinoa Pasta 3 | This mathematical modeling task also illustrates making sense of a problem. Students are only told that there are two ingredients in the pasta and they have a picture of the box. It might even be better to just show the picture of the box, or to bring in the box and ask the students to pose the question themselves. The brand of pasta is quite commonly available at supermarkets or health food stores such as Whole Foods and even at Amazon.com. The box has the nutritional label and a reference to the website where the students can find other information about the ingredients |
| Quinoa Pasta 2 | This mathematical modeling task also illustrates making sense of a problem. Students are given all the relevant information on the nutritional labels, but they have to figure out how to use this information. They have to come up with the idea that they can set up two equations in two unknowns to solve the problem. |
| Pairs of Whole Numbers | This task addresses solving systems of linear equations, and provides a simple example of a system with three equations and three unknown. Two (of many) methods for solving the system are presented. The first takes the given information to make three equations in three unknowns which can then be solved via algebraic manipulation to find the three numbers. The second solution is more clever, creating a single equation in three unknowns from the given information. This equation is then combined with the given information about the sums of pairs of numbers to deduce what the third number is. In reality, this solution is not simpler than the first: rather it sets up a slightly different set of equations which can be readily solved (the key being to take the sum of the three equations in the first solution). It provides a good opportunity for the instructor to show different methods for solving the same system of linear equations. |
| Cash Box | The given solutions for this task involve the creation and solving of a system of two equations and two unknowns, with the caveat that the context of the problem implies that we are interested only in non-negative integer solutions. Indeed, in the first solution, we must also restrict our attention to the case that one of the variables is further even. This aspect of the task is illustrative of the mathematical practice of modeling with mathematics, and crucial as the system has an integer solution for both situations, that is, whether we include the dollar on the floor in the cash box or not. |
| Accurately weighing pennies II | This task is a somewhat more complicated version of "Accurately weighing pennies I'' as a third equation is needed in order to solve part (a) explicitly. Instead, students have to combine the algebraic techniques with some additional problem-solving (numerical reasoning, informed guess-and-check, etc.) Part (b) is new to this task, as with only two types of pennies the weight of the collection determines how many pennies of each type are in the collection. This is no longer the case with three different weights but in this particular case, a collection of 50 is too small to show any ambiguity. This is part of the reason for part (c) of the question where the weight alone no longer determines which type of pennies are in the roll. This shows how important levels of accuracy in measurement are as the answer to part (b) could be different if we were to measure on a scale which is only accurate to the nearest tenth of a gram instead of to the nearest hundredth of a gram. |
| Accurately weighing pennies I | This problem involves solving a system of algebraic equations from a context: depending how the problem is interpreted, there may be one equation or two. The main work in parts (a) and (b) is in setting up the equation(s) appropriately. Question (c) is more subtle and it requires thinking carefully about the accuracy available in a particular measurement (weight). The first two parts of this task could be used for instructional or assessment purposes while the third part should strictly be implemented for instructional purposes. |
| Selling Fuel Oil at a Loss | The task is a modeling problem which ties in to financial decisions faced routinely by businesses, namely the balance between maintaining inventory and raising short-term capital for investment or re-investment in developing the business. |
| Name |
Description |
| Example 3: Solving Systems by Elimination | This video is an example of solving a system of linear equations by elimination where the system has infinite solutions. |
| Solving Systems of Linear Equations with Elimination Example 1 | This video shows how to solve a system of equations through simple elimination. |
| Inconsistent Systems of Equations | This video explains how to identify systems of equations without a solution. |
| Example 2: Solving Systems by Elimination | This video shows how to solve systems of equations by elimination. |
| Addition Elimination Example 1 | This video is an introduction to the elimination method of solving a system of equations. |
| Example 3: Solving Systems by Substitution | This example demonstrates solving a system of equations algebraically and graphically. |
| Substitution Method Example 2 | This video demonstrates a system of equations with no solution. |
| The Substitution Method | This video shows how to solve a system of equations using the substitution method. |
| Systems of Equations Word Problems Example 1 | This video demonstrates solving a word problem by creating a system of linear equations that represents the situation and solving them using elimination. |
| Graphing systems of equations | In this tutorial, students will learn how to solve and graph a system of equations.
|
| Solving system of equations by graphing | This tutorial shows students how to solve a system of linear equations by graphing the two equations on the same coordinate plane and identifying the intersection point.
|
| Solving a system of equations by graphing | This tutorial shows how to solve a system of equations by graphing. Students will see what a no solution system of equations looks like in a graph. |
| Solving a system of equations using substitution | This tutorial shows how to solve a system of equations using substitution.
|
| Solving Basic Systems Using the Elimination Method | This 8 minute video will show step-by-step directions for using the elimination method to solve a system of linear equations. |
| Solving Inconsistent or Dependent Systems | When solving a system of linear equations in x and y with a single solution, we get a unique pair of values for x and y. But what happens when try to solve a system with no solutions or an infinite number of solutions? |
| Inconsistent, Dependent, and Independent Systems | Systems of two linear equations in two variables can have a single solution, no solutions, or an infinite number of solutions. This video gives a great description of inconsistent, dependent, and independent systems. A consistent independent system of equations will have one solution. A consistent dependent system of equations will have infinite number of solutions, and an inconsistent system of equations will have no solution. This tutorial also provides information on how to distinguish a given system of linear equations as inconsistent, independent, or dependent system by looking at the slope and intercept. |
| Solving Systems of Equations by Elimination | Systems of two equations in x and y can be solved by adding the equations to create a new equation with one variable eliminated. This new equation can then be solved to find the value of the remaining variable. That value is then substituted into either equation to find the value of other variable. |
| Solving Systems of Equations by Substitution | A system of two equations in x and y can be solved by rearranging one equation to represent x in terms of y, and "substituting" this expression for x in the other equation. This creates an equation with only y which can then be solved to find y's value. This value can then be substituted into either equation to find the value of x. |
| Name |
Description |
| Quinoa Pasta 3: | This mathematical modeling task also illustrates making sense of a problem. Students are only told that there are two ingredients in the pasta and they have a picture of the box. It might even be better to just show the picture of the box, or to bring in the box and ask the students to pose the question themselves. The brand of pasta is quite commonly available at supermarkets or health food stores such as Whole Foods and even at Amazon.com. The box has the nutritional label and a reference to the website where the students can find other information about the ingredients |
| Quinoa Pasta 2: | This mathematical modeling task also illustrates making sense of a problem. Students are given all the relevant information on the nutritional labels, but they have to figure out how to use this information. They have to come up with the idea that they can set up two equations in two unknowns to solve the problem. |
| Pairs of Whole Numbers: | This task addresses solving systems of linear equations, and provides a simple example of a system with three equations and three unknown. Two (of many) methods for solving the system are presented. The first takes the given information to make three equations in three unknowns which can then be solved via algebraic manipulation to find the three numbers. The second solution is more clever, creating a single equation in three unknowns from the given information. This equation is then combined with the given information about the sums of pairs of numbers to deduce what the third number is. In reality, this solution is not simpler than the first: rather it sets up a slightly different set of equations which can be readily solved (the key being to take the sum of the three equations in the first solution). It provides a good opportunity for the instructor to show different methods for solving the same system of linear equations. |
| Cash Box: | The given solutions for this task involve the creation and solving of a system of two equations and two unknowns, with the caveat that the context of the problem implies that we are interested only in non-negative integer solutions. Indeed, in the first solution, we must also restrict our attention to the case that one of the variables is further even. This aspect of the task is illustrative of the mathematical practice of modeling with mathematics, and crucial as the system has an integer solution for both situations, that is, whether we include the dollar on the floor in the cash box or not. |
| Accurately weighing pennies II: | This task is a somewhat more complicated version of "Accurately weighing pennies I'' as a third equation is needed in order to solve part (a) explicitly. Instead, students have to combine the algebraic techniques with some additional problem-solving (numerical reasoning, informed guess-and-check, etc.) Part (b) is new to this task, as with only two types of pennies the weight of the collection determines how many pennies of each type are in the collection. This is no longer the case with three different weights but in this particular case, a collection of 50 is too small to show any ambiguity. This is part of the reason for part (c) of the question where the weight alone no longer determines which type of pennies are in the roll. This shows how important levels of accuracy in measurement are as the answer to part (b) could be different if we were to measure on a scale which is only accurate to the nearest tenth of a gram instead of to the nearest hundredth of a gram. |
| Accurately weighing pennies I: | This problem involves solving a system of algebraic equations from a context: depending how the problem is interpreted, there may be one equation or two. The main work in parts (a) and (b) is in setting up the equation(s) appropriately. Question (c) is more subtle and it requires thinking carefully about the accuracy available in a particular measurement (weight). The first two parts of this task could be used for instructional or assessment purposes while the third part should strictly be implemented for instructional purposes. |
| Selling Fuel Oil at a Loss: | The task is a modeling problem which ties in to financial decisions faced routinely by businesses, namely the balance between maintaining inventory and raising short-term capital for investment or re-investment in developing the business. |
| Name |
Description |
| Example 3: Solving Systems by Elimination: | This video is an example of solving a system of linear equations by elimination where the system has infinite solutions. |
| Solving Systems of Linear Equations with Elimination Example 1: | This video shows how to solve a system of equations through simple elimination. |
| Inconsistent Systems of Equations: | This video explains how to identify systems of equations without a solution. |
| Example 2: Solving Systems by Elimination: | This video shows how to solve systems of equations by elimination. |
| Addition Elimination Example 1: | This video is an introduction to the elimination method of solving a system of equations. |
| Example 3: Solving Systems by Substitution: | This example demonstrates solving a system of equations algebraically and graphically. |
| Substitution Method Example 2: | This video demonstrates a system of equations with no solution. |
| The Substitution Method: | This video shows how to solve a system of equations using the substitution method. |
| Systems of Equations Word Problems Example 1: | This video demonstrates solving a word problem by creating a system of linear equations that represents the situation and solving them using elimination. |
| Graphing systems of equations: | In this tutorial, students will learn how to solve and graph a system of equations.
|
| Solving system of equations by graphing: | This tutorial shows students how to solve a system of linear equations by graphing the two equations on the same coordinate plane and identifying the intersection point.
|
| Solving a system of equations by graphing: | This tutorial shows how to solve a system of equations by graphing. Students will see what a no solution system of equations looks like in a graph. |
| Solving a system of equations using substitution: | This tutorial shows how to solve a system of equations using substitution.
|
| Solving Basic Systems Using the Elimination Method: | This 8 minute video will show step-by-step directions for using the elimination method to solve a system of linear equations. |
| Solving Inconsistent or Dependent Systems: | When solving a system of linear equations in x and y with a single solution, we get a unique pair of values for x and y. But what happens when try to solve a system with no solutions or an infinite number of solutions? |
| Inconsistent, Dependent, and Independent Systems: | Systems of two linear equations in two variables can have a single solution, no solutions, or an infinite number of solutions. This video gives a great description of inconsistent, dependent, and independent systems. A consistent independent system of equations will have one solution. A consistent dependent system of equations will have infinite number of solutions, and an inconsistent system of equations will have no solution. This tutorial also provides information on how to distinguish a given system of linear equations as inconsistent, independent, or dependent system by looking at the slope and intercept. |
| Solving Systems of Equations by Elimination: | Systems of two equations in x and y can be solved by adding the equations to create a new equation with one variable eliminated. This new equation can then be solved to find the value of the remaining variable. That value is then substituted into either equation to find the value of other variable. |
| Solving Systems of Equations by Substitution: | A system of two equations in x and y can be solved by rearranging one equation to represent x in terms of y, and "substituting" this expression for x in the other equation. This creates an equation with only y which can then be solved to find y's value. This value can then be substituted into either equation to find the value of x. |
| Name |
Description |
| Quinoa Pasta 3: | This mathematical modeling task also illustrates making sense of a problem. Students are only told that there are two ingredients in the pasta and they have a picture of the box. It might even be better to just show the picture of the box, or to bring in the box and ask the students to pose the question themselves. The brand of pasta is quite commonly available at supermarkets or health food stores such as Whole Foods and even at Amazon.com. The box has the nutritional label and a reference to the website where the students can find other information about the ingredients |
| Quinoa Pasta 2: | This mathematical modeling task also illustrates making sense of a problem. Students are given all the relevant information on the nutritional labels, but they have to figure out how to use this information. They have to come up with the idea that they can set up two equations in two unknowns to solve the problem. |
| Pairs of Whole Numbers: | This task addresses solving systems of linear equations, and provides a simple example of a system with three equations and three unknown. Two (of many) methods for solving the system are presented. The first takes the given information to make three equations in three unknowns which can then be solved via algebraic manipulation to find the three numbers. The second solution is more clever, creating a single equation in three unknowns from the given information. This equation is then combined with the given information about the sums of pairs of numbers to deduce what the third number is. In reality, this solution is not simpler than the first: rather it sets up a slightly different set of equations which can be readily solved (the key being to take the sum of the three equations in the first solution). It provides a good opportunity for the instructor to show different methods for solving the same system of linear equations. |
| Cash Box: | The given solutions for this task involve the creation and solving of a system of two equations and two unknowns, with the caveat that the context of the problem implies that we are interested only in non-negative integer solutions. Indeed, in the first solution, we must also restrict our attention to the case that one of the variables is further even. This aspect of the task is illustrative of the mathematical practice of modeling with mathematics, and crucial as the system has an integer solution for both situations, that is, whether we include the dollar on the floor in the cash box or not. |
| Accurately weighing pennies II: | This task is a somewhat more complicated version of "Accurately weighing pennies I'' as a third equation is needed in order to solve part (a) explicitly. Instead, students have to combine the algebraic techniques with some additional problem-solving (numerical reasoning, informed guess-and-check, etc.) Part (b) is new to this task, as with only two types of pennies the weight of the collection determines how many pennies of each type are in the collection. This is no longer the case with three different weights but in this particular case, a collection of 50 is too small to show any ambiguity. This is part of the reason for part (c) of the question where the weight alone no longer determines which type of pennies are in the roll. This shows how important levels of accuracy in measurement are as the answer to part (b) could be different if we were to measure on a scale which is only accurate to the nearest tenth of a gram instead of to the nearest hundredth of a gram. |
| Accurately weighing pennies I: | This problem involves solving a system of algebraic equations from a context: depending how the problem is interpreted, there may be one equation or two. The main work in parts (a) and (b) is in setting up the equation(s) appropriately. Question (c) is more subtle and it requires thinking carefully about the accuracy available in a particular measurement (weight). The first two parts of this task could be used for instructional or assessment purposes while the third part should strictly be implemented for instructional purposes. |
| Selling Fuel Oil at a Loss: | The task is a modeling problem which ties in to financial decisions faced routinely by businesses, namely the balance between maintaining inventory and raising short-term capital for investment or re-investment in developing the business. |