General Information
Clusters should not be sorted from Major to Supporting and then taught in that order. To do so would strip the coherence of the mathematical ideas and miss the opportunity to enhance the major work of the grade with the supporting clusters.
Test Item Specifications
Items must not include shapes beyond triangles
Neutral
No context
Sample Test Items (4)
| Test Item # | Question | Difficulty | Type |
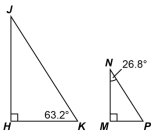
| Sample Item 1 | What is the measure of
|
N/A | EE: Equation Editor |
| Sample Item 2 | What is the measure of
|
N/A | EE: Equation Editor |
| Sample Item 3 | Two similar triangles are shown.
What is the measure of |
N/A | EE: Equation Editor |
| Sample Item 4 | A figure with parallel lines m and n is shown.
What is the measure, in degrees, of |
N/A | EE: Equation Editor |
Related Courses
| Course Number1111 | Course Title222 |
| 1205050: | M/J Accelerated Mathematics Grade 7 (Specifically in versions: 2014 - 2015, 2015 - 2020, 2020 - 2022, 2022 - 2024, 2024 and beyond (current)) |
| 1205070: | M/J Grade 8 Pre-Algebra (Specifically in versions: 2014 - 2015, 2015 - 2022, 2022 - 2024, 2024 and beyond (current)) |
| 1204000: | M/J Foundational Skills in Mathematics 6-8 (Specifically in versions: 2014 - 2015, 2015 - 2022, 2022 - 2024, 2024 and beyond (current)) |
| 7812030: | Access M/J Grade 8 Pre-Algebra (Specifically in versions: 2014 - 2015, 2015 - 2018, 2018 - 2019, 2019 - 2022, 2022 and beyond (current)) |
Related Resources
Educational Software / Tool
| Name | Description |
| Glossary | This resource is an online glossary to find the meaning of math terms. Students can also use the online glossary to find words that are related to the word typed in the search box. For example: Type in "transversal" and 11 other terms will come up. Click on one of those terms and its meaning is displayed. |
Formative Assessments
| Name | Description |
| What Is the Triangle Relationship? | Students are asked to write an informal justification of the AA Similarity Theorem. |
| Same Side Interior Angles | Students are asked to describe and justify the relationship between same side interior angles. |
| Justifying the Triangle Sum Theorem | Students are asked to provide an informal justification of the Triangle Sum Theorem. |
| Justifying the Exterior Angle of a Triangle Theorem | Students are asked to apply the Exterior Angle of a Triangle Theorem and provide an informal justification. |
| Justifying Angle Relationships | Students are asked to describe and justify the relationship between corresponding angles and alternate interior angles. |
Image/Photograph
| Name | Description |
| Angles (Clipart ETC) | This large collection of clipart contains images of angles that can be freely used in lesson plans, worksheets, and presentations. |
Lesson Plans
| Name | Description |
| Identifying Similar Triangles | This 105-minute lesson series helps teachers assess how students reason about geometry, including how they use facts about the angle sum and exterior angles of triangles to calculate missing angles, apply angle theorems to parallel lines cut by a transversal, and interpret geometrical diagrams using mathematical properties to identify similarity of triangles. In order to complete this lesson, students will need whiteboards, pens, wipes, copies of the assessment tasks, pencils, markers, scissors, glue sticks, and poster paper. |
| Identifying Similar Triangles | This 105-minute lesson series helps teachers assess how students reason about geometry, including how they use facts about the angle sum and exterior angles of triangles to calculate missing angles, apply angle theorems to parallel lines cut by a transversal, and interpret geometrical diagrams using mathematical properties to identify similarity of triangles. In order to complete this lesson, students will need whiteboards, pens, wipes, copies of the assessment tasks, pencils, markers, scissors, glue sticks, and poster paper. |
| Scientific calculations from a distant planet | Students will act as mathematicians and scientists as they use models, observations and space science concepts to perform calculations and draw inferences regarding a fictional solar system with three planets in circular orbits around a sun. Among the calculations are estimates of the size of the home planet (using a method more than 2000 years old) and the relative distances of the planets from their sun. |
| Shape It Up | Students will derive the formula for the sum of the interior angles of a polygon by drawing diagonals and applying the Triangle Sum Theorem. The measure of each interior angle of a regular polygon is also determined. |
| The Ins and Outs of Polygons | In this lesson, students will explore how to find the sum of the measures of the angles of a triangle, then use this knowledge to find the sum of the measures of angles of other polygons. They will also be able to find the sum of the exterior angles of triangles and other polygons. Using both concepts, students will be able to find missing measurements. |
| Triangles: Finding Interior Angle Measures | The lesson begins with a hands-on activity and then an experiment with a GeoGebra-based computer model to discover the Triangle Angle Sum Theorem. The students write and solve equations to find missing angle measures in a variety of examples. |
| Special Angle Pairs Discovery Activity | This lesson uses a discovery approach to identify the special angles formed when a set of parallel lines is cut by a transversal. During this lesson, students identify the angle pair and the relationship between the angles. Students use this relationship and special angle pairs to make conjectures about which angle pairs are considered special angles. |
| How Many Degrees? | This lesson facilitates the discovery of a formula for the sum of the interior angles of a regular polygon. Students will draw all the diagonals from one vertex of various polygons to find how many triangles are formed. They will use this and their prior knowledge of triangles to figure out the sum of the interior angles. This will lead to the development of a formula for finding the sum of interior angles and the measure of one interior angle. |
| Help me Find my Relationship! | In this lesson, students will investigate the relationship between angles when parallel lines are cut by a transversal. Students will identify angles, and find angle measures, and they will use the free application GeoGebra (see download link under Suggested Technology) to provide students with a visual representation of angle relationships. |
| An Investigation of Angle Relationships Formed by Parallel Lines and a Transversal Using GeoGebra | In this lesson, students will discover angle relationships formed when two parallel lines are cut by a transversal (corresponding, alternate interior, alternate exterior, same-side interior, same-side exterior). They will establish definitions and identify whether these angle pairs are supplementary or congruent. |
Problem-Solving Tasks
| Name | Description |
| Tile Patterns I: octagons and squares | Students use interior and exterior angles to to verify attributes of an octagon and square. Students are given a tile pattern involving congruent regular octagons and squares. |
| Find the Angle | Use informal arguments to establish facts about the angle sum and exterior angle of triangles, about the angles created when parallel lines are cut by a transversal, and the angle-angle criterion for similarity of triangles. |
| Find the Missing Angle | This task provides students the opportunity to see how the mathematical ideas embedded in the standards and clusters mature over time. The task uses facts about supplementary, complementary, vertical, adjacent, and alternate interior angles in a multi-step problem to write and solve simple equations for an unknown angle in a figure. It is a good introduction to writing paragraphs, 2-column, and/or flow chart proofs. |
| Tile Patterns II: hexagons | This task is ideally suited for instruction purposes where students can take their time and develop several of the standards, as the mathematical content is directly related to, but somewhat exceeds, the content of the standard on sums of angles in triangles. Careful analysis of the angles requires students to construct valid arguments using abstract and quantitative reasoning. Producing the picture in part (c) helps students identify a common mathematical argument repeated multiple times. Students may use pattern blocks to develop the intuition for decomposing the hexagon into triangles. |
Student Center Activity
| Name | Description |
| Edcite: Mathematics Grade 8 | Students can practice answering mathematics questions on a variety of topics. With an account, students can save their work and send it to their teacher when complete. |
Tutorials
| Name | Description |
| Finding Missing Angle Measures | In this video, we find missing angle measures from a variety of examples.
|
| Proving congruent angles | In this tutorial, students are asked to prove two angles congruent when given limited information. Students need to have a foundation of parallel lines, transversals and triangles before viewing this video.
|
| Sum of measures of triangles proof | This video gives the proof of sum of measures of angles in a triangle. This video is beneficial for both Algebra and Geometry students. |
Student Resources
Educational Software / Tool
| Name | Description |
| Glossary: | This resource is an online glossary to find the meaning of math terms. Students can also use the online glossary to find words that are related to the word typed in the search box. For example: Type in "transversal" and 11 other terms will come up. Click on one of those terms and its meaning is displayed. |
Problem-Solving Tasks
| Name | Description |
| Tile Patterns I: octagons and squares: | Students use interior and exterior angles to to verify attributes of an octagon and square. Students are given a tile pattern involving congruent regular octagons and squares. |
| Find the Angle: | Use informal arguments to establish facts about the angle sum and exterior angle of triangles, about the angles created when parallel lines are cut by a transversal, and the angle-angle criterion for similarity of triangles. |
| Find the Missing Angle: | This task provides students the opportunity to see how the mathematical ideas embedded in the standards and clusters mature over time. The task uses facts about supplementary, complementary, vertical, adjacent, and alternate interior angles in a multi-step problem to write and solve simple equations for an unknown angle in a figure. It is a good introduction to writing paragraphs, 2-column, and/or flow chart proofs. |
| Tile Patterns II: hexagons: | This task is ideally suited for instruction purposes where students can take their time and develop several of the standards, as the mathematical content is directly related to, but somewhat exceeds, the content of the standard on sums of angles in triangles. Careful analysis of the angles requires students to construct valid arguments using abstract and quantitative reasoning. Producing the picture in part (c) helps students identify a common mathematical argument repeated multiple times. Students may use pattern blocks to develop the intuition for decomposing the hexagon into triangles. |
Student Center Activity
| Name | Description |
| Edcite: Mathematics Grade 8: | Students can practice answering mathematics questions on a variety of topics. With an account, students can save their work and send it to their teacher when complete. |
Tutorials
| Name | Description |
| Finding Missing Angle Measures: | In this video, we find missing angle measures from a variety of examples.
|
| Proving congruent angles: | In this tutorial, students are asked to prove two angles congruent when given limited information. Students need to have a foundation of parallel lines, transversals and triangles before viewing this video.
|
| Sum of measures of triangles proof: | This video gives the proof of sum of measures of angles in a triangle. This video is beneficial for both Algebra and Geometry students. |
Parent Resources
Educational Software / Tool
| Name | Description |
| Glossary: | This resource is an online glossary to find the meaning of math terms. Students can also use the online glossary to find words that are related to the word typed in the search box. For example: Type in "transversal" and 11 other terms will come up. Click on one of those terms and its meaning is displayed. |
Problem-Solving Tasks
| Name | Description |
| Tile Patterns I: octagons and squares: | Students use interior and exterior angles to to verify attributes of an octagon and square. Students are given a tile pattern involving congruent regular octagons and squares. |
| Find the Angle: | Use informal arguments to establish facts about the angle sum and exterior angle of triangles, about the angles created when parallel lines are cut by a transversal, and the angle-angle criterion for similarity of triangles. |
| Find the Missing Angle: | This task provides students the opportunity to see how the mathematical ideas embedded in the standards and clusters mature over time. The task uses facts about supplementary, complementary, vertical, adjacent, and alternate interior angles in a multi-step problem to write and solve simple equations for an unknown angle in a figure. It is a good introduction to writing paragraphs, 2-column, and/or flow chart proofs. |
| Tile Patterns II: hexagons: | This task is ideally suited for instruction purposes where students can take their time and develop several of the standards, as the mathematical content is directly related to, but somewhat exceeds, the content of the standard on sums of angles in triangles. Careful analysis of the angles requires students to construct valid arguments using abstract and quantitative reasoning. Producing the picture in part (c) helps students identify a common mathematical argument repeated multiple times. Students may use pattern blocks to develop the intuition for decomposing the hexagon into triangles. |

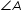 , in degrees, in the figure shown?
, in degrees, in the figure shown?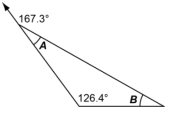
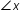 , in degrees, in the figure shown?
, in degrees, in the figure shown?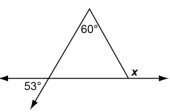
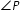 , in degrees?
, in degrees?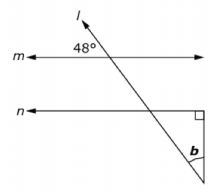
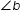 ?
?