General Information
Subject Area: Mathematics (B.E.S.T.)
Grade: 912
Strand: Data Analysis and Probability
Date Adopted or Revised: 08/20
Status: State Board Approved
Benchmark Instructional Guide
Connecting Benchmarks/Horizontal Alignment
Terms from the K-12 Glossary
- Data
- Population (in data analysis)
- Random sampling
- Association
- Repeated experiment
- Statistical question
Vertical Alignment
Previous Benchmarks
Next Benchmarks
Purpose and Instructional Strategies
In middle grades, students examined the processes for generating data and performing calculations from data. Students continued these processes to a degree in Algebra I. In Mathematics for College Statistics, students learn to compare and contrast surveys, observations, and experiments. This benchmark has a direct relationship to the benchmark MA.912.DP.5.6.- Instruction defines a survey to be the set of questions asked to participants. Surveys can
be deployed in an observation or an experiment.
- Students should have practice examining and comparing statistical studies; students
should examine how participants were selected or organized. If subjects are randomly
sampled and all asked the same set of questions, an observational study was utilized. If
the study was designed such that participants are randomly assigned to different groups
(at least two or more), an experimental study was utilized.
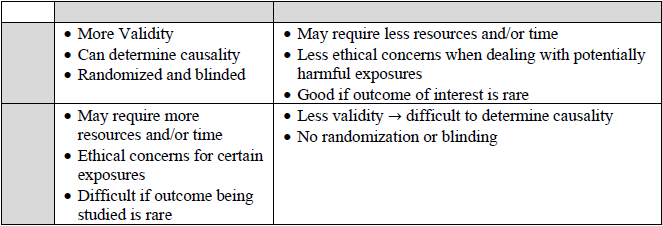
- For enrichment of this benchmark, different aspects can be discussed with experimental studies, including treatment groups, control groups, placebos, blinding and double-blinding.
- A single-blinded experiment is designed so that the participants are not aware of the treatment being administered in the study. A double-blinded experiment is designed so that participants and those collecting data are not aware of the treatment being administered in the study.
- As an expectation of this benchmark, students examine different types of studies and decide the conclusion that can be made.
- For example, if the study is experimental, it is possible to infer a cause and effect relationship between variables.
- If the study is observational, do not conclude a causal relationship. Instead, infer that there is a link or an association between variable.
- Instruction includes emphasizing that observational studies should include random sampling, while experimental studies should include random assignment among the various groups.
Common Misconceptions or Errors
- Students may initially make incorrect assumptions when determining if a study is an observation or experiment.
- Students may have difficulty deciding upon an appropriate conclusion from the statistical study.
- Students may believe experiments must use a placebo or have a control group.
Instructional Tasks
Instructional Task 1 (MTR.4.1, MTR.7.1)- Suppose a nutritionist is interested in the relationship between coffee consumption and a
person’s sleeping habits.
- Part A. Explain, in detail, how to design a statistical study that could possibly show a link between increased coffee consumption and a decrease in an individual’s average number of hours slept per night. Be sure to include the use of a survey and use proper statistical terminology.
- Part B. Explain, in detail, how to design a statistical study that could possibly show increased coffee consumption causes a decrease in an individual’s average number of hours slept per night. Be sure to include the use of a survey and use proper statistical terminology.
- Part C. Partner with a classmate to compare and contrast the designs of the two studies above.
Instructional Items
Instructional Item 1- Mr. Artemis is reviewing his students’ grades and their attendance over the course of the
school year. He notices that students who miss very few classes have higher grades, and that
as students miss more and more classes, their grades tend to decrease.
- Part A. Should Mr. Artemis conclude that missing fewer classes will cause students’ grades to increase? Explain why this is or is not an appropriate conclusion.
- Part B. Would it be possible (or ethical) to design an experimental study where Mr. Artemis could determine if missing fewer classes will cause students’ grades to increase? Explain.