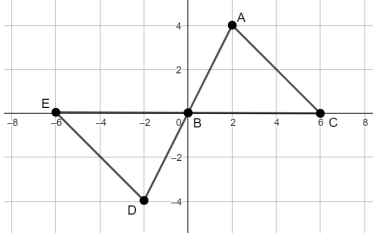
Apply rigid transformations to map one figure onto another to justify that the two figures are congruent.
: Instruction includes showing that the corresponding sides and the corresponding angles are congruent.
| Name |
Description |
| Justifying HL Congruence | Students are asked to use rigid motion to explain why the HL pattern of congruence ensures right triangle congruence. |
| Congruent Trapezoids | Students will determine whether two given trapezoids are congruent. |
| Transform This | Students are asked to translate and rotate a triangle in the coordinate plane and explain why the pre-image and image are congruent. |
| Proving the Alternate Interior Angles Theorem | In a diagram involving two parallel lines and a transversal, students are asked to use rigid motion to prove that alternate interior angles are congruent. |
| Showing Triangles Congruent Using Rigid Motion | Students are asked to use the definition of congruence in terms of rigid motion to show that two triangles are congruent in the coordinate plane. |
| Justifying SSS Congruence | Students are asked to use rigid motion to explain why the SSS pattern of congruence ensures triangle congruence. |
| Justifying SAS Congruence | Students are asked to use rigid motion to explain why the SAS pattern of congruence ensures triangle congruence. |
| Justifying ASA Congruence | Students are asked to use rigid motion to explain why the ASA pattern of congruence ensures triangle congruence. |
| Name |
Description |
| "Triangle Congruence Show" Starring Rigid Transformations | Students will be introduced to the definition of congruence in terms of rigid motion and use it to determine if two triangles are congruent. |
| Regular Polygon Transformation Investigation | This is an introductory lesson on regular polygon transformation for congruency using a hands-on approach. |
| Match That! | Students will prove that two figures are congruent based on a rigid motion(s) and then identify the corresponding parts using paragraph proof and vice versa, prove that two figures are congruent based on corresponding parts and then identify which rigid motion(s) map the images. |
| Rotations of Regular Polygons | This lesson guides students through the development of a formula to find the first angle of rotation of any regular polygon to map onto itself. Free rotation simulation tools such as GeoGebra, are used. |
| Turning to Congruence | This lesson uses rigid motions to prove the ASA and HL triangle congruence theorems. |
| Slip, Slide, Tip, and Turn: Corresponding Angles and Corresponding Sides | Using the definition of congruence in terms of rigid motion, students will show that two triangles are congruent. |
| Where Will I Land? | This is a beginning level lesson on predicting the effect of a series of reflections or a quick review of reflections for high school students. |
| Exploring Congruence Using Transformations | This is an exploratory lesson that elicits the relationship between the corresponding sides and corresponding angles of two congruent triangles. |
| How Much Proof Do We Need? | Students determine the minimum amount of information needed to prove that two triangles are similar. |
| How do your Air Jordans move? | In this inquiry lesson, students are moving their individually designed Air Jordans around the room to explore rigid transformations on their shoes. They will Predict-Observe-Explain the transformations and then have to explain their successes/failures to other students. |
| Triangles on a Lattice | In this activity, students will use a 3x3 square lattice to study transformations of triangles whose vertices are part of the lattice. The tasks include determining whether two triangles are congruent, which transformations connect two congruent triangles, and the number of non-congruent triangles (with vertices on the lattice) that are possible. |
| Congruence vs. Similarity | Students will learn the difference between congruence and similarity of classes of figures (such as circles, parallelograms) in terms of the number of variable lengths in the class. A third category will allow not only rigid motions and dilations, but also a single one-dimensional stretch, allowing more classes of figures to share sufficient common features to belong. |
| Let's Reflect On This... | Students will use parallel and intersecting lines on the coordinate plane to transform reflections into translations and rotations. |
| Transformers 3 | Students will learn the vocabulary of three rigid transformations, reflection, translation, and rotation, and how they relate to congruence. Students will practice transforming figures by applying each isometry and identifying which transformation was used on a figure. The teacher will assign students to take pictures of the three transformations found in the real world. |
| Name |
Description |
| Partitioning a Hexagon | The purpose of this task is for students to find a way to decompose a regular hexagon into congruent figures. This is meant as an instructional task that gives students some practice working with transformations. |
| Why Does ASA Work? | This problem solving task ask students to show the reflection of one triangle maps to another triangle. |
| When Does SSA Work to Determine Triangle Congruence? | In this problem, we considered SSA. The triangle congruence criteria, SSS, SAS, ASA, all require three pieces of information. It is interesting, however, that not all three pieces of information about sides and angles are sufficient to determine a triangle up to congruence. |
| Why Does SAS Work? | This problem solving task challenges students to explain the reason why the given triangles are congruent, and to construct reflections of the points. |
| Reflections and Isosceles Triangles | This activity uses rigid transformations of the plane to explore symmetries of classes of triangles. |
| Reflections and Equilateral Triangles | This activity is one in a series of tasks using rigid transformations of the plane to explore symmetries of classes of triangles, with this task in particular focusing on the class of equilaterial triangles |
| Reflected Triangles | This task asks students to use a straightedge and compass to construct the line across which a triangle is reflected. |
| Why does SSS work? | This particular problem solving task exhibits congruency between two triangles, demonstrating translation, reflection and rotation. |
| Building a tile pattern by reflecting octagons | This task applies reflections to a regular octagon to construct a pattern of four octagons enclosing a quadrilateral: the focus of the task is on using the properties of reflections to deduce that the quadrilateral is actually a square. |
| Building a tile pattern by reflecting hexagons | This task applies reflections to a regular hexagon to construct a pattern of six hexagons enclosing a seventh: the focus of the task is on using the properties of reflections to deduce this seven hexagon pattern. |
| Is This a Rectangle? | The goal of this task is to provide an opportunity for students to apply a wide range of ideas from geometry and algebra in order to show that a given quadrilateral is a rectangle. Creativity will be essential here as the only given information is the Cartesian coordinates of the quadrilateral's vertices. Using this information to show that the four angles are right angles will require some auxiliary constructions. Students will need ample time and, for some of the methods provided below, guidance. The reward of going through this task thoroughly should justify the effort because it provides students an opportunity to see multiple geometric and algebraic constructions unified to achieve a common purpose. The teacher may wish to have students first brainstorm for methods of showing that a quadrilateral is rectangle (before presenting them with the explicit coordinates of the rectangle for this problem): ideally, they can then divide into groups and get to work straightaway once presented with the coordinates of the quadrilateral for this problem. |
| Name |
Description |
| Partitioning a Hexagon: | The purpose of this task is for students to find a way to decompose a regular hexagon into congruent figures. This is meant as an instructional task that gives students some practice working with transformations. |
| Why Does ASA Work?: | This problem solving task ask students to show the reflection of one triangle maps to another triangle. |
| When Does SSA Work to Determine Triangle Congruence?: | In this problem, we considered SSA. The triangle congruence criteria, SSS, SAS, ASA, all require three pieces of information. It is interesting, however, that not all three pieces of information about sides and angles are sufficient to determine a triangle up to congruence. |
| Why Does SAS Work?: | This problem solving task challenges students to explain the reason why the given triangles are congruent, and to construct reflections of the points. |
| Reflections and Isosceles Triangles: | This activity uses rigid transformations of the plane to explore symmetries of classes of triangles. |
| Reflections and Equilateral Triangles: | This activity is one in a series of tasks using rigid transformations of the plane to explore symmetries of classes of triangles, with this task in particular focusing on the class of equilaterial triangles |
| Reflected Triangles: | This task asks students to use a straightedge and compass to construct the line across which a triangle is reflected. |
| Why does SSS work?: | This particular problem solving task exhibits congruency between two triangles, demonstrating translation, reflection and rotation. |
| Building a tile pattern by reflecting octagons: | This task applies reflections to a regular octagon to construct a pattern of four octagons enclosing a quadrilateral: the focus of the task is on using the properties of reflections to deduce that the quadrilateral is actually a square. |
| Building a tile pattern by reflecting hexagons: | This task applies reflections to a regular hexagon to construct a pattern of six hexagons enclosing a seventh: the focus of the task is on using the properties of reflections to deduce this seven hexagon pattern. |
| Is This a Rectangle?: | The goal of this task is to provide an opportunity for students to apply a wide range of ideas from geometry and algebra in order to show that a given quadrilateral is a rectangle. Creativity will be essential here as the only given information is the Cartesian coordinates of the quadrilateral's vertices. Using this information to show that the four angles are right angles will require some auxiliary constructions. Students will need ample time and, for some of the methods provided below, guidance. The reward of going through this task thoroughly should justify the effort because it provides students an opportunity to see multiple geometric and algebraic constructions unified to achieve a common purpose. The teacher may wish to have students first brainstorm for methods of showing that a quadrilateral is rectangle (before presenting them with the explicit coordinates of the rectangle for this problem): ideally, they can then divide into groups and get to work straightaway once presented with the coordinates of the quadrilateral for this problem. |
| Name |
Description |
| Partitioning a Hexagon: | The purpose of this task is for students to find a way to decompose a regular hexagon into congruent figures. This is meant as an instructional task that gives students some practice working with transformations. |
| Why Does ASA Work?: | This problem solving task ask students to show the reflection of one triangle maps to another triangle. |
| When Does SSA Work to Determine Triangle Congruence?: | In this problem, we considered SSA. The triangle congruence criteria, SSS, SAS, ASA, all require three pieces of information. It is interesting, however, that not all three pieces of information about sides and angles are sufficient to determine a triangle up to congruence. |
| Why Does SAS Work?: | This problem solving task challenges students to explain the reason why the given triangles are congruent, and to construct reflections of the points. |
| Reflections and Isosceles Triangles: | This activity uses rigid transformations of the plane to explore symmetries of classes of triangles. |
| Reflections and Equilateral Triangles: | This activity is one in a series of tasks using rigid transformations of the plane to explore symmetries of classes of triangles, with this task in particular focusing on the class of equilaterial triangles |
| Reflected Triangles: | This task asks students to use a straightedge and compass to construct the line across which a triangle is reflected. |
| Why does SSS work?: | This particular problem solving task exhibits congruency between two triangles, demonstrating translation, reflection and rotation. |
| Building a tile pattern by reflecting octagons: | This task applies reflections to a regular octagon to construct a pattern of four octagons enclosing a quadrilateral: the focus of the task is on using the properties of reflections to deduce that the quadrilateral is actually a square. |
| Building a tile pattern by reflecting hexagons: | This task applies reflections to a regular hexagon to construct a pattern of six hexagons enclosing a seventh: the focus of the task is on using the properties of reflections to deduce this seven hexagon pattern. |
| Is This a Rectangle?: | The goal of this task is to provide an opportunity for students to apply a wide range of ideas from geometry and algebra in order to show that a given quadrilateral is a rectangle. Creativity will be essential here as the only given information is the Cartesian coordinates of the quadrilateral's vertices. Using this information to show that the four angles are right angles will require some auxiliary constructions. Students will need ample time and, for some of the methods provided below, guidance. The reward of going through this task thoroughly should justify the effort because it provides students an opportunity to see multiple geometric and algebraic constructions unified to achieve a common purpose. The teacher may wish to have students first brainstorm for methods of showing that a quadrilateral is rectangle (before presenting them with the explicit coordinates of the rectangle for this problem): ideally, they can then divide into groups and get to work straightaway once presented with the coordinates of the quadrilateral for this problem. |