Standard #: MA.8.GR.2.1


This document was generated on CPALMS - www.cpalms.org



Given a preimage and image generated by a single transformation, identify the transformation that describes the relationship.


Clarifications


Clarification 1: Within this benchmark, transformations are limited to reflections, translations or rotations of images.

Clarification 2: Instruction focuses on the preservation of congruence so that a figure maps onto a copy of itself.



General Information

Subject Area: Mathematics (B.E.S.T.)
Grade: 8
Strand: Geometric Reasoning
Standard: Understand similarity and congruence using models and transformations.
Date Adopted or Revised: 08/20
Status: State Board Approved

Benchmark Instructional Guide

Terms from the K-12 Glossary

  • Congruent
  • Reflection
  • Rigid Transformation
  • Rotation
  • Translation

 

Vertical Alignment

Previous Benchmarks

Next Benchmarks

 

Purpose and Instructional Strategies

In grade 6, students identified the x- or y-axis as lines of reflection, and in grade 7, students solved problems involving scale drawings of geometric figures. In grade 8, students are introduced to the geometric transformations of reflection, dilation (scaling) and translation. In Algebra 1, students will describe transformations applied to functions. In Geometry, students will describe transformations given a preimage and an image and represent the transformation algebraically using coordinates. They will use transformations to justify congruence and similarity.
  • Informal language such as turns, flips, and slides can be used when exploring the concepts. As students transition, they should use formal mathematical language of rotations, reflections and translations. Students should have materials such as shapes cut from paper to model the transformations.
  • Instruction includes the use of real-world examples that don’t have to be a geometric figure.
    • For example, wallpaper, art, architecture and mirrors have images generated by simple transformations.
  • The work of transformations builds from students being able to visually see the images and developing a spatial understanding as the images move about the coordinate plane.
  • Transformations can be noted using the prime notation (′) for the image and its vertices. The preimage and its vertices will not have prime notation.
    • For example, the picture below showcases a single transformation.
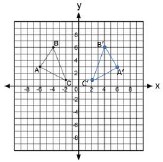
      single transformation.
  • Problem type include stating which direction, clockwise or counterclockwise, for rotations.
  • The expectation of this benchmark is not to represent a transformation on the coordinate plane as this will be included in MA.8.GR.2.3 instruction. During instruction, there should be flexibility moving from this benchmark to MA.8.GR.2.3 with each transformation which allows students to build conceptually prior to algorithmically.
  • For mastery of this benchmark, single transformations include one vertical translation or one horizontal translation. A vertical and horizontal translation would be considered two transformations.

 

Common Misconceptions or Errors

  • Students may incorrectly visualize the movement of a figure. To support instruction, students may need manipulatives such as tangrams and tessellations to help with physically moving the figures to understand the transformations.

 

Strategies to Support Tiered Instruction

  • Teacher supports instruction by using manipulatives such as tangrams and tessellations to help with physically moving the figures to understand the transformations.
  • Teacher models using geometric software and creates a graphic organizer to understand each transformation with relatable vocabulary.
  • Teacher uses example images and preimages to demonstrate the different types of transformation and how to identify images and preimages.
  • Teacher encourages the use of manipulatives and models counting the units moved to verify the proper movement of the transformation.
  • Instruction includes the use of tracing paper to trace the pre-image and explore possible transformations by slides, rotating, and flipping the image to try to reproduce the image.

 

Instructional Tasks

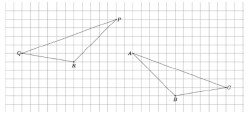
Instructional Task 1 (MTR.6.1)
Is it possible to have ΔPRQ make one translation, rotation, or reflection to become the image of ΔABC? Explain why or why it is not possible. Determine which transformation(s) may be used.
Two triangles

 

Instructional Items

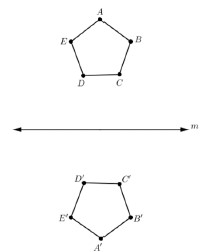
Instructional Item 1
Determine the transformation from the preimage above line m to the image below line m.
transformation from the preimage

Instructional Item 2
Draw a right triangle labeled with vertices MNO and then sketch the right triangle that has been rotated 90° counterclockwise.

 

*The strategies, tasks and items included in the B1G-M are examples and should not be considered comprehensive.


Related Courses

Course Number1111 Course Title222
1205050: M/J Accelerated Mathematics Grade 7 (Specifically in versions: 2014 - 2015, 2015 - 2020, 2020 - 2022, 2022 and beyond (current))
1205070: M/J Grade 8 Pre-Algebra (Specifically in versions: 2014 - 2015, 2015 - 2022, 2022 and beyond (current))
1204000: M/J Foundational Skills in Mathematics 6-8 (Specifically in versions: 2014 - 2015, 2015 - 2022, 2022 and beyond (current))
7812030: Access M/J Grade 8 Pre-Algebra (Specifically in versions: 2014 - 2015, 2015 - 2018, 2018 - 2019, 2019 - 2022, 2022 and beyond (current))


Related Access Points

Access Point Number Access Point Title
MA.8.GR.2.AP.1 Given two figures on a coordinate plane, identify if the image is translated, rotated or reflected.


Related Resources

Formative Assessments

Name Description
Define a Translation

Students are asked to develop a definition for translation.

Define a Rotation

Students are asked to develop a definition of rotation.

Define a Reflection

Students are asked to develop a definition of reflection.

Angle Transformations

Students are given the opportunity to experimentally verify the properties of angle transformations (translations, reflections, and rotations).

Segment Transformations

Students are given the opportunity to experimentally verify the properties of segment transformations (translations, reflections, and rotations).

Parallel Line Transformations

Students are given the opportunity to experimentally verify the properties of parallel line transformations (translations, reflections, and rotations).

Transformations of Rectangles and Squares

Students are asked to describe the rotations and reflections that carry a rectangle and a square onto itself.

Transformations of Parallelograms and Rhombi

Students are asked to describe the rotations and reflections that carry a parallelogram and rhombus onto itself.

Transformations of Trapezoids

Students are asked to describe the rotations and reflections that carry a trapezoid onto itself.

Transformations of Regular Polygons

Students are asked to describe the rotations and reflections that carry a regular polygon onto itself.

Lesson Plans

Name Description
Transformations in the Coordinate Plane

In this exploration activity of reflections, translations, and rotations, students are guided to discover general algebraic rules for special classes of transformations in the coordinate plane. This lesson is intended to be used after the development of formal definitions of rotations, translations, and reflections.

"Triangle Congruence Show" Starring Rigid Transformations

Students will be introduced to the definition of congruence in terms of rigid motion and use it to determine if two triangles are congruent.

Sequence of Transformations

This lesson will assist students in performing multi-step transformations. Students will follow a sequence of transformations on geometric figures using translations, reflections, and rotations.

Regular Polygon Transformation Investigation

This is an introductory lesson on regular polygon transformation for congruency using a hands-on approach.

Match That!

Students will prove that two figures are congruent based on a rigid motion(s) and then identify the corresponding parts using paragraph proof and vice versa, prove that two figures are congruent based on corresponding parts and then identify which rigid motion(s) map the images.

Isometries with GeoGebra

In this lesson, students explore transformations with GeoGebra and then apply concepts using a straightedge on paper. Students apply rules for each isometry. There is a teacher-driven opening followed by individual student activity.

How Did It Get There? A Series of Transformation Events

Students will perform a series of transformations in order to determine how the pre-image will map onto the final image of a given figure. Students will use patty paper to manipulate their pre-image onto the image. Students will also work in collaborative groups to discuss their findings and will have the opportunity to share their series of transformations with the class. The class discussion will be used to demonstrate that there are several ways for the students to map their pre-image onto the final image.

Dancing For Joy

We have danced our way through reflections, rotations, and translations; now we are ready to take it up a notch by performing a sequence of transformations. Students will also discover the results when reflecting over parallel lines versus intersecting lines.

Turning to Congruence

This lesson uses rigid motions to prove the ASA and HL triangle congruence theorems.

Product of Two Transformations

Students will identify a sequence of steps that will translate a pre-image to its image. Students will also demonstrate that the sequence of two transformations is not always commutative.

Slip, Slide, Tip, and Turn: Corresponding Angles and Corresponding Sides

Using the definition of congruence in terms of rigid motion, students will show that two triangles are congruent.

How to Land Your Spaceship

Teach your students to maneuver a "spaceship" through a sequence of transformations that will successfully carry it onto its landing pad. GeoGebra directons are provided.

Exploring Congruence Using Transformations

This is an exploratory lesson that elicits the relationship between the corresponding sides and corresponding angles of two congruent triangles.

How do your Air Jordans move?

In this inquiry lesson, students are moving their individually designed Air Jordans around the room to explore rigid transformations on their shoes. They will Predict-Observe-Explain the transformations and then have to explain their successes/failures to other students.

Triangles on a Lattice

In this activity, students will use a 3x3 square lattice to study transformations of triangles whose vertices are part of the lattice. The tasks include determining whether two triangles are congruent, which transformations connect two congruent triangles, and the number of non-congruent triangles (with vertices on the lattice) that are possible.

Reflections Hands On

Students will use a protractor/ruler to construct reflections and a composite of reflections. They will create transformations using paper cut-outs and a coordinate plane. For independent practice, students will predict and verify sequences of transformations. The teacher will need an LCD Projector and document camera.

A Transformation's Adventure with Patty Paper: Exploring Translations, Reflections and Rotations.

Students are introduced to isometric transformations using patty paper. Translations, reflections, and rotations will be explained and practiced, emphasizing the properties preserved during those transformations and, without sacrificing precision, allowing students to differentiate between these isometries. The lesson can also be taught using GeoGebra free software.

Let's Reflect On This...

Students will use parallel and intersecting lines on the coordinate plane to transform reflections into translations and rotations.

Dilation Transformation

Students identify dilations, verify that polygons are similar, and use the dilation rule to map dilations. Task cards are provided for independent practice. The PowerPoint also includes detailed illustrations for constructing a dilation using a compass and a straight edge.

Transformers 3

Students will learn the vocabulary of three rigid transformations, reflection, translation, and rotation, and how they relate to congruence. Students will practice transforming figures by applying each isometry and identifying which transformation was used on a figure. The teacher will assign students to take pictures of the three transformations found in the real world.

Rotation of Polygons about a Point

Students will rotate polygons of various shapes about a point. Degrees of rotation vary but generally increase in increments of 90 degrees. Points of rotation include points on the figure, the origin, and points on the coordinate plane. The concept of isometry is addressed.

Transform through the Maze

In this fun activity, students will use rigid transformations to move a triangle through a maze. The activity provides applications for both honors and standard levels. It requires students to perform rotations, translations, and reflections.

Problem-Solving Tasks

Name Description
Congruent Segments

Students' first experience with transformations is likely to be with specific shapes like triangles, quadrilaterals, circles, and figures with symmetry. Exhibiting a sequence of transformations that shows that two generic line segments of the same length are congruent is a good way for students to begin thinking about transformations in greater generality.

Congruent Triangles

This task has two goals: first to develop student understanding of rigid motions in the context of demonstrating congruence. Secondly, student knowledge of reflections is refined by considering the notion of orientation in part (b). Each time the plane is reflected about a line, this reverses the notions of ''clockwise'' and ''counterclockwise.''

Student Resources

Problem-Solving Tasks

Name Description
Congruent Segments:

Students' first experience with transformations is likely to be with specific shapes like triangles, quadrilaterals, circles, and figures with symmetry. Exhibiting a sequence of transformations that shows that two generic line segments of the same length are congruent is a good way for students to begin thinking about transformations in greater generality.

Congruent Triangles:

This task has two goals: first to develop student understanding of rigid motions in the context of demonstrating congruence. Secondly, student knowledge of reflections is refined by considering the notion of orientation in part (b). Each time the plane is reflected about a line, this reverses the notions of ''clockwise'' and ''counterclockwise.''



Parent Resources

Problem-Solving Tasks

Name Description
Congruent Segments:

Students' first experience with transformations is likely to be with specific shapes like triangles, quadrilaterals, circles, and figures with symmetry. Exhibiting a sequence of transformations that shows that two generic line segments of the same length are congruent is a good way for students to begin thinking about transformations in greater generality.

Congruent Triangles:

This task has two goals: first to develop student understanding of rigid motions in the context of demonstrating congruence. Secondly, student knowledge of reflections is refined by considering the notion of orientation in part (b). Each time the plane is reflected about a line, this reverses the notions of ''clockwise'' and ''counterclockwise.''



Printed On:5/1/2024 9:56:49 PM
Print Page | Close this window