Standard #: MA.8.AR.3.1


This document was generated on CPALMS - www.cpalms.org



Determine if a linear relationship is also a proportional relationship.


Clarifications


Clarification 1: Instruction focuses on the understanding that proportional relationships are linear relationships whose graph passes through the origin.

Clarification 2: Instruction includes the representation of relationships using tables, graphs, equations and written descriptions.



General Information

Subject Area: Mathematics (B.E.S.T.)
Grade: 8
Strand: Algebraic Reasoning
Standard: Extend understanding of proportional relationships to two-variable linear equations.
Date Adopted or Revised: 08/20
Status: State Board Approved

Benchmark Instructional Guide

Connecting Benchmarks/Horizontal Alignment

 

Terms from the K-12 Glossary

  • Constant of Proportionality
  • Proportional Relationship

 

Vertical Alignment

Previous Benchmarks

Next Benchmarks

 

Purpose and Instructional Strategies

In grade 7, students determined if two quantities are in a proportional relationship from a table and determined the constant of proportionality. In grade 8, students determine whether a given linear relationship is also a proportional relationship. In Algebra 1, students will use two-variable linear equations to represent mathematical or real-world contexts.
  • Instruction includes using a variety of variables to represent the slope, which is the same as the constant of proportionality when the linear relationship is also a proportional relationship. Students used p or k in grade 7 to represent the constant of proportionality and now in grade 8 may use m to represent slope. Students should understand that the slope or constant rate of change can be represented by any variable.
  • Instruction includes students graphing relationships and writing equations to determine if two linearly related quantities are also in a proportional. Students need to be provided examples to show evidence that not all linear relationships are proportional.
  • Students should connect unit rates, the constant of proportionality and slope in order to represent similar ideas in different contexts.

 

Common Misconceptions or Errors

  • Students may incorrectly state a relationship is not proportional if the origin is not visible in the graph or given in the table.
  • Students may incorrectly think all linear relationships are proportional. Some students find a constant rate of change and confuse this with a constant ratio. Help students understand that a constant ratio is only possible if the relationship passes through the origin.

 

Strategies to Support Tiered Instruction

  • Instruction includes providing opportunities to explore relationships represented on graphs and in tables that do not include the origin. Students should determine the rate of change in these situations and use ratio reasoning to determine if the relationships pass through the origin or not.
  • Instruction includes the use of geometric software to visually compare proportional and non-proportional graphs to model that all linear graphs are not proportional relationships.
  • Teacher co-creates a graphic organizer to represent the similarities and differences of the terms: unit rate, constant of proportionality, and slope. Specifically include the different contexts applicable to each.

 

Instructional Tasks

Instructional Task 1 (MTR.4.1, MTR.6.1, MTR.7.1)
A student is making trail mix for each serving size in the table given.
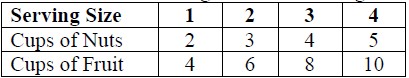
Table
  • Part A. Is this relationship linear? If so, state the constant rate of change.
  • Part B. Determine if this relationship is also proportional. 
  • Part C. What do you notice about the number of cups of nuts and fruit that would be in a serving size of zero? Discuss with a partner.

Instructional Task 2 (MTR.4.1)
Part A. Write the two-variable equation that represents each graph.
Part B. Which graph represents a proportional relationship? Justify your answer.
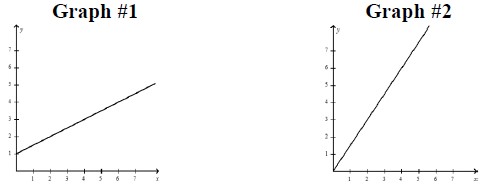
Graph

 

Instructional Items

Instructional Item 1
Alexia earns $14.75 an hour as a hostess at a local restaurant. She earns an additional $30 in tips each night from take-out orders. Determine if this linear relationship is proportional.

Instructional Item 2
The circumference of a circle is proportional to its diameter. This relationship can be expressed by the equation C = πd. Determine if this linear relationship is proportional.

 

*The strategies, tasks and items included in the B1G-M are examples and should not be considered comprehensive.



Related Courses

Course Number1111 Course Title222
1205050: M/J Accelerated Mathematics Grade 7 (Specifically in versions: 2014 - 2015, 2015 - 2020, 2020 - 2022, 2022 and beyond (current))
1205070: M/J Grade 8 Pre-Algebra (Specifically in versions: 2014 - 2015, 2015 - 2022, 2022 and beyond (current))
1204000: M/J Foundational Skills in Mathematics 6-8 (Specifically in versions: 2014 - 2015, 2015 - 2022, 2022 and beyond (current))
7812030: Access M/J Grade 8 Pre-Algebra (Specifically in versions: 2014 - 2015, 2015 - 2018, 2018 - 2019, 2019 - 2022, 2022 and beyond (current))


Related Access Points

Access Point Number Access Point Title
MA.8.AR.3.AP.1 Given a table, a graph, or equation, determine whether a linear relationship is proportional.


Related Resources

Lesson Plans

Name Description
The Linear Function Connection

The students will compare two linear functions that have been represented in different ways (equation, table, graph, verbal description). They will be able to find and compare the rate of change, or slope, of the function from any of the representations.

Determining the density of regular and irregular objects

This MEA provides students with opportunities to practice solving one-step equations while learning about density. Students will calculate density of regular and irregular objects.

Model Eliciting Activities, MEAs, are open-ended, interdisciplinary problem-solving activities that are meant to reveal students’ thinking about the concepts embedded in realistic situations. Click here to learn more about MEAs and how they can transform your classroom.

Perspectives Video: Teaching Idea

Name Description
Solving Quadratic Equations by Completing the Square

Unlock an effective teaching strategy for teaching solving quadratic equations by completing the square in this Teacher Perspectives video for educators.

Problem-Solving Tasks

Name Description
Sore Throats, Variation 2

Students graph proportional relationships and understand the unit rate as a measure of the steepness of the related line, called the slope. Students will also treat slopes more formally when they graph proportional relationships and interpret the unit rate as the slope of the graph.

Who Has the Best Job?

This task asks the student to graph and compare two proportional relationships and interpret the unit rate as the slope of the graph. Students are also asked to write an equation and graph each scenario.

Comparing Speeds in Graphs and Equations

This task provides the opportunity for students to reason about graphs, slopes, and rates without having a scale on the axes or an equation to represent the graphs. Students who prefer to work with specific numbers can write in scales on the axes to help them get started.

Peaches and Plums

This task asks students to reason about the relative costs per pound of two fruits without actually knowing what the costs are. Students who find this difficult may add a scale to the graph and reason about the meanings of the ordered pairs. Comparing the two approaches in a class discussion can be a profitable way to help students make sense of slope.

Student Resources

Problem-Solving Tasks

Name Description
Who Has the Best Job?:

This task asks the student to graph and compare two proportional relationships and interpret the unit rate as the slope of the graph. Students are also asked to write an equation and graph each scenario.

Comparing Speeds in Graphs and Equations:

This task provides the opportunity for students to reason about graphs, slopes, and rates without having a scale on the axes or an equation to represent the graphs. Students who prefer to work with specific numbers can write in scales on the axes to help them get started.

Peaches and Plums:

This task asks students to reason about the relative costs per pound of two fruits without actually knowing what the costs are. Students who find this difficult may add a scale to the graph and reason about the meanings of the ordered pairs. Comparing the two approaches in a class discussion can be a profitable way to help students make sense of slope.



Parent Resources

Problem-Solving Tasks

Name Description
Sore Throats, Variation 2:

Students graph proportional relationships and understand the unit rate as a measure of the steepness of the related line, called the slope. Students will also treat slopes more formally when they graph proportional relationships and interpret the unit rate as the slope of the graph.

Who Has the Best Job?:

This task asks the student to graph and compare two proportional relationships and interpret the unit rate as the slope of the graph. Students are also asked to write an equation and graph each scenario.

Comparing Speeds in Graphs and Equations:

This task provides the opportunity for students to reason about graphs, slopes, and rates without having a scale on the axes or an equation to represent the graphs. Students who prefer to work with specific numbers can write in scales on the axes to help them get started.

Peaches and Plums:

This task asks students to reason about the relative costs per pound of two fruits without actually knowing what the costs are. Students who find this difficult may add a scale to the graph and reason about the meanings of the ordered pairs. Comparing the two approaches in a class discussion can be a profitable way to help students make sense of slope.



Printed On:4/23/2024 4:11:45 PM
Print Page | Close this window