Standard #: MA.7.DP.2.3

Benchmark Instructional Guide

Connecting Benchmarks/Horizontal Alignment

• MA.7.NSO.1.2

 

Terms from the K-12 Glossary

• Event
• Theoretical Probability

 

Vertical Alignment

Previous Benchmarks

• MA.6.NSO.3.5

Next Benchmarks

• MA.8.DP.2.1
• MA.8.DP.2.2
• MA.8.DP.2.3

 

Purpose and Instructional Strategies

In grade 7, students find the theoretical probability of an event related to a simple experiment, and in grade 8, they will find the theoretical probability of an event related to a repeated experiment. 

• Instruction builds on finding sample spaces from MA.7.DP.2.1. Have students discuss their understanding of the words “theoretical” and “probability” to build toward a formal definition of theoretical probability.
• When finding theoretical probability, have students work from their sample space. Doing so will lead to the understanding that since experiments for this benchmark are fair, the probability of an event is equivalent to $\frac{\text{<i>number of outcomes in the event</i>}}{\text{<i>number of outcomes in the sample space</i>}}$.
  • For example, if rolling a fair 6-sided die, the sample space is {1, 2, 3, 4, 5, 6,}. If one wants to find $P$($rolling\mathrm{ a}n\mathrm{ o}dd\mathrm{ n}umber$), students can circle all of the odd numbers from the sample space to determine the probability as , or 0.5.
• While the benchmark does focus on fair experiments, instruction could include spinners with unequal sections making the connection to angle measures and to circle graphs.
• Instruction focuses on the simple experiments listed in Clarification 2.
  • For example, when tossing a coin with one side colored yellow and the other side colored red, $P$($landing on blue$) = 0.
  • For example, when rolling a 10-sided die, $P$($no\mathrm{t }rollin\mathrm{g }a\mathrm{ m}ultiple\mathrm{ o}f$ 3) = 0.7.
  • For example, when picking a card from a deck that contains each of the letters of the alphabet, $P$($picking a constant$) = $\frac{\text{21}}{\text{26}}$.
  • For example, when picking a tiles from a bag that contains a set of chess pieces, $P$($picking a pawn$) = 50%.
  • For example, when spinning a spinner that contains 5 sections where two of the sections are green and the remaining sections are red, white and blue,
    $P$($landing on a color from the American flag$) = $\frac{\text{3}}{\text{5}}$.

 

Common Misconceptions or Errors

• Students may incorrectly convert forms of probability between fractions and percentages. To address this misconception, scaffold with more familiar values initially to facilitate the interpretation.
• Students may incorrectly count outcomes when one outcome appears more than once in the sample space.
  • For example, if the sample space is {red, red, blue} and one wants to find $P$($red$), a student may incorrectly state $\frac{\text{1}}{\text{2}}$ or $\frac{\text{1}}{\text{3}}$ instead of $\frac{\text{2}}{\text{3}}$.

 

Strategies to Support Tiered Instruction

• Teacher provides instruction in converting between fractions and percentages, by using more familiar values.
  • For example, $\frac{\text{1}}{\text{2}}$ = 50%, $\frac{\text{1}}{\text{4}}$ = 25%, $\frac{\text{1}}{\text{3}}$ ≈ 33.3%, etc.
• Teacher co-creates a T-chart (like the one below) to list the experiment and the sample space necessary for the examples provided.
  
  
  

 

Instructional Tasks

Instructional Task 1 (MTR.4.1, MTR.7.1)
Look at the shirt you are wearing today and determine how many buttons it has. Then complete the following table for all the members of your class.
Suppose each student writes his or her name on an index card, and one card is selected randomly.

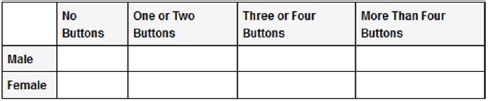
• Part A. What is the probability that the student whose card is selected is wearing a shirt with no buttons?
• Part B. What is the probability that the student whose card is selected is female and is wearing a shirt with two or fewer buttons?

Instructional Task 2 (MTR.4.1, MTR.5.1)
There is only one question on the next quiz and it will be true or false.

• Part A. If a student randomly answers the question, what is the probability of earning a score of 100%?
• Part B. What is the probability of earning a 50%?

 

Instructional Items

Instructional Item 1
What is the probability of choosing a 9 from a standard deck of 52 cards?

Instructional Item 2
There are 7 red, 5 blue and 12 green marbles in a bag.

• Part A. What is the probability of choosing a red marble?
• Part B. What is the probability of not choosing a green marble?
• Part C. What is the probability of choosing a yellow marble?
• Part D. What is the probability of choosing a red or blue marble?

 

*The strategies, tasks and items included in the B1G-M are examples and should not be considered comprehensive.