Standard #: MA.7.DP.2.2

Benchmark Instructional Guide

Connecting Benchmarks/Horizontal Alignment

• MA.7.NSO.1.2

 

Terms from the K-12 Glossary

• Event
• Theoretical Probability

 

Vertical Alignment

Previous Benchmarks

• MA.6.NSO.3.5

Next Benchmarks

• MA.8.DP.2.2
• MA.8.DP.2.3

 

Purpose and Instructional Strategies

In grade 7, students interpret the probability of a chance event and the likelihood of it occurring. In grade 8, students will solve problems involving probabilities related to single or repeated experiments, including making predictions based on theoretical probability. 

• An event is a set of outcomes.
  • For example, if the experiment is to roll a six-sided die, possible events could be:
    • “rolling a 3 or a 4;”
    • “rolling an even number;” or
    • “not rolling a 2.”
• Instruction includes the understanding that some events can have a probability of 1 or 0. Students should understand that if an event has a probability of zero, the event is impossible or will not occur. If an event has a probability of one, the event is certain or must occur.
  • For example, in the experiment of rolling a 6-sided die, the event of rolling a 1, 2, 3, 4, 5 or 6 would have a probability of 1.
  • For example, when rolling a 6-sided fair die, the event of rolling a 7 would have a probability of 0.
• Instruction includes having students use probabilities of 1, 0.5 and 0 as benchmark probabilities to interpret the likelihood of other events.
  • For example, if a student wants to interpret the likelihood represented by the probability of 80%, they can compare 80% to the benchmark probabilities of 50% and 100%.
• If an event has a probability of 0.5, it can be interpreted that is has the same likelihood as its opposite.
  • For example, in the experiment of picking a card from a standard 52-card deck, the event of picking a red card has a probability of 0.5, which can be interpreted as having the same likelihood as the opposite event, which is picking a black card.

 

Common Misconceptions or Errors

• Students may invert the meaning of an event and an experiment.
• Students may confuse the mathematical meaning of a word like “event” with the everyday meaning.
• Students may incorrectly convert forms of probability between fractions and percentages. To address this misconception, scaffold with more familiar values initially to facilitate the interpretation.
• Students may incorrectly interpret a value with a negative sign as a possible probability.
  • For example, −$\frac{\text{1}}{\text{2}}$ cannot represent a probability since negative values are less than 0.

 

Strategies to Support Tiered Instruction

• Teacher creates and posts an anchor chart with visual representations of probability terms to assist students in correct academic vocabulary when solving real-world problems.
• Teacher provides opportunities for students to use a 100 frame to review place value for and the connections to decimal, fractional and percentage forms of probabilities.
• Instruction includes the use of a 100 frame to review place value for tenths, hundredths, and if needed, thousandths and the connections for decimal and fractional forms of probabilities.
• When students incorrectly convert from one form to another (i.e, fraction to percentage), the teacher scaffolds with more familiar values initially to facilitate the interpretation.

 

Instructional Tasks

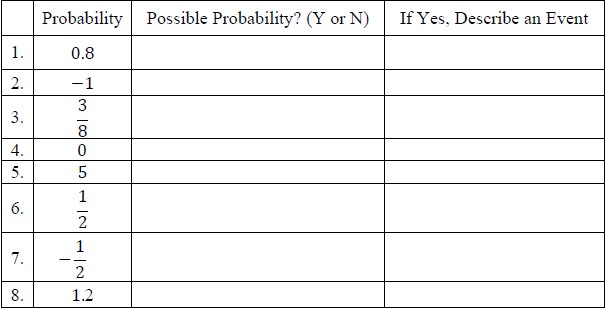
Instructional Task 1 (MTR.1.1)
Determine which of the following could represent the probability of an event. For those that can, provide a possible event that would fit the probability given.

 

Instructional Items

Instructional Item 1
In each scenario, a probability is given. Describe each event as likely, unlikely or neither.