Standard #: MA.7.GR.1.3


This document was generated on CPALMS - www.cpalms.org



Explore the proportional relationship between circumferences and diameters of circles. Apply a formula for the circumference of a circle to solve mathematical and real-world problems.


Clarifications


Clarification 1: Instruction includes the exploration and analysis of circular objects to examine the proportional relationship between circumference and diameter and arrive at an approximation of pi (π) as the constant of proportionality.

Clarification 2: Solutions may be represented in terms of pi (π) or approximately.



General Information

Subject Area: Mathematics (B.E.S.T.)
Grade: 7
Strand: Geometric Reasoning
Standard: Solve problems involving two-dimensional figures, including circles.
Date Adopted or Revised: 08/20
Status: State Board Approved

Benchmark Instructional Guide

Connecting Benchmarks/Horizontal Alignment

 

Terms from the K-12 Glossary

  • Circumference
  • Constant of Proportionality
  • Diameter
  • Pi (π)
  • Proportional Relationship

 

Vertical Alignment

Previous Benchmarks

Next Benchmarks

 

Purpose and Instructional Strategies

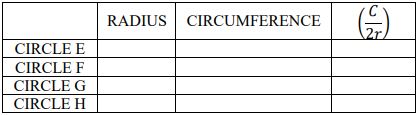
In grade 6, students solved problems involving the perimeter and area of two-dimensional figures. In grade 7, students explore the proportional relationship between circumferences and diameters of circles and develop and learn a formula to solve circumference problems. In grade 8, students will learn and use the Pythagorean Theorem to find the distance between points in the coordinate plane, and this builds the foundation for the equation of a circle in high school geometry. 
  • Instruction includes opportunities for students to see circular or cylindrical household objects of different sizes. Students will measure the diameter and the circumference of the circle in each object to the nearest tenth of a centimeter to arrive at an approximation of pi (π) as the constant of proportionality. Students can record the values in a table and plot the points on a coordinate plane to discover the pattern that arises (MTR.5.1). Students should complete multiple trials to best support their conclusions using both radius and diameter.
    Trial #1
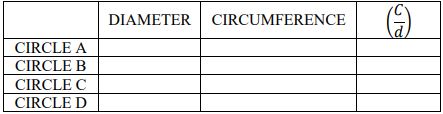
    Table
    Trial #2
    Table
  • Instruction emphasizes the relationship between radius and diameter so students will easily move between the equivalent forms of the circumference formula (MTR.3.1).
  • Instruction includes student understanding that circumference of a circle is the same as perimeter of any other two-dimensional figure.
  • Students are expected to know approximations of pi (355113, 227 or 3.14).

 

Common Misconceptions or Errors

  • Students may invert the terms radius and diameter. To address this misconception, review parts of a circle including radii, diameters and chords.
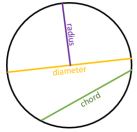
    Circle with radius, diameter and chord
  • Students may incorrectly believe pi is a variable, rather than a constant for every circle.
  • Students may confuse circumference and area. To address this misconception, help students connect circumference as perimeter of a circle.

 

Strategies to Support Tiered Instruction

  • Teacher provides opportunities for students to measure the radius and diameter of various circles and to explore and discuss the similarities and differences between radius and diameter.
  • To clarify misconceptions between the relationship of the diameter and circumference, instruction includes solving for the constant of proportionality between a given diameter and circumference of a circle and discussing the patterns that arise. Teacher provides opportunities for students to solve for the circumference of a given circle in terms of pi before replacing the value of pi with an approximation to determine the estimated circumference.
  • Teacher co-constructs a graphic organizer with students containing color-coded examples of circumference, area, diameter and radius.

 

Instructional Tasks

Instructional Task 1 (MTR.4.1, MTR.7.1)
Amy and Eunice are participating in a bike-a-thon this weekend. Amy has 29-inch road bike wheels and Eunice has 26-inch mountain bike wheels, where the bike wheel measurements are based on their diameter.
  • Part A. If they choose a bike-a-thon distance of 5 miles, whose bike wheels will need to do the fewest revolutions to reach the finish line?
  • Part B. How many more revolutions will the other bike need to make to reach the finish line? Explain your reasoning.

 

Instructional Items

Instructional Item 1
Determine the circumference of the following circles.
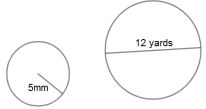
circles with radius 5mm, and diameter 12 yards

Instructional Item 2
When baking an apple pie, a strip of aluminum foil needs to be placed around the edge of the crust until the last 20 minutes of baking so that it will not burn. If using a 912-inch diameter pie pan, how long should the strip of foil be?

 

*The strategies, tasks and items included in the B1G-M are examples and should not be considered comprehensive.


Related Courses

Course Number1111 Course Title222
1205040: M/J Grade 7 Mathematics (Specifically in versions: 2014 - 2015, 2015 - 2022, 2022 and beyond (current))
1205050: M/J Accelerated Mathematics Grade 7 (Specifically in versions: 2014 - 2015, 2015 - 2020, 2020 - 2022, 2022 and beyond (current))
1204000: M/J Foundational Skills in Mathematics 6-8 (Specifically in versions: 2014 - 2015, 2015 - 2022, 2022 and beyond (current))
7812020: Access M/J Grade 7 Mathematics (Specifically in versions: 2014 - 2015, 2015 - 2018, 2018 - 2019, 2019 - 2022, 2022 and beyond (current))


Related Access Points

Access Point Number Access Point Title
MA.7.GR.1.AP.3 Apply a given formula for the circumference of a circle to solve mathematical problems.


Related Resources

Formative Assessments

Name Description
The Meaning of Pi

Students are asked to explain the relationship between the circumference and diameter of a circle in terms of pi.

Eye on Circumference

Students are asked to solve a problem involving the circumference of a circle.

Circumference Formula

Students are asked to write the formula for the circumference of a circle, explain what each symbol represents, and label the variables on a diagram.

Lesson Plans

Name Description
How Fast Can One Travel on a Bicycle?

Students investigate how the pedal and rear wheel gears affect the speed of a bicycle. A GeoGebra sketch is included that allows a simulation of the turning of the pedal and the rear wheel. A key goal is to provide an experience for the students to apply and integrate the key concepts in seventh-grade mathematics in a familiar context.

Circumference/Rotation Relationship in LEGO/NXT Robots or Do I Wheely need to learn this?

7th grade math/science lesson plan that focuses on the concept of circumference and rotation relationship. Culminates in a problem-solving exercise where students apply their knowledge to the "rotations" field in programming a LEGO/NXT robot to traverse a set distance.

Original Student Tutorials

Name Description
Pizza Pi: Circumference

Explore the origins of Pi as the ratio of Circumference to diameter of a circle. In this interactive tutorial you'll work with the circumference formula to determine the circumference of a circle and work backwards to determine the diameter and radius of a circle.

Swimming in Circles

Learn to solve problems involving the circumference and area of circle-shaped pools in this interactive tutorial.

Perspectives Video: Experts

Name Description
B.E.S.T. Journey

What roles do exploration, procedural reliability, automaticity, and procedural fluency play in a student's journey through the B.E.S.T. benchmarks? Dr. Lawrence Gray explains the path through the B.E.S.T. maththematics benchmarks in this Expert Perspectives video.

Practical Use of Area and Circumference

A math teacher describes the relationship between area and circumference and gives examples in nature.

Perspectives Video: Professional/Enthusiasts

Name Description
Pizza Pi: Area, Circumference & Unit Rate

How many times larger is the area of a large pizza compared to a small pizza? Which pizza is the better deal? Michael McKinnon of Gaines Street Pies talks about how the area, circumference and price per square inch is different depending on the size of the pizza.

Download the CPALMS Perspectives video student note taking guide.

Using Geometry for Interior Design and Architecture

An architect discusses how he uses circumference and area calculations to accurately create designs and plans.

Geometry for Dance Costume Designs

A dance costume designer describes how she uses circumference and area calculations to make clothing for the stage.

Perspectives Video: Teaching Ideas

Name Description
Cookies and Pi

A math teacher presents an idea for a classroom activity to engage students in measuring diameter and circumference to calculate pi.

Bicycle Mathematics: Speed and Distance Calculations

Cycling involves a lot of real-time math when you use an on-board computer. Learn about lesson ideas and how computers help with understanding performance.

Download the CPALMS Perspectives video student note taking guide.

Robot Mathematics: Gearing Ratio Calculations for Performance

A science teacher demonstrates stepwise calculations involving multiple variables for designing robots with desired characteristics.

Problem-Solving Tasks

Name Description
Running around a track II

The goal of this task is to model a familiar object, an Olympic track, using geometric shapes. Calculations of perimeters of these shapes explain the staggered start of runners in a 400 meter race.

Running around a track I

In this problem, geometry is applied to a 400 meter track to find the perimeter of the track.

Paper Clip

In this task, a typographic grid system serves as the background for a standard paper clip. A metric measurement scale is drawn across the bottom of the grid and the paper clip extends in both directions slightly beyond the grid. Students are given the approximate length of the paper clip and determine the number of like paper clips made from a given length of wire.

Tutorials

Name Description
Impact of a Radius Change on the Area of a Circle

This video shows how the area and circumference relate to each other and how changing the radius of a circle affects the area and circumference.

 

Circles: Radius, Diameter, Circumference, and Pi

In this video, students are shown the parts of a circle and how the radius, diameter, circumference and Pi relate to each other.

Circumference of a Circle

This video shows how to find the circumference, the distance around a circle, given the area.

Student Resources

Original Student Tutorials

Name Description
Pizza Pi: Circumference:

Explore the origins of Pi as the ratio of Circumference to diameter of a circle. In this interactive tutorial you'll work with the circumference formula to determine the circumference of a circle and work backwards to determine the diameter and radius of a circle.

Swimming in Circles:

Learn to solve problems involving the circumference and area of circle-shaped pools in this interactive tutorial.

Perspectives Video: Expert

Name Description
Practical Use of Area and Circumference:

A math teacher describes the relationship between area and circumference and gives examples in nature.

Problem-Solving Tasks

Name Description
Running around a track II:

The goal of this task is to model a familiar object, an Olympic track, using geometric shapes. Calculations of perimeters of these shapes explain the staggered start of runners in a 400 meter race.

Running around a track I:

In this problem, geometry is applied to a 400 meter track to find the perimeter of the track.

Paper Clip:

In this task, a typographic grid system serves as the background for a standard paper clip. A metric measurement scale is drawn across the bottom of the grid and the paper clip extends in both directions slightly beyond the grid. Students are given the approximate length of the paper clip and determine the number of like paper clips made from a given length of wire.

Tutorials

Name Description
Impact of a Radius Change on the Area of a Circle:

This video shows how the area and circumference relate to each other and how changing the radius of a circle affects the area and circumference.

 

Circles: Radius, Diameter, Circumference, and Pi:

In this video, students are shown the parts of a circle and how the radius, diameter, circumference and Pi relate to each other.

Circumference of a Circle:

This video shows how to find the circumference, the distance around a circle, given the area.



Parent Resources

Perspectives Video: Expert

Name Description
Practical Use of Area and Circumference:

A math teacher describes the relationship between area and circumference and gives examples in nature.

Problem-Solving Tasks

Name Description
Running around a track II:

The goal of this task is to model a familiar object, an Olympic track, using geometric shapes. Calculations of perimeters of these shapes explain the staggered start of runners in a 400 meter race.

Running around a track I:

In this problem, geometry is applied to a 400 meter track to find the perimeter of the track.

Paper Clip:

In this task, a typographic grid system serves as the background for a standard paper clip. A metric measurement scale is drawn across the bottom of the grid and the paper clip extends in both directions slightly beyond the grid. Students are given the approximate length of the paper clip and determine the number of like paper clips made from a given length of wire.



Printed On:4/16/2024 7:30:22 AM
Print Page | Close this window