Standard #: MA.6.GR.2.2

Benchmark Instructional Guide

Connecting Benchmarks/Horizontal Alignment

• MA.6.NSO.2.1
• MA.6.NSO.2.2
• MA.6.NSO.2.3
• MA.6.AR.1.1

 

Terms from the K-12 Glossary

• Algorithm
• Area
• Rectangle
• Quadrilateral
• Triangle

 

Vertical Alignment

Previous Benchmarks

• MA.5.GR.2.1

Next Benchmarks

• MA.7.GR.1.2

 

Purpose and Instructional Strategies

Students will use their understanding from grade 5 of finding the perimeter and area of rectangles with fractional or decimal sides to finding the areas of quadrilaterals and composite figures with positive rational number side lengths in grade 6 (MTR.1.1, MTR.2.1). Students will extend this knowledge in grade 7 to decompose composite figures into triangles and quadrilaterals in order to find area. 

• Instruction includes finding missing dimensions with quadrilaterals and composite figures (MTR.1.1, MTR.5.1).
• Instruction includes representing measurements for area as square units, units squared or units2.
• When using rational numbers, instruction is restricted to numbers within the same form. Students should not be penalized though if they convert from one form to another when performing operations.
  • For example, if students are working with fractions, the side lengths will not include decimals. If students are working with decimals, the side lengths will not include fractions.
• Students should look for opportunities to either decompose or compose shapes to enhance their geometric reasoning.
  • For example, in the diagram below, students can solve by decomposing or by composing.
    
    
    
    Area = (18·7)− $\frac{\text{1}}{\text{2}}$(6·7) = 105 cm²
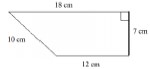
    Area = (12·7)+ $\frac{\text{1}}{\text{2}}$(6·7) = 105 cm²
• Problem types include having students measure lengths using a ruler to determine the area.

 

Common Misconceptions or Errors

• Students may invert the perimeter and area formulas.
• Students may incorrectly label all sides of the figure.
• Students may incorrectly identify a side of a triangle as the height.

 

Strategies to Support Tiered Instruction

• Teacher reviews the definitions of surface area and volume, and co-creates an anchor chart to display in the room explaining each. Providing flash cards or cue cards with the formulas will help students in place of anchor charts when they are outside the classroom area.
• Teacher models the use of different color markers or pencils to match similar sides when decomposing figures. This will help student accurately label the sides of each shape.
• Use manipulatives that students can measure to better understand there is a difference between a side length and the height in non-right triangles.
• Teacher models the use of manipulatives and geometric software to review the concept of perimeter or area.
• Teacher models the use of manipulatives shapes to reinforce the sides of the pieces that make up a decomposed figure.
• Teacher models the use of manipulatives that students can measure to better understand there is a difference between a side length and the height in non-right triangles.

 

Instructional Tasks

Instructional Task 1 (MTR.1.1, MTR.2.1, MTR.5.1)
The diagram shows the dimensions, in feet, of the local playground. While playing with friends, Shona lost the key to her diary somewhere in the dirt. By composing or decomposing into rectangles, determine the maximum number of square feet that Shona may need to search to find the missing key? If she only searched one rectangular area, what is the least number of square feet Shona searched?

 

Instructional Items

Instructional Item 1
A pentagon is shown. What is the area, in square inches, of the pentagon? Image not to scale.
Instructional Item 2
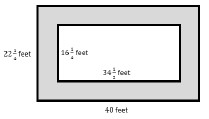
Mr. Moretti wants to cover the walkway around his swimming pool with tile. Determine how many square feet of tile he will need to cover the shaded portion of the diagram.

 

 

*The strategies, tasks and items included in the B1G-M are examples and should not be considered comprehensive.