Standard #: MA.6.NSO.1.1

Benchmark Instructional Guide

Connecting Benchmarks/Horizontal Alignment

• MA.6.NSO.3.5
• MA.6.NSO.4.1
• MA.6.NSO.4.2
• MA.6.AR.1.2
• MA.6.AR.3.1
• MA.6.AR.3.5
• MA.6.GR.1.1
• MA.6.GR.1.2
• MA.6.GR.1.3
• MA.6.DP.1.5 

 

Terms from the K-12 Glossary

• Integers
• Number Line
• Rational Number
• Whole Number 

 

Vertical Alignment

Previous Benchmarks

• MA.5.NSO.1.4  

http://flbt5.floridaearlylearning.com/standards.html 

Next Benchmarks

• MA.7.NSO.1.2
• MA.8.NSO.1.1
• MA.8.NSO.1.2 

 

Purpose and Instructional Strategies

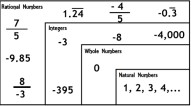
In grade 4, students plotted, ordered and compared fractions, including mixed numbers and fractions greater than one, with different numerators and different denominators. In grade 5, students plotted, ordered and compared multi-digit numbers with decimals up to the thousandths. In grade 6, students plot, order and compare on both sides of zero on the number line with all forms of rational numbers. Additionally, students will rewrite positive rational numbers in different but equivalent forms, which will extend to all rational numbers, including repeating decimals, in grade 7. In grade 8, students will plot, order and compare rational and irrational numbers. 

• Instruction builds on the understanding of numbers from elementary grades to include rational numbers. Students should understand that rational numbers are part of a larger number system, the real numbers, and that numbers from previous learning are all rational numbers. The graphic below explains the types of numbers that make up the rational number system.

• Inequality is a comparison between two values. Students should connect the words to the symbols, for example, the symbol < reads “is less than” and the symbol > reads “is greater than.” 
• It can be helpful for students to think about placement of rational numbers on a number line using benchmark comparison values.  
• • Examples of this could include the following questions: Between what two whole numbers does the rational number fall? Is it more than half or less than half way to the next whole number? 
• Within the grade 6 benchmarks, students are only expected to convert between forms of positive rational numbers. When working with negative rational numbers, students are only expected to determine their relationship based on relative location to each other, as can be demonstrated on a number line. 
• Having students read the comparison relationships from right to left and from left to right can help students develop flexibility of thinking and fluency with using the inequality symbols. 
• When comparing negative rational numbers, it is helpful to reason through the comparisons using real-world scenarios (MTR.7.1). 
  • For example, if a person owes $12.47 and another person owes $6.50, most students can quickly see and understand that −12.47 < −6.50 because the person owes more money would have less money. 
• Students should be given opportunities to order rational numbers by notating as a list (as is often done with data sets) and by notating using inequality symbols. 
  • For example, when ordering from least to greatest, the set could be written as –$\frac{\text{5}}{\text{8}}$,  −0.33, 2, 5.8 or as –$\frac{\text{5}}{\text{8}}$ < −0.33 < 2 < 5.8.
• Instruction includes the use of technology to help define and explore rational numbers.

 

Common Misconceptions or Errors

• Students may think if a positive number is larger than a second positive number that the relationship will hold true given the negative or opposite of the given numbers. 
  • For example, if given 6.75 > 6.71, then a student may think that – 6.75 > −6.71. Using a number line (vertical or horizontal) can help students to see the relationship with whole numbers, meaning the greater value is always to the right (horizontal) or above (vertical). 
This relationship also holds true with rational numbers. If students accurately plot the points they can determine which value is larger by looking at the position. 
• Students may think that rational numbers cannot be compared unless they are in the same form (i.e., only fractions can be compared to fractions). Instruction should showcase how to make comparisons using benchmark values for plotting numbers without converting between forms.

 

Strategies to Support Tiered Instruction

• Instruction includes using a physical or digital number line where students can plot the values and see that the further to the left they move on the number line, the lesser the number. 
• When comparing positive numbers in different forms, students may think they can order them just by looking, or that they cannot be compared since they are in different forms. Reviewing converting between fractions, decimals, and percent will help students in the comparing process. 
• Teacher provides explanation of negative numbers (perhaps starting with integers) in terms of currency to help bring real-world connection to the standard. Students should know they would rather have someone owe them $34 over someone owing them $21. Similarly, they understand that if they owed someone $6.25 (−6.25), that is less than owing them $11.84 (−11.84). Then, using the number lines, students can begin to recognize patterns that will bridge their understanding from conceptual to procedural, as well as apply their understanding in real world situations. 
• When plotting rational numbers, students should start by making assumptions and reasonable estimations. 
  • For example, if a student is plotting −4$\frac{\text{3}}{\text{5}}$ on a number line, they need to know that $\frac{\text{3}}{\text{5}}$ is more than half. So, to graph this value, they should plot a point between −4 and −5, with the point closer to the −5. Then, the student can more precisely adjust the point if necessary if any other value is between −4 and −5. 
• Instruction showcases how to make comparisons using benchmark values for plotting numbers without needing to convert between forms.

 

Instructional Tasks

Instructional Task 1 (MTR.1.1, MTR.4.1, MTR.5.1)

• Part A. Arrange the cards on the number line.
• Part B. Discuss your rationale for placement of rational numbers in your group or with a partner.
• Part C. Compare your work and discuss whether you agree or disagree on rational number placement on the number line.

Instructional Task 2 (MTR.6.1, MTR.7.1) 
Sharon was being paid to paint a mural on a wall in the gym. The mural was supposed to be at least 6.2 feet tall and 12.8 feet wide. When she measured her completed mural, the tape measure showed the height as 6$\frac{\text{1}}{\text{4}}$ feet and the width as 12$\frac{\text{7}}{\text{8}}$ feet. Did the dimensions of Sharon’s mural meet the given requirements? How do you know?