General Information
Subject Area: Mathematics (B.E.S.T.)
Grade: 5
Strand: Algebraic Reasoning
Date Adopted or Revised: 08/20
Status: State Board Approved
Benchmark Instructional Guide
Connecting Benchmarks/Horizontal Alignment
Terms from the K-12 Glossary
- Equal Sign
- Equation
- Expression
Vertical Alignment
Previous Benchmarks
Next Benchmarks
Purpose and Instructional Strategies
The purpose of this benchmark is to determine if students can connect their understanding of using the four operations reliably or fluently (MTR.3.1) to the concept of the meaning of the equal sign. Students have evaluated whether equations are true or false since grade 2. In grade 5, additional expectations include non-whole numbers and parentheses. In grade 6, students extend this work to involve negative numbers and inequalities (MA.6.AR.2.1).- Students will use their understanding of order of operations (MA.5.AR.2.2) to simplify expressions on each side of an equation (MTR.5.1).
- Students will determine if the expression on the left of equal sign is equivalent to the expression to the right of the equal sign. If these expressions are equivalent, then the equation is true.
- Students may use comparative relational thinking, instead of solving, in order to determine if the equation is true or false (MTR.2.1, MTR.3.1, MTR.5.1).
Common Misconceptions or Errors
- Some students may not understand that the equal sign is a relational symbol showing expressions on both sides that are the same. While justifying whether equations are true or false, students should explain what makes the equation true.
Strategies to Support Tiered Instruction
- Instruction includes opportunities to explore the meaning of the equal sign. The teacher provides explicit clarification that the equal sign means “the same as” rather than “the answer is” along with multiple examples for students to evaluate equations as true or false using the four operations with the answers on both the left and right side of the equation. Instruction begins by using single numbers on either side of the equal sign to build understanding using the same equations written in different ways to reinforce the concept.
- For example, the teacher shows the following equations, asking students if they are true or false statements. Students explain why each equation is true or false. This is repeated with additional true and false equations using the four operations.
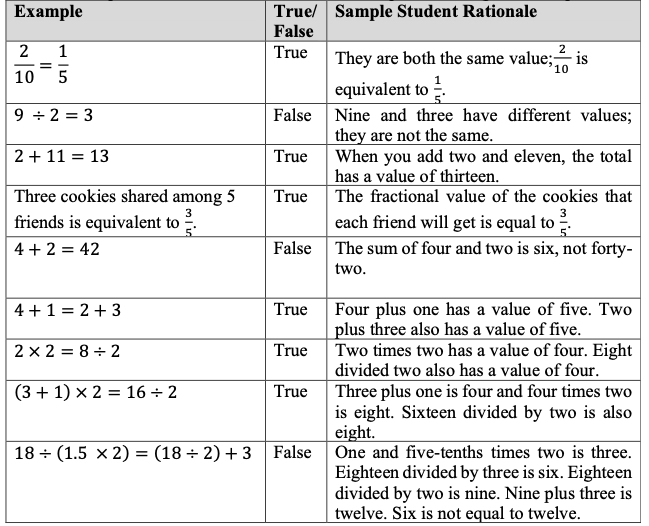
- For example, the teacher shows the following equations having students use counters, drawings, or base-ten blocks on a t-chart to represent the equation. The teacher asks students if they are true or false statements and to explain what makes equations true. This is repeated with additional true and false equations using the four operations.

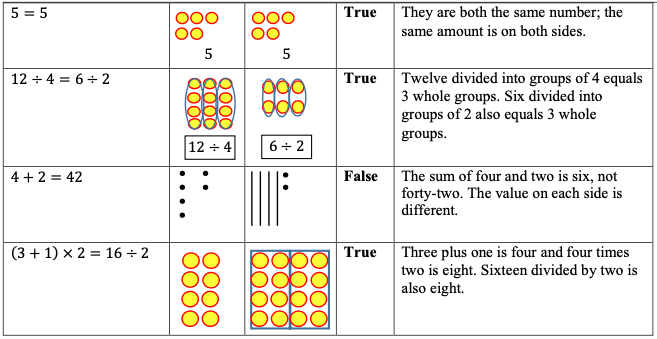
Instructional Tasks
Instructional Task 1 (MTR.2.1)
Using the numbers below, create the equation that is true.( ___ × ___ ) − ____ = ____ − ____
12, 6.2, 5 1, 4, 3.5
Instructional Items
Instructional Item 1
Which best explains the equation below?13.8 − 6 + 3 = 4 × 1.2
- a. This equation is true because both sides of the equation are equal to 4.8.
- b. This equation is true because both sides of the equation are equal to 10.8.
- c. This equation is false because both sides of the equation are equal to 4.8.
- d. This equation is false because both sides of the equation are unequal.
*The strategies, tasks and items included in the B1G-M are examples and should not be considered comprehensive.
