Standard #: MA.5.AR.2.2


This document was generated on CPALMS - www.cpalms.org



Evaluate multi-step numerical expressions using order of operations.


Examples


Patti says the expression 12÷2×3 is equivalent to 18 because she works each operation from left to right. Gladys says the expression 12÷2×3 is equivalent to 2 because first multiplies 2×3 then divides 6 into 12. David says that Patti is correctly using order of operations and suggests that if parentheses were added, it would give more clarity.

Clarifications


Clarification 1: Multi-step expressions are limited to any combination of arithmetic operations, including parentheses, with whole numbers, decimals and fractions.

Clarification 2: Within this benchmark, the expectation is not to include exponents or nested grouping symbols.

Clarification 3: Decimals are limited to hundredths. Expressions cannot include division of a fraction by a fraction.



General Information

Subject Area: Mathematics (B.E.S.T.)
Grade: 5
Strand: Algebraic Reasoning
Standard: Demonstrate an understanding of equality, the order of operations and equivalent numerical expressions.
Date Adopted or Revised: 08/20
Status: State Board Approved

Benchmark Instructional Guide

Connecting Benchmarks/Horizontal Alignment

 

Terms from the K-12 Glossary

  • Expression
  • Order of Operations

 

Vertical Alignment

Previous Benchmarks

 

Next Benchmarks

 

Purpose and Instructional Strategies

The purpose of this benchmark is for students to use the order of operations to evaluate numerical expressions. In grade 4, students had experience with numerical expressions involving all four operations (MA.4.AR.2.1, MA.4.AR.2.2), but the focus was not on order of operations. In grade 6, students will be evaluating algebraic expressions using substitution and these expressions can include negative numbers (MA.6.AR.1.3). 
  • Begin instruction by exposing student to expressions that have two operations without any grouping symbols, before introducing expressions with multiple operations. Use the same digits, with the operations in a different order, and have students evaluate the expressions, then discuss why the value of the expression is different.  
o For example, have students evaluate 6 × 3 + 7 and 6 + 3 × 7. 
  • In grade 5, students should learn to first work to simplify within any parentheses, if present in the expression. Within the parentheses, the order of operations is followed. Next, while reading left to right, perform any multiplication and division in the order in which it appears. Finally, while reading from left to right, perform addition and subtraction in the order in which it appears. 
  • During instruction, students should be expected to explain how they used the order of operations to evaluate expressions and share with others. To address misconceptions around the order of operations, instruction should include reasoning and error analysis tasks for students to complete (MTR.3.1, MTR.4.1, MTR.5.1).

 

Common Misconceptions or Errors

  • When students learn mnemonics like PEMDAS to perform the order of operations, they can confuse that multiplication must always be performed before division, and likewise addition before subtraction. Students should have experiences solving expressions with multiple instances of procedural operations and their inverse, such as addition and subtraction, so they learn how to solve them left to right.

 

Strategies to Support Tiered Instruction

  • Instruction includes opportunities to solve expressions with multiple instances of procedural operations and their inverse, explicitly teaching the order of operations with an emphasis on the left to right order to solving multiplication and division, and addition and subtraction. Students use models or drawings as they solve. 
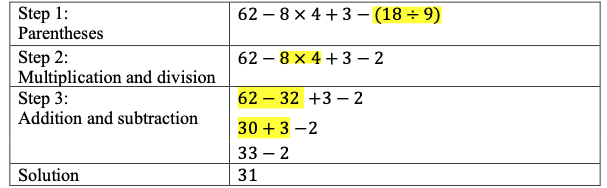
    • For example, the teacher displays the following problem: 62 − 8 × 4 + 3 − (18 ÷ 9). The teacher reviews the order of operations, reminding students that they must work to simplify within the parentheses first. The teacher then prompts students to multiply and divide from left to right next. Then, students are prompted to add and subtract from left to right and reminded that adding and subtracting fall within the same step. So, they will need to subtract 62 − 32 to get 30 and then add 30 + 3. The teacher repeats with additional expressions containing multiplication, division, addition, and subtraction in a variety of orders. 

  • Instruction includes manipulatives to practice solving expressions with multiple instances of procedural operations and their inverse, such as addition and subtraction, so they learn how to solve them left to right. Instruction also includes explicitly teaching the order of operations with an emphasis on the left to right order to solving multiplication and division, and addition and subtraction. Students use manipulatives as they solve. 
    • For example, display the following problem: 5 − 10 ÷ 5 + (2 × 3). The teacher reviews the order of operations, reminding students that they must work to simplify within the parentheses first. The teacher prompts students to multiply and divide from left to right next. Then, prompts students to add and subtract from left to right. Finally, the teacher reminds students that adding and subtracting falls within the same step, so they will need to subtract 5 − 2 before they add +6. This is repeated with additional expressions containing multiplication, division, addition, and subtraction in a variety of orders.

 

Instructional Tasks

Instructional Task 1 (MTR.4.1) 

The two equations below are very similar. Are both equations true? Why or why not? 
Equation One: 4 × 6 + 3 × 2 + 4 = 34 
Equation Two: 4 × (6 + 3 × 2 + 4) = 64 

 

Instructional Task 2 (MTR.5.1) 

Part A. Insert one set of parentheses around two numbers in the expression below. Then evaluate the expression.

40 ÷ 5 × 2 + 6 

Part B. Now insert one set of parentheses around a different pair of numbers. Then evaluate this expression.

40 ÷ 5 × 2 + 6 

 

Instructional Items

Instructional Item 1

What is the value of the numerical expression below: 
(2.45 + 3.05) ÷ (7.15 − 2.15)  

 

Instructional Item 2

A numerical expression is evaluated as shown. 
12 (3 x 5 + 1) - 2

 

In which step does the first mistake appear 
  • a. Step 1: 12 × (15 + 1) − 2 
  • b. Step 2: 12 × 14
  • c. Step 3: 142
  • d. Step 4: 7 

 

*The strategies, tasks and items included in the B1G-M are examples and should not be considered comprehensive.



Related Courses

Course Number1111 Course Title222
5012070: Grade Five Mathematics (Specifically in versions: 2014 - 2015, 2015 - 2022, 2022 - 2024, 2024 and beyond (current))
7712060: Access Mathematics Grade 5 (Specifically in versions: 2014 - 2015, 2015 - 2018, 2018 - 2022, 2022 and beyond (current))
5012065: Grade 4 Accelerated Mathematics (Specifically in versions: 2019 - 2022, 2022 - 2024, 2024 and beyond (current))
5012015: Foundational Skills in Mathematics 3-5 (Specifically in versions: 2019 - 2022, 2022 - 2024, 2024 and beyond (current))


Related Access Points

Access Point Number Access Point Title
MA.5.AR.2.AP.2 Evaluate an expression containing three terms and one set of parentheses.


Related Resources

Formative Assessments

Name Description
Evaluating Expressions

Students are asked to evaluate two similar expressions and explain why the answers are different.

More Expressions

Students are asked to insert parentheses into an expression in two different ways, evaluate each way, and explain why the answers are different.

Lesson Plans

Name Description
Gimme Two Steps!

In this lesson, students will create representations for different multi-step word problems. One of these representations will be an expression with a variable.

Please Excuse My Dear Aunt Sally

The students will learn and use the order of operations. In groups, the students will work together to discover the importance of following step-by-step instructions and apply that knowledge in solving algebraic equations by correctly using the order of operations. Students should accurately use the mathematical steps of the PEMDAS model to solve mathematical expressions.

Fly Runners Order of Operations MEA

In this Model Eliciting Activity, MEA, the students will determine the best ad for a tennis shoe company.

Model Eliciting Activities, MEAs, are open-ended, interdisciplinary problem-solving activities that are meant to reveal students’ thinking about the concepts embedded in realistic situations. MEAs resemble engineering problems and encourage students to create solutions in the form of mathematical and scientific models. Students work in teams to apply their knowledge of science and mathematics to solve an open-ended problem, while considering constraints and tradeoffs. Students integrate their ELA skills into MEAs as they are asked to clearly document their thought process. MEAs follow a problem-based, student centered approach to learning, where students are encouraged to grapple with the problem while the teacher acts as a facilitator. To learn more about MEA’s visit: https://www.cpalms.org/cpalms/mea.aspx

Original Student Tutorials

Name Description
Bee A Coder Part 4: Repeat Loops

Learn how to use repeat loops in this interactive tutorial. Repeat loops iterate though a list of instructions based on a desired number of times. Combined with variables, condition statements, if statements, and repeat loops we practice using order of operations to code.

This is part 1 of a 4-part series. Click below to check out the other tutorials in the series.

Bee A Coder Part 3: If Statements

Learn how to perform instructions using an if statement and explore relational operators (less than, greater than, equal and not equal to) and how they are used to compare to values in this interactive tutorial.

Bee A Coder Part 2: Condition Statements

Construct efficient lines of code using condition- and if-statements to solve equations as you complete this interactive tutorial. You'll also review the order of operations in expressions.

This is part 2 of a 4-part series on coding. Click below to open the other tutorials in the series.

The Expression Debate

Learn to evaluate expressions that have all four operations (multiplication, division, addition, and subtraction) and parentheses as you settle debates in this interactive tutorial.

Problem-Solving Tasks

Name Description
Bowling for Numbers

The purpose of this game is to help students think flexibly about numbers and operations and to record multiple operations using proper notation. Students eager to knock down all of the pins quickly develop patterns in their expressions. They may re-use parts of an expression, perhaps changing just the final operation.

Watch Out for Parentheses 1

This problem asks the student to evaluate six numerical expressions that contain the same integers and operations yet have differing results due to placement of parentheses. This type of problem helps students to see structure in numerical expressions. In later grades they will be working with similar ideas in the context of seeing and using structure in algebraic expressions.

Tutorials

Name Description
Evaluate an Expression With and Without Parentheses

This Khan Academy tutorial video presents the impact of parentheses notation in an expression.  As the tutorial does the same problem with and without parenthesis, negative numbers are part of the solution.  

Introduction to the Order of Operations

In this video, you will see why it is important to have one agreed upon order of operations.

Virtual Manipulative

Name Description
Order of Operations Quiz

In this activity, students practice solving algebraic expressions using order of operations. The applet records their score so the student can track their progress. This activity allows students to practice applying the order of operations when solving problems. This activity includes supplemental materials, including background information about the topics covered, a description of how to use the application, and exploration questions for use with the java applet.

Student Resources

Original Student Tutorials

Name Description
Bee A Coder Part 4: Repeat Loops:

Learn how to use repeat loops in this interactive tutorial. Repeat loops iterate though a list of instructions based on a desired number of times. Combined with variables, condition statements, if statements, and repeat loops we practice using order of operations to code.

This is part 1 of a 4-part series. Click below to check out the other tutorials in the series.

Bee A Coder Part 3: If Statements:

Learn how to perform instructions using an if statement and explore relational operators (less than, greater than, equal and not equal to) and how they are used to compare to values in this interactive tutorial.

Bee A Coder Part 2: Condition Statements:

Construct efficient lines of code using condition- and if-statements to solve equations as you complete this interactive tutorial. You'll also review the order of operations in expressions.

This is part 2 of a 4-part series on coding. Click below to open the other tutorials in the series.

The Expression Debate:

Learn to evaluate expressions that have all four operations (multiplication, division, addition, and subtraction) and parentheses as you settle debates in this interactive tutorial.

Problem-Solving Task

Name Description
Watch Out for Parentheses 1:

This problem asks the student to evaluate six numerical expressions that contain the same integers and operations yet have differing results due to placement of parentheses. This type of problem helps students to see structure in numerical expressions. In later grades they will be working with similar ideas in the context of seeing and using structure in algebraic expressions.

Tutorials

Name Description
Evaluate an Expression With and Without Parentheses:

This Khan Academy tutorial video presents the impact of parentheses notation in an expression.  As the tutorial does the same problem with and without parenthesis, negative numbers are part of the solution.  

Introduction to the Order of Operations:

In this video, you will see why it is important to have one agreed upon order of operations.

Virtual Manipulative

Name Description
Order of Operations Quiz:

In this activity, students practice solving algebraic expressions using order of operations. The applet records their score so the student can track their progress. This activity allows students to practice applying the order of operations when solving problems. This activity includes supplemental materials, including background information about the topics covered, a description of how to use the application, and exploration questions for use with the java applet.



Parent Resources

Problem-Solving Tasks

Name Description
Bowling for Numbers:

The purpose of this game is to help students think flexibly about numbers and operations and to record multiple operations using proper notation. Students eager to knock down all of the pins quickly develop patterns in their expressions. They may re-use parts of an expression, perhaps changing just the final operation.

Watch Out for Parentheses 1:

This problem asks the student to evaluate six numerical expressions that contain the same integers and operations yet have differing results due to placement of parentheses. This type of problem helps students to see structure in numerical expressions. In later grades they will be working with similar ideas in the context of seeing and using structure in algebraic expressions.



Printed On:4/2/2025 2:12:39 AM
Print Page | Close this window