Standard #: MA.5.AR.2.1


This document was generated on CPALMS - www.cpalms.org



Translate written real-world and mathematical descriptions into numerical expressions and numerical expressions into written mathematical descriptions.


Examples


The expression 4.5 + (3×2) in word form is four and five tenths plus the quantity 3 times 2.

Clarifications


Clarification 1: Expressions are limited to any combination of the arithmetic operations, including parentheses, with whole numbers, decimals and fractions. 

Clarification 2: Within this benchmark, the expectation is not to include exponents or nested grouping symbols.



General Information

Subject Area: Mathematics (B.E.S.T.)
Grade: 5
Strand: Algebraic Reasoning
Standard: Demonstrate an understanding of equality, the order of operations and equivalent numerical expressions.
Date Adopted or Revised: 08/20
Status: State Board Approved

Benchmark Instructional Guide

Connecting Benchmarks/Horizontal Alignment

 

Terms from the K-12 Glossary

  • Expression

 

Vertical Alignment

Previous Benchmarks

 

Next Benchmarks

 

Purpose and Instructional Strategies

The purpose of this benchmark is for students to translate between numerical and written mathematical expressions. This builds from previous work where students wrote equations with unknowns in any position of the equation in grade 4 (MA.4.AR.2.2). Algebraic expressions are a major theme in grade 6 starting with MA.6.AR.1.1. 
  • During instruction, teachers should model how to translate numerical expressions into words using correct vocabulary. This includes naming fractions and decimals correctly. Students should use diverse vocabulary to describe expressions. 
    • For example, in the expression 4.5 + (3 × 2) could be read in multiple ways to show its operations. Students should explore them and find connections between their meanings (MTR.3.1, MTR.4.1, MTR.5.1). 
      • 4 and five tenths plus the quantity 3 times 2  
      • 4 and 5 tenths plus the product of 3 and 2 
      • The sum of 4 and 5 tenths and the quantity 3 times 2 
      • The sum of 4 and 5 tenths and the products of 3 and 2 
  • The expectation of this benchmark is to not use exponents or nested grouping symbols. Nested grouping symbols refer to grouping symbols within one another in an expression, like in 3 + [5.2 + (4 × 2)]. 
  • Instruction of this benchmark helps students understand the order of operations, the expectation of MA.5.AR.2.2.

 

Common Misconceptions or Errors

  • Students can misrepresent decimal and fraction numbers in words. This benchmark helps students practice naming numbers according to place value. 
  • Some students can confuse the difference between what is expected in the expressions 5(9 + 3) and 5 + (9 + 3). Students need practice naming the former as multiplication (e.g., 5 times the sum of 9 and 3) and understanding that in that expression, both 5 and 9 + 3 are factors.

 

Strategies to Support Tiered Instruction

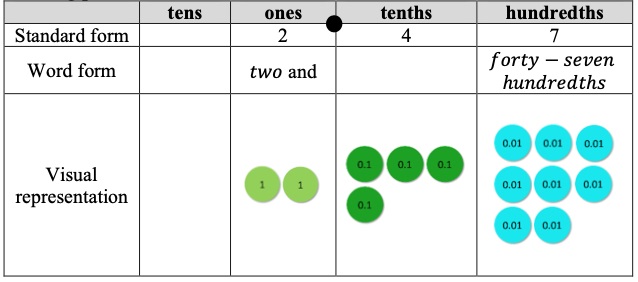
  • Instruction includes opportunities to name fractions and decimals correctly according to place value. The teacher provides students a place value chart to support correctly naming decimals. Students use appropriate terminology for naming fractions. 
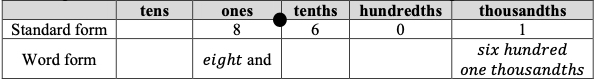
o For example, write 8.601 in standard form and word form in a place value chart. 
place value chart
    • For example, students write 10.36 in standard form and word form in a place value chart. 
place value chart
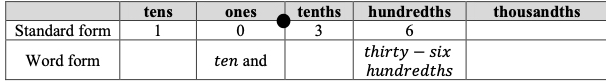
    • For example, students write 2.47 in standard form and word form in a place value chart using place value disks. 

    • For example, students write 512 in word form (five twelfths). 
    • For example, students write 2 78 in word form (two and seven eighths). This is repeated with additional fractions and decimals. 
  • Instruction includes opportunities to correctly translate numerical expressions into words using appropriate vocabulary.
    • For example, the teacher has students read aloud the following expression and write in word form. Next, the teacher models one way of reading aloud and has students provide alternate ways while using questioning to facilitate the conversation about the multiple ways the expression can be read aloud to show its operations. 
18.49−(27÷3) 
      • Eighteen and forty-nine hundredths minus the quotient of twenty-seven divided by three.
      • 18 and 49 hundredths minus the quantity 27 divided by 3.
      • The difference between 18 and 49 hundredths and the quotient of 27 divided by 3. 
      • The difference between 18 and 49 hundredths and the quantity 27 divided by 3. 
    • For example, the teacher models how to translate the expression 5(9 + 3) into words (e.g., 5 times the sum of 9 and 3) and explains that in this expression, both 5 and 9 + 3 are factors.

 

Instructional Tasks

Instructional Task 1 (MTR.4.1) 

  • Nadia sees the numerical expression 6.5 + 12 (4-2). She translates the expression as, “6 and five tenths plus 1 half times 4, minus 2."
  • Part A: Is her translation correct? Explain. 
  • Part B: Evaluate the expression.

 

Instructional Task 2 (MTR.3.1) 

Translate the written mathematical description below into a numerical expression: Divide the difference of 20 and 5 by the sum of 4 and 1. 

 

Instructional Items

Instructional Item 1 

Translate the numerical expression below into a written mathematical description.
2(53.8 + 4 − 22.9) 

 

Instructional Item 2

Translate the written mathematical description into a numerical expression. “one half the difference of 6 and 8 hundredths and 2” 

*The strategies, tasks and items included in the B1G-M are examples and should not be considered comprehensive. 




Related Courses

Course Number1111 Course Title222
5012070: Grade Five Mathematics (Specifically in versions: 2014 - 2015, 2015 - 2022, 2022 and beyond (current))
7712060: Access Mathematics Grade 5 (Specifically in versions: 2014 - 2015, 2015 - 2018, 2018 - 2022, 2022 and beyond (current))
5012065: Grade 4 Accelerated Mathematics (Specifically in versions: 2019 - 2022, 2022 and beyond (current))
5012015: Foundational Skills in Mathematics 3-5 (Specifically in versions: 2019 - 2022, 2022 and beyond (current))


Related Access Points

Access Point Number Access Point Title
MA.5.AR.2.AP.1 Translate mathematical descriptions (e.g., five plus two; the product of three and four) into numerical expressions with two terms.


Related Resources

Formative Assessments

Name Description
Write the Expression

Students are presented with a verbal description of a numerical expression and are asked to write the expression and then compare it to a similar expression.

Brayden’s Video Game

Students are asked to write an expression requiring more than one operation and the use of parentheses to model a word problem.

How Much Greater Is The Product?

Students are asked to model an expression that is a multiple of a sum and to compare the expression to the sum.

Comparing Products

Students are asked to analyze and compare two related products.

Lesson Plan

Name Description
“Express Yourself!” with Math Story Chains

Students work in small groups to write math story chains (multi-step real world problems) and write expressions or equations for their story chains.

Original Student Tutorial

Name Description
Expressions: Not Just for Faces

Learn how to write mathematical expressions while making faces in this interactive tutorial!

Problem-Solving Tasks

Name Description
Video Game Scores

This task asks students to exercise both of these complementary skills, writing an expression in part (a) and interpreting a given expression in (b). The numbers given in the problem are deliberately large and "ugly" to discourage students from calculating Eric's and Leila's scores. The focus of this problem is not on numerical answers, but instead on building and interpreting expressions that could be entered in a calculator or communicated to another student unfamiliar with the context.

Seeing is Believing

The purpose of this task is to help students see that 4×(9+2) is four times as big as (9+2). Though this task may seem very simple, it provides students and teachers with a very useful visual for interpreting an expression without evaluating it because they can see for themselves that 4×(9+2) is four times as big as (9+2).

Comparing Products

The purpose of this task is to generate a classroom discussion that helps students synthesize what they have learned about multiplication in previous grades. It builds on applying properties of operations as strategies to multiply and divide and interpreting a multiplication equation as a comparison.

Words to Expressions 1

This problem allows student to see words that can describe an expression although the solution requires nested parentheses.  Additionally , the words (add, sum) and (product, multiply) are all strategically used so that the student can see that these words have related meanings.

Tutorials

Name Description
Translating Expressions with Parentheses

This Khan Academy tutorial video interprets written statements and writes them as mathematical expressions.

Constructing a Numerical Expression Example

This Khan Academy tutorial video demonstrates how to write a simple expression from a word problem.

Student Resources

Original Student Tutorial

Name Description
Expressions: Not Just for Faces:

Learn how to write mathematical expressions while making faces in this interactive tutorial!

Problem-Solving Tasks

Name Description
Video Game Scores:

This task asks students to exercise both of these complementary skills, writing an expression in part (a) and interpreting a given expression in (b). The numbers given in the problem are deliberately large and "ugly" to discourage students from calculating Eric's and Leila's scores. The focus of this problem is not on numerical answers, but instead on building and interpreting expressions that could be entered in a calculator or communicated to another student unfamiliar with the context.

Seeing is Believing:

The purpose of this task is to help students see that 4×(9+2) is four times as big as (9+2). Though this task may seem very simple, it provides students and teachers with a very useful visual for interpreting an expression without evaluating it because they can see for themselves that 4×(9+2) is four times as big as (9+2).

Comparing Products:

The purpose of this task is to generate a classroom discussion that helps students synthesize what they have learned about multiplication in previous grades. It builds on applying properties of operations as strategies to multiply and divide and interpreting a multiplication equation as a comparison.

Words to Expressions 1:

This problem allows student to see words that can describe an expression although the solution requires nested parentheses.  Additionally , the words (add, sum) and (product, multiply) are all strategically used so that the student can see that these words have related meanings.

Tutorials

Name Description
Translating Expressions with Parentheses:

This Khan Academy tutorial video interprets written statements and writes them as mathematical expressions.

Constructing a Numerical Expression Example:

This Khan Academy tutorial video demonstrates how to write a simple expression from a word problem.



Parent Resources

Problem-Solving Tasks

Name Description
Video Game Scores:

This task asks students to exercise both of these complementary skills, writing an expression in part (a) and interpreting a given expression in (b). The numbers given in the problem are deliberately large and "ugly" to discourage students from calculating Eric's and Leila's scores. The focus of this problem is not on numerical answers, but instead on building and interpreting expressions that could be entered in a calculator or communicated to another student unfamiliar with the context.

Seeing is Believing:

The purpose of this task is to help students see that 4×(9+2) is four times as big as (9+2). Though this task may seem very simple, it provides students and teachers with a very useful visual for interpreting an expression without evaluating it because they can see for themselves that 4×(9+2) is four times as big as (9+2).

Comparing Products:

The purpose of this task is to generate a classroom discussion that helps students synthesize what they have learned about multiplication in previous grades. It builds on applying properties of operations as strategies to multiply and divide and interpreting a multiplication equation as a comparison.

Words to Expressions 1:

This problem allows student to see words that can describe an expression although the solution requires nested parentheses.  Additionally , the words (add, sum) and (product, multiply) are all strategically used so that the student can see that these words have related meanings.



Printed On:4/26/2024 9:12:10 PM
Print Page | Close this window