Standard #: MA.5.NSO.1.1

Benchmark Instructional Guide

Connecting Benchmarks/Horizontal Alignment

• MA.5.NSO.2.4
• MA.5.NSO.2.5 
• MA.5.AR.2.1
• MA.5.AR.2.2
• MA.5.AR.2.3 
• MA.5.M.1.1
• MA.5.M.2.1

 

Terms from the K-12 Glossary

• NA

 

Vertical Alignment

Previous Benchmarks

• MA.4.NSO.1.1

 

Next Benchmarks

• MA.6.NSO.2.1

 

Purpose and Instructional Strategies

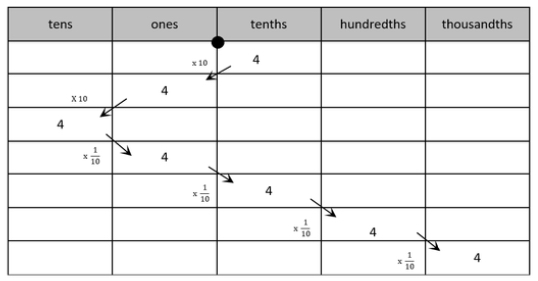
This purpose of this benchmark is for students to reason about the magnitude of digits in a number. This benchmark extends the understanding from grade 4 (MA.4.NSO.1.1), where 1 represents 10 times what it represents in the place to its right and $\frac{\text{1}}{\text{10}}$ of what it represents in the students expressed their understanding that in multi-digit whole numbers, a digit in one place place to its left. All of this work forms the foundation for arithmetic and algorithms with decimals which is completed in grade 6 (MA.6.NSO.2.1). 

• To help students understand the meaning of the 10 times and $\frac{\text{1}}{\text{10}}$ of relationship, students can use base ten manipulatives or simply bundle classroom objects (e.g., paper clips, pretzel sticks). Students should name numbers and use verbal descriptions to explain the relationship between numbers (e.g., “6 is 10 times greater than 6 tenths, and 6 tenths is $\frac{\text{1}}{\text{10}}$ of 6”). In addition to physical manipulatives, place value charts help students understand the relationship between digits in different places (MTR.2.1). 
• Instruction of this benchmark should connect with student work with whole numbers. For example, students who understand 35 × 2 = 70 can reason that 3.5 × 2 = 7 because 3.5 is $\frac{\text{1}}{\text{10}}$ of 35, therefore its product with 2 will be $\frac{\text{1}}{\text{10}}$ of 70 (MTR.5.1).

 

Common Misconceptions or Errors

• Students can misunderstand what “$\frac{\text{1}}{\text{10}}$of” a number represents. Teachers can connect of $\frac{\text{1}}{\text{10}}$ of to “ten times less” or “dividing by 10” to help students connect $\frac{\text{1}}{\text{10}}$ of a number to 10 times greater. 
• Students who use either rule “move the decimal point” or “shift the digits” without understanding when multiplying by a power of ten can easily make errors. Students need to understand that from either point of view, the position of the decimal point marks the transition between the ones and the tenths place.

 

Strategies to Support Tiered Instruction

• Instruction includes the use of place value charts and models such as place value disks to demonstrate how the value of a digit changes if the digit moves one place to the left or right. Explicit instruction includes using place value understanding to make the connections between the concepts of “$\frac{\text{1}}{\text{10}}$ of,” “ten times less” and “dividing by 10.” Place value charts are used to demonstrate that the decimal point marks the transition between the ones place and the tenths place. 
  • For example, students multiply 4 by 10, then record 4 and the product of 40 in a place value chart. This process is repeated by multiplying 40 by 10 while asking students to explain what happens to the digit 4 each time it is multiplied by 10. Next, the teacher explains that multiplying by $\frac{\text{1}}{\text{10}}$ is the same as dividing by 10. Students multiply 400 by $\frac{\text{1}}{\text{10}}$ and record the product in their place value chart. This process is repeated, multiplying 40 and 4 by $\frac{\text{1}}{\text{10}}$. The teacher asks students to explain how the value of the 4 changed when being multiplied by 10 and $\frac{\text{1}}{\text{10}}$. 

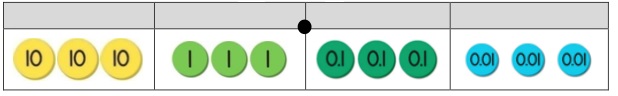
• For example, instruction includes using a familiar context such as money, asking students to explain the value of each digit in $33.33. Next, students represent 33.33 in a place value chart using place value disks. Then, students compare the value of the whole numbers (3 dollars and 30 dollars) and compare 0.3 and 0.03 (30 cents and 3 cents). The teacher asks, “How does the value of the three in the hundredths place compare to the value of the three in the tenths place?” and explains that the three in the hundredths place is $\frac{\text{1}}{\text{10}}$ the value of the three in the tenths place and that multiplying by $\frac{\text{1}}{\text{10}}$ is the same as dividing by 10.

 

Instructional Tasks

Instructional Task 1 (MTR.7.1) 

At the Sunshine Candy Store, salt water taffy costs $0.18 per piece. 

• Part A. How much would 10 pieces of candy cost? 
• Part B. How much would 100 pieces of candy cost? 
• Part C. How much would 1000 pieces of candy cost? 
• Part D. At the same store, you can buy 100 chocolate coins for $89.00. How much does each chocolate coin cost? Explain how you know.

 

Instructional Items

Instructional Item 1

Which statement correctly compares 0.034 and 34? 

• a. 0.034 is 10 times the value of 34. 
• b. 0.034 is $\frac{\text{1}}{\text{10}}$ the value of 34. 
• c. 0.034 is $\frac{\text{1}}{\text{100}}$ the value of 34. 
• d. 0.034 is $\frac{\text{1}}{\text{1000}}$the value of 34. 

 

Instructional Item 2 

What number is 100 times the value of 45.03? 

 

*The strategies, tasks and items included in the B1G-M are examples and should not be considered comprehensive.