Standard #: MA.4.GR.1.3


This document was generated on CPALMS - www.cpalms.org



Solve real-world and mathematical problems involving unknown whole-number angle measures. Write an equation to represent the unknown.


Examples


A 60° angle is decomposed into two angles, one of which is 25°. What is the measure of the other angle?

Clarifications


Clarification 1: Instruction includes the connection to angle measure as being additive.

General Information

Subject Area: Mathematics (B.E.S.T.)
Grade: 4
Strand: Geometric Reasoning
Standard: Draw, classify and measure angles.
Date Adopted or Revised: 08/20
Status: State Board Approved

Benchmark Instructional Guide

Connecting Benchmarks/Horizontal Alignment

 

Terms from the K-12 Glossary

  • Angle
  • Circle 
  • Right Angle 
  • Straight Angle

 

Vertical Alignment

Previous Benchmarks

 

Next Benchmarks

 

Purpose and Instructional Strategies

The purpose of this benchmark is to extend student thinking about angle measures beyond right angles that were taught in grade 3 (MA.3.GR.1.1) and introducing the idea that angle measures are additive (MA.4.GR.1.2). Students will use this idea to find a missing angle measure. 
  • For instruction, students should use protractors to draw angles that add up to make right angles, straight angles and circles.
  • With the knowledge that angle measures are additive, students can solve interesting and challenging problems with all four operations to find the measurements of unknown angles on a diagram in real world and mathematical problems. 
  • Students can use a protractor to ensure that they develop understanding of benchmark angles (e.g., 30°, 45°, 60° and 90°).

 

Common Misconceptions or Errors

  • Students may make errors when writing equations used to solve angle measurement problems. During instruction, expect students to justify their equations and solutions. Students may not understand that straight lines, even if intersected, measure 180°.

 

Strategies to Support Tiered Instruction

  • The teacher provides a right angle, straight angle, or circle and asks students to use the protractor to divide the angle into two angles and specify their measurements. The teacher has students write an equation to show that the sum of the two angles is equivalent to the angle they started with and explain their equation. 
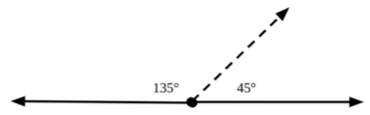
    • For example, when provided with a straight angle, students divide the angle into a 45-degree angle and a 135-degree angle as show below. Students label each angle and explain the equation 135 + 45 = 180, knowing that a straight angle measures 180-degrees. 
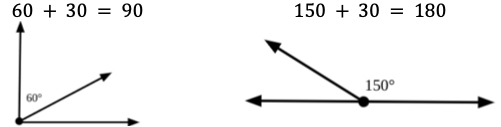
two angles
  • Instruction includes matching equations with given angle images containing angle measures. Students explain the equations and how they know they match the image selected. 
    •  For example, when provided images similar to those shown below, students match the equation from a list of equations provided and explain how they know the equation matches.
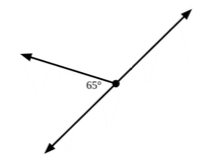
 angles
  • The teacher provides images that contain missing angle measures. Students identify straight angles in the image and use their understanding that straight angles measure 180-degrees to help them find the missing angle measure. 
    • For example, when provided with the image below, students highlight or trace over the straight angle and label as 180-degrees. Students then write an equation using this information and the angle measure provided to help them solve for the unknown angle. 
angles
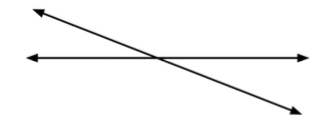
  • Instruction includes identifying straight angles as measuring 180-degrees. 
    • For example, when provided images similar to the one shown below, students highlight straight angles and label them as 180-degrees. In this example, students identify both straight angles, highlighting them with different colors and explain that both have a measure of 180-degrees.

straight angles

 

Instructional Tasks

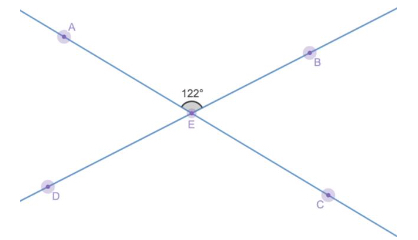
Instructional Task 1 

Two straight lines, AC and BD, intersect at point E. Using the given angle ∠AEB, find the measure of the other 3 angles. 
  • This item may seem a bit challenging but it fits within the benchmark, because it can be solved by repeatedly using additivity, and that fact that a straight line is 180°.
two straight lines

 

Instructional Items

Instructional Item 1 

Carlos is adding angles together to create a 150° angle. Select all the angle measures that Carlos can use to create a 150° angle. 
  • a. 50°+100° 
  • b. 45°+95° 
  • c. 50°+90° 
  • d. 50° +20° +20° 
  • e. 50° +50° +50° 

*The strategies, tasks and items included in the B1G-M are examples and should not be considered comprehensive.



Related Courses

Course Number1111 Course Title222
5012060: Grade Four Mathematics (Specifically in versions: 2014 - 2015, 2015 - 2022, 2022 - 2024, 2024 and beyond (current))
7712050: Access Mathematics Grade 4 (Specifically in versions: 2014 - 2015, 2015 - 2018, 2018 - 2022, 2022 and beyond (current))
5012055: Grade 3 Accelerated Mathematics (Specifically in versions: 2019 - 2022, 2022 - 2024, 2024 and beyond (current))
5012015: Foundational Skills in Mathematics 3-5 (Specifically in versions: 2019 - 2022, 2022 - 2024, 2024 and beyond (current))


Related Access Points

Access Point Number Access Point Title
MA.4.GR.1.AP.3 Recognize that angle measure is additive by exploring when an angle is decomposed into two non-overlapping parts the angle measure of the whole is the sum of the angle measures of the parts.


Related Resources

Formative Assessments

Name Description
Understanding Angles

Students are asked to recognize the additive nature of angles to determine an unknown angle measure.

Using Known Angles

Students are asked to determine adjacent angle measurements using the additive structure of angle measurement and known angle measurements.

Turns on a Skateboard

Students are asked to determine an unknown angle measure that is one component of a larger known angle when given the other component.

Lesson Plans

Name Description
Edible Angles: Decomposing Angles Into Parts of a Whole

This lesson is designed to review help students apply their understanding that when an angle is decomposed into parts, the measure of the parts is equal to the whole measure of the original angle. 

Kissing “V”s

This lesson uses a paper-cutting activity to teach vertical angles. The lesson provides examples in which students must solve equations to find missing measures.

What’s Your Angle?

In this lesson, students will use addition or subtraction to find the measure of two adjacent angles and determine the measure of an unknown angle.

Original Student Tutorials

Name Description
Additive Angles: Wondrous Windows

Decompose and compose various angles while exploring clocks and windows in this interactive tutorial.

Note: this tutorial exceeds clarification limits and is meant as enrichment for students to improve their problem-solving skills.

Additive Angles: Tessellating Tiles

Are you up for a challenge? You will use tile designs to explore how angles can be decomposed into smaller angles and how those parts can be shown as addends in equations in this interactive tutorial.

Note: this tutorial exceeds clarification limits and is meant as enrichment for students who met the standards to increase problem-solving skills.

Perspectives Video: Teaching Ideas

Name Description
Discovering Math Vocabulary in Context

Unlock an effective teaching strategy for vocabulary instruction in this Teacher Perspectives video for educators.

Finding Angles on Clocks

Unlock an effective teaching strategy for identifying angles in this Teacher Perspectives video for educators.

Estimating Angles

Unlock an effective teaching strategy for teaching students to estimate angle measurements in this Teacher Perspectives video for educators.

Problem-Solving Task

Name Description
Finding an unknown angle

The purpose of this task is to give students a problem involving an unknown quantity that has a clear visual representation. Students must understand that the four interior angles of a rectangle are all right angles and that right angles have a measure of 90° and that angle measure is additive.

Student Resources

Original Student Tutorials

Name Description
Additive Angles: Wondrous Windows:

Decompose and compose various angles while exploring clocks and windows in this interactive tutorial.

Note: this tutorial exceeds clarification limits and is meant as enrichment for students to improve their problem-solving skills.

Additive Angles: Tessellating Tiles:

Are you up for a challenge? You will use tile designs to explore how angles can be decomposed into smaller angles and how those parts can be shown as addends in equations in this interactive tutorial.

Note: this tutorial exceeds clarification limits and is meant as enrichment for students who met the standards to increase problem-solving skills.

Problem-Solving Task

Name Description
Finding an unknown angle:

The purpose of this task is to give students a problem involving an unknown quantity that has a clear visual representation. Students must understand that the four interior angles of a rectangle are all right angles and that right angles have a measure of 90° and that angle measure is additive.



Parent Resources

Problem-Solving Task

Name Description
Finding an unknown angle:

The purpose of this task is to give students a problem involving an unknown quantity that has a clear visual representation. Students must understand that the four interior angles of a rectangle are all right angles and that right angles have a measure of 90° and that angle measure is additive.



Printed On:4/15/2025 8:07:27 AM
Print Page | Close this window