General Information
Subject Area: Mathematics (B.E.S.T.)
Grade: 3
Strand: Algebraic Reasoning
Date Adopted or Revised: 08/20
Status: State Board Approved
Benchmark Instructional Guide
Connecting Benchmarks/Horizontal Alignment
Terms from the K-12 Glossary
Vertical Alignment
Previous Benchmarks
Next Benchmarks
Purpose and Instructional Strategies
The purpose of this benchmark is for students to identify, create, and extend numerical patterns using all four operations. Understanding of ordinal numbers from Kindergarten is the foundation for describing the sequence of numbers in a pattern.- “ Identifying” a numerical pattern requires students to determine when a pattern exists in a sequence of numbers, and to potentially determine a rule that can be used to find each term in the sequence. For example, students may be asked whether a pattern exists in the numbers 20, 17, 14, 11,... and to discuss possible rules used to determine the next term.
- “ Creating” a numerical pattern requires students to write a pattern given a rule and starting value. For example, students may be asked to write the first five terms of a sequence that begins with 500 and then create each successive term by subtracting 35 from the previous term.
- Finally, “extending” asks students to identify a future term in a sequence when provided with a rule. For example, students may be asked to find the next three terms in which each term is multiplied by 2 to get the next term 2: 1, 2, ___, ___, ___ (MTR.2.1, MTR.5.1).
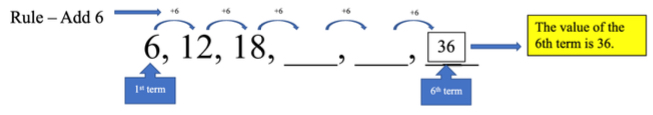
- Instruction of this standard can begin by relating patterns to skip-counting to explore patterns in sequences of numbers and look for relationships in the patterns and be able to describe and make generalizations. When exploring patterns, teachers should allow for students to describe pattern rules flexibly. For example, in the pattern 6, 12, 18,..., one student may describe the pattern’s rule as “ add 6.” Another student may describe the rule as, “ add 7, then subtract 1” or “ list the multiples of 6.” Classroom discussion could compare these rules (MTR.2.1, MTR.4.1).
- Instruction should be limited to whole numbers and operations that are appropriate for Grade 3.
- This foundation for identifying and using patterns extends into Grades 4 and 5 to build algebraic thinking for functions in middle and high school.
Common Misconceptions or Errors
- Students can confuse a term’s number and its value in the sequence. For example, in the pattern 6, 12, 18,..., students can struggle to understand that even though 12 is the 2nd term, 6 is being added to it to find the value of the 3rd term (18). Encourage students to use precise vocabulary while describing patterns to address this confusion.
Strategies to Support Tiered Instruction
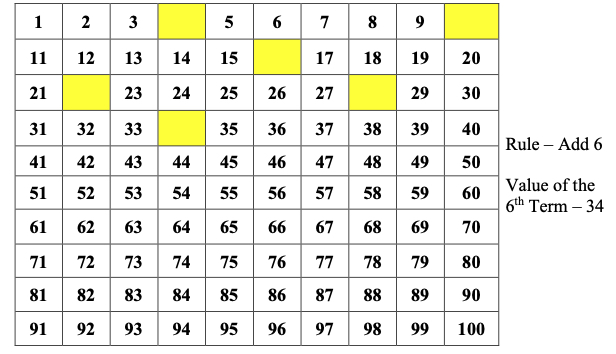
- Instruction includes explicit vocabulary instruction regarding patterns (first term, second term, third term..., rule, value, etc.). Instruction also includes relating the pattern to skip counting where appropriate.
- Example:
- For example, a 100 chart may be a referent that can be used for arithmetic patterns. The teacher makes connections between the rule and counting on the 100s chart.
Instructional Tasks
Instructional Task 1
- Part A. Write a pattern that shows the first 10 multiples of 6.
- Part B. What do you notice about the ones digits of the pattern’s numbers?
- Part C. What would you expect the ones digit of the 12th multiple to be? Explain how you know using the pattern you observed.
Instructional Items
Instructional Item 1
What are the fourth and fifth terms of the sequence below that follows the rule “subtract 4”?34, 30, 26, ____, ____
*The strategies, tasks and items included in the B1G-M are examples and should not be considered comprehensive.