Clusters should not be sorted from Major to Supporting and then taught in that order. To do so would strip the coherence of the mathematical ideas and miss the opportunity to enhance the major work of the grade with the supporting clusters.
-
Also assesses:
- Assessment Limits :
Items that require the student to write a system of equations using a real-world context are limited to a system of 2 x 2 linear equations.Items that require the student to solve a system of equations are limited to a system of 2 x 2 linear equations.
Items that require the student to graph a system of equations or inequalities to find the solution are limited to a 2 x 2 system.
- Calculator :
Neutral
- Clarification :
Students will identify the quantities in a real-world situation that should be represented by distinct variables.Students will write a system of equations given a real-world situation.
Students will graph a system of equations that represents a realworld context using appropriate axis labels and scale.
Students will solve systems of linear equations.
Students will provide steps in an algebraic proof that shows one equation being replaced with another to find a solution for a system of equations.
Students will identify systems whose solutions would be the same through examination of the coefficients.
Students will identify the graph that represents a linear inequality. Students will graph a linear inequality.
Students will identify the solution set to a system of inequalities.
Students will identify ordered pairs that are in the solution set of a system of inequalities.
Students will graph the solution set to a system of inequalities
- Stimulus Attributes :
Items assessing A-CED.1.2 must be placed in a real-world context.Items assessing A-REI.3.5 must be placed in a mathematical context.
Items assessing A-REI.3.6 and A-REI.4.12 may be set in a real-world or mathematical context.
Items may result in infinitely many solutions or no solution
- Response Attributes :
Items may require the student to choose an appropriate level of accuracy.Items may require the student to choose and interpret the scale in a graph.
Items may require the student to choose and interpret units.
For A-CED.1.2, items may require the student to apply the basic modeling cycle.
MAFS.912.A-REI.3.5
MAFS.912.A-REI.3.6
MAFS.912.A-REI.4.12
- Test Item #: Sample Item 1
- Question:
Phillip is designing a deck, where the length of the deck, x, is at least 8 feet (ft). He wants the width to be 4 ft less than the length. The deck will have a bench and a planter, and the remaining area of the deck will be painted. The dimensions for each are shown in the diagram.
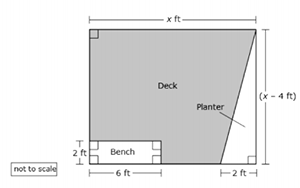
Let A represent the painted area, in square feet, of the deck.
Click on the blank to enter an expression in terms of x that completes the equation for A.
- Difficulty: N/A
- Type: EE: Equation Editor
Related Courses
Related Access Points
Related Resources
Educational Software / Tool
Formative Assessments
Lesson Plans
Original Student Tutorials
Perspectives Video: Professional/Enthusiast
Perspectives Video: Teaching Idea
Problem-Solving Tasks
Tutorials
Unit/Lesson Sequences
Video/Audio/Animations
Virtual Manipulative
STEM Lessons - Model Eliciting Activity
In this Model Eliciting Activity, MEA, students create a plan for a movie theater to stay in business. Data is provided for students to determine the best film to show, and then based on that decision, create a model of ideal sales. Students will create equations and graph them to visually represent the relationships.
Model Eliciting Activities, MEAs, are open-ended, interdisciplinary problem-solving activities that are meant to reveal students’ thinking about the concepts embedded in realistic situations. Click here to learn more about MEAs and how they can transform your classroom.
MFAS Formative Assessments
Students are asked to write an equation in two variables given a verbal description of the relationship among the variables.
Students are asked to sketch a graph that depicts the exponential decline in the population of fir trees in a forest.
Students are asked to write an equation in three variables from a verbal description.
Note: This task may assess skills that exceed the general expectation for this mathematical concept at this grade level. The task is intended for students who have demonstrated mastery within the scope of instruction who may be ready for a more rigorous extensions of the content. As with all materials, ensure to gauge the readiness of students or adapt according to students needs prior to administration.
Students are asked to write a function that represents an annual loss of 3 percent each year.
Original Student Tutorials Mathematics - Grades 9-12
Learn how to create systems of linear equations to represent contextual situations in this interactive tutorial.
This part 6 in a 7-part series. Click below to explore the other tutorials in the series.
- Part 1: Solving Systems of Linear Equations: Using Graphs
- Part 2: Solving Systems of Linear Equations: Substitution
- Part 3: Solving Systems of Linear Equations: Basic Elimination
- Part 4: Solving Systems of Linear Equations: Advanced Elimination
- Part 5: Solving Systems of Linear Equations: Connecting Algebraic Methods to Graphing
- Part 7: Solving Systems of Linear Equations: Word Problems (Coming soon)
Learn how to write equations in two variables in this interactive tutorial.
Student Resources
Original Student Tutorials
Learn how to create systems of linear equations to represent contextual situations in this interactive tutorial.
This part 6 in a 7-part series. Click below to explore the other tutorials in the series.
- Part 1: Solving Systems of Linear Equations: Using Graphs
- Part 2: Solving Systems of Linear Equations: Substitution
- Part 3: Solving Systems of Linear Equations: Basic Elimination
- Part 4: Solving Systems of Linear Equations: Advanced Elimination
- Part 5: Solving Systems of Linear Equations: Connecting Algebraic Methods to Graphing
- Part 7: Solving Systems of Linear Equations: Word Problems (Coming soon)
Type: Original Student Tutorial
Learn how to write equations in two variables in this interactive tutorial.
Type: Original Student Tutorial
Problem-Solving Tasks
The given solutions for this task involve the creation and solving of a system of two equations and two unknowns, with the caveat that the context of the problem implies that we are interested only in non-negative integer solutions. Indeed, in the first solution, we must also restrict our attention to the case that one of the variables is further even. This aspect of the task is illustrative of the mathematical practice of modeling with mathematics, and crucial as the system has an integer solution for both situations, that is, whether we include the dollar on the floor in the cash box or not.
Type: Problem-Solving Task
This task presents a simple but mathematically interesting game whose solution is a challenging exercise in creating and reasoning with algebraic inequalities. The core of the task involves converting a verbal statement into a mathematical inequality in a context in which the inequality is not obviously presented, and then repeatedly using the inequality to deduce information about the structure of the game.
Type: Problem-Solving Task
This task examines the ways in which the plane can be covered by regular polygons in a very strict arrangement called a regular tessellation. These tessellations are studied here using algebra, which enters the picture via the formula for the measure of the interior angles of a regular polygon (which should therefore be introduced or reviewed before beginning the task). The goal of the task is to use algebra in order to understand which tessellations of the plane with regular polygons are possible.
Type: Problem-Solving Task
Tutorials
This video demonstrates solving a word problem by creating a system of linear equations that represents the situation and solving them using elimination.
Type: Tutorial
This video provides a real-world scenario and step-by-step instructions to constructing equations using two variables. Possible follow-up videos include Plotting System of Equations - Yoga Plan, Solving System of Equations with Substitution - Yoga Plan, and Solving System of Equations with Elimination - Yoga Plan.
Type: Tutorial
Evaluating Expressions with Two Variables
Type: Tutorial
Video/Audio/Animations
When should a system of equations with multiple variables be used to solve an Algebra problem, instead of using a single equation with a single variable?
Type: Video/Audio/Animation
The points of intersection of two graphs represent common solutions to both equations. Finding these intersection points is an important tool in analyzing physical and mathematical systems.
Type: Video/Audio/Animation
The point-slope form of the equation for a line can describe any non-vertical line in the Cartesian plane, given the slope and the coordinates of a single point which lies on the line.
Type: Video/Audio/Animation
The two point form of the equation for a line can describe any non-vertical line in the Cartesian plane, given the coordinates of two points which lie on the line.
Type: Video/Audio/Animation
Khan Academy video tutorial on graphing linear equations: "Algebra: Graphing Lines 1"
Type: Video/Audio/Animation
Parent Resources
Problem-Solving Tasks
The given solutions for this task involve the creation and solving of a system of two equations and two unknowns, with the caveat that the context of the problem implies that we are interested only in non-negative integer solutions. Indeed, in the first solution, we must also restrict our attention to the case that one of the variables is further even. This aspect of the task is illustrative of the mathematical practice of modeling with mathematics, and crucial as the system has an integer solution for both situations, that is, whether we include the dollar on the floor in the cash box or not.
Type: Problem-Solving Task
This task presents a simple but mathematically interesting game whose solution is a challenging exercise in creating and reasoning with algebraic inequalities. The core of the task involves converting a verbal statement into a mathematical inequality in a context in which the inequality is not obviously presented, and then repeatedly using the inequality to deduce information about the structure of the game.
Type: Problem-Solving Task
This task examines the ways in which the plane can be covered by regular polygons in a very strict arrangement called a regular tessellation. These tessellations are studied here using algebra, which enters the picture via the formula for the measure of the interior angles of a regular polygon (which should therefore be introduced or reviewed before beginning the task). The goal of the task is to use algebra in order to understand which tessellations of the plane with regular polygons are possible.
Type: Problem-Solving Task
Video/Audio/Animation
Khan Academy video tutorial on graphing linear equations: "Algebra: Graphing Lines 1"
Type: Video/Audio/Animation








