Clusters should not be sorted from Major to Supporting and then taught in that order. To do so would strip the coherence of the mathematical ideas and miss the opportunity to enhance the major work of the grade with the supporting clusters.
-
Also assesses:
- Assessment Limits :
Expressions should contain no more than three variables.For N-RN.1.2, items should not require the student to do more than
two operations. - Calculator :
Neutral
- Clarification :
Students will use the properties of exponents to rewrite a radical
expression as an expression with a rational exponent.Students will use the properties of exponents to rewrite an
expression with a rational exponent as a radical expression.Students will apply the properties of operations of integer exponents
to expressions with rational exponents.Students will apply the properties of operations of integer exponents
to radical expressions.Students will write algebraic proofs that show that a sum or product
of two rational numbers is rational; that the sum of a rational number
and an irrational number is irrational; and that the product of a
nonzero rational number and an irrational number is irrational. - Stimulus Attributes :
Items should be set in a mathematical context. - Response Attributes :
Items may require the student to complete an algebraic proof.Items may require the student to determine equivalent expressions
or equations.Responses with square roots should require the student to rewrite
the square root so that the radicand has no square factors
MAFS.912.N-RN.1.1
MAFS.912.N-RN.2.3
- Test Item #: Sample Item 1
- Question:
Jeremy determines that
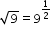 . Part of his work is shown.
. Part of his work is shown.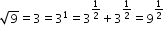
Which expression or equation should be placed in the blank to correctly complete Jeremy's work?
- Difficulty: N/A
- Type: MC: Multiple Choice
Related Courses
Related Access Points
Related Resources
Formative Assessments
Lesson Plans
Original Student Tutorials
Problem-Solving Tasks
Tutorial
Unit/Lesson Sequence
Video/Audio/Animations
MFAS Formative Assessments
Students are asked to convert numerical expressions from radical to exponential form.
Students are asked to convert numerical expressions from exponential to radical form.
Students are asked to convert a product of a radical and exponential expression to a single power of two.
Students are asked to rewrite expressions involving radicals and rational exponents in equivalent forms.
Original Student Tutorials Mathematics - Grades 9-12
Learn how to solve rational linear and quadratic equations using cross multiplication in this interactive tutorial.
Learn to rewrite products involving radicals and rational exponents using properties of exponents in this interactive tutorial.
Student Resources
Original Student Tutorials
Learn how to solve rational linear and quadratic equations using cross multiplication in this interactive tutorial.
Type: Original Student Tutorial
Learn to rewrite products involving radicals and rational exponents using properties of exponents in this interactive tutorial.
Type: Original Student Tutorial
Problem-Solving Task
In this example, students use properties of rational exponents and other algebraic concepts to compare and verify the relative size of two real numbers that involve decimal exponents.
Type: Problem-Solving Task
Tutorial
This tutorial demonstrates how to use the power of a power property with both numerals and variables.
Type: Tutorial
Video/Audio/Animations
Exponents are not only integers and unit fractions. An exponent can be any rational number expressed as the quotient of two integers.
Type: Video/Audio/Animation
Radical expressions can often be simplified by moving factors which are perfect roots out from under the radical sign.
Type: Video/Audio/Animation
Parent Resources
Problem-Solving Task
In this example, students use properties of rational exponents and other algebraic concepts to compare and verify the relative size of two real numbers that involve decimal exponents.
Type: Problem-Solving Task
Tutorial
This tutorial demonstrates how to use the power of a power property with both numerals and variables.
Type: Tutorial







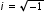 , but this is optional.
, but this is optional.
